Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\Delta'=m^2-m^2+2m-4=2m-4\)
Để phương trình có hai nghiệm thì:
\(2m-4\ge0\Rightarrow m\ge2\)
Theo vi ét: \(\left\{{}\begin{matrix}x_1+x_2=2m\\x_1x_2=m^2-2m+4\end{matrix}\right.\)
Theo đề: \(\left(x_1+1\right)\left(x_2+1\right)=9\)
\(\Leftrightarrow x_1x_2+x_1+x_2+1=9\)
\(\Leftrightarrow m^2-2m+4+2m=8\)
\(\Leftrightarrow m^2-4=0\)
\(\Rightarrow\left\{{}\begin{matrix}m=-2\left(loại\right)\\m=2\left(nhận\right)\end{matrix}\right.\)
Vậy m = 2 là giá trị cần tìm.

\(x^2-x+1-m=0\left(1\right)\\ \text{PT có 2 nghiệm }x_1,x_2\\ \Leftrightarrow\Delta=1-4\left(1-m\right)\ge0\\ \Leftrightarrow4m-3\ge0\Leftrightarrow m\ge\dfrac{3}{4}\\ \text{Vi-ét: }\left\{{}\begin{matrix}x_1+x_2=1\\x_1x_2=1-m\end{matrix}\right.\\ \text{Ta có }5\left(\dfrac{1}{x_1}+\dfrac{1}{x_2}\right)-x_1x_2+4=0\\ \Leftrightarrow5\cdot\dfrac{x_1+x_2}{x_1x_2}-x_1x_2+4=0\\ \Leftrightarrow\dfrac{5}{1-m}+m-1+4=0\\ \Leftrightarrow\dfrac{5}{1-m}+m+3=0\\ \Leftrightarrow5+\left(1-m\right)\left(m+3\right)=0\\ \Leftrightarrow m^2+2m-8=0\\ \Leftrightarrow m^2-2m+4m-8=0\\ \Leftrightarrow\left(m-2\right)\left(m+4\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}m=2\left(n\right)\\m=-4\left(l\right)\end{matrix}\right.\)
Vậy $m=2$

\(\dfrac{1}{2+\sqrt{3}}+\dfrac{1}{2-\sqrt{3}}\\ =\dfrac{2-\sqrt{3}}{\left(2+\sqrt{3}\right)\left(2-\sqrt{3}\right)}+\dfrac{2+\sqrt{3}}{\left(2+\sqrt{3}\right)\left(2-\sqrt{3}\right)}\\ =\dfrac{2-\sqrt{3}+2+\sqrt{3}}{2^2-\left(\sqrt{3}\right)^2}\\ =\dfrac{2+2}{4-3}\\ =4\)
Ta có: \(\dfrac{1}{2+\sqrt{3}}+\dfrac{1}{2-\sqrt{3}}\)
\(=2-\sqrt{3}+2+\sqrt{3}\)
=4


Ptr có `2` nghiệm phân biệt `<=>\Delta' > 0`
`=>(m+1)^2-m^2+2m-3 > 0`
`<=>m^2+2m+1-m^2+2m-3 > 0`
`<=>m > 1/2`
`=>` Áp dụng Viét có: `{(x_1+x_2=-b/a=2m+2),(x_1.x_2=c/a=m^2-2m+3):}`
Ta có: `1/[x_1 ^2]-[4x_2]/[x_1]+3x_2 ^2=0`
`=>1-4x_1.x_2+3(x_1.x_2)^2=0`
`<=>1-4(m^2-2m+3)+3(m^2-2m+3)^2=0`
`<=>[(m^2-2m+3=1),(m^2-2m+3=1/3):}`
`<=>[(m^2-2m+2=0(VN)),(m^2-2m+8/3=0(VN)):}`
`=>` Không có `m` thỏa mãn.


Lời giải:
Để pt có 2 nghiê pb thì:
$\Delta'=1-(m-3)>0\Leftrightarrow m< 4$
Áp dụng định lý Viet: \(\left\{\begin{matrix} x_1+x_2=2\\ x_1x_2=m-3\end{matrix}\right.\)
Khi đó:
\(x_1^2-2x_2+x_1x_2=-12\)
\(\Leftrightarrow x_1^2-2(2-x_1)+x_1(2-x_1)=-12\)
\(\Leftrightarrow x_1=-2\Leftrightarrow x_2=2-x_1=4\)
$m-3=x_1x_2=(-2).4=-8$
$\Leftrightarrow m=-5$ (tm)


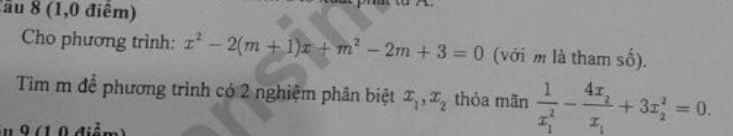




\(\left|x_1\right|+\left|x_2\right|=5\)
\(\Leftrightarrow\left(\left|x_1\right|+\left|x_2\right|\right)^2=25\)
\(\Leftrightarrow x_1^2+x_2^2+2\left|x_1x_2\right|=25\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2+2\left|x_1x_2\right|=25\)
Đến đây thay Viet vào và xét 2 trường hợp: \(\left[{}\begin{matrix}x_1x_2\ge0\\x_1x_2< 0\end{matrix}\right.\) để phá trị tuyệt đối
em cảm ơn nhiều ạ