
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1.
a. Em tự giải
b.
\(\left\{{}\begin{matrix}2x+y=4m-1\\3x-2y=-m+9\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}4x+2y=8m-2\\3x-2y=-m+9\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}7x=7m+7\\y=\dfrac{3x+m-9}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=m+1\\y=2m-3\end{matrix}\right.\)
Để \(x+y=7\Rightarrow m+1+2m-3=7\)
\(\Rightarrow3m=9\Rightarrow m=3\)
2.
a. Em tự giải
b.
Phương trình có 2 nghiệm khi:
\(\Delta'=\left(m+1\right)^2-\left(2m+10\right)=m^2-9\ge0\)
\(\Rightarrow\left[{}\begin{matrix}m\ge3\\m\le-3\end{matrix}\right.\)
Khi đó theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)\\x_1x_2=2m+10\end{matrix}\right.\)
Ta có:
\(P=x_1^2+x_2^2+8x_1x_2=\left(x_1+x_2\right)^2+6x_1x_2\)
\(=4\left(m+1\right)^2+6\left(2m+10\right)=4m^2+20m+64\)
\(=4\left(m^2+5m+6\right)+40=4\left(m+2\right)\left(m+3\right)+40\)
Do \(\left[{}\begin{matrix}m\ge3\\m\le-3\end{matrix}\right.\) \(\Rightarrow\left(m+2\right)\left(m+3\right)\ge0\)
\(\Rightarrow P\ge40\)
Vậy \(P_{min}=40\) khi \(m=-3\)
(Nếu bài này giải là \(4m^2+20m+64=\left(2m+5\right)^2+39\ge39\) là sai vì dấu = khi đó xảy ra tại \(m=-\dfrac{5}{2}\) ko thỏa mãn điều kiện \(\Delta\) để pt có nghiệm)

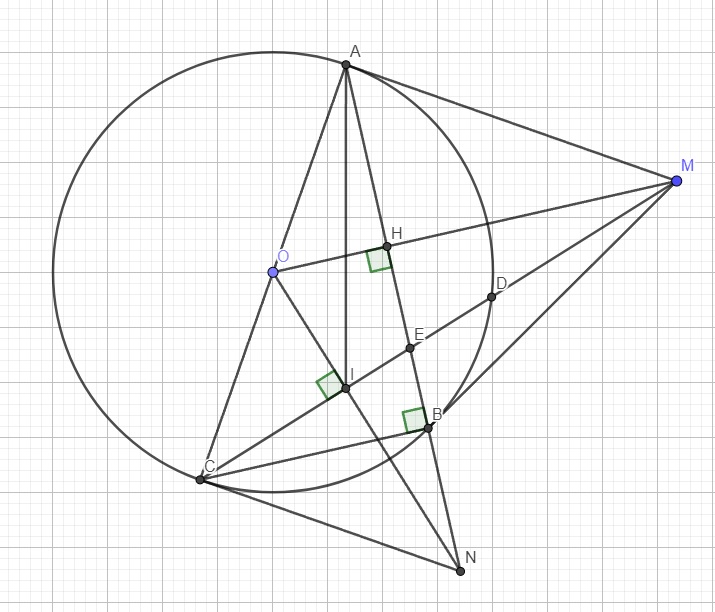
a. Câu này đơn giản em tự giải
b.
Xét hai tam giác OIM và OHN có:
\(\left\{{}\begin{matrix}\widehat{OIM}=\widehat{OHN}=90^0\\\widehat{MON}\text{ chung}\\\end{matrix}\right.\) \(\Rightarrow\Delta OIM\sim\Delta OHN\left(g.g\right)\)
\(\Rightarrow\dfrac{OI}{OH}=\dfrac{OM}{ON}\Rightarrow OI.ON=OH.OM\)
Cũng từ 2 tam giác đồng dạng ta suy ra \(\widehat{OMI}=\widehat{ONH}\)
Tứ giác OAMI nội tiếp (I và A cùng nhìn OM dưới 1 góc vuông)
\(\Rightarrow\widehat{OAI}=\widehat{OMI}\)
\(\Rightarrow\widehat{OAI}=\widehat{ONH}\) hay \(\widehat{OAI}=\widehat{ONA}\)
c.
Xét hai tam giác OAI và ONA có:
\(\left\{{}\begin{matrix}\widehat{OAI}=\widehat{ONA}\left(cmt\right)\\\widehat{AON}\text{ chung}\end{matrix}\right.\) \(\Rightarrow\Delta OAI\sim\Delta ONA\left(g.g\right)\)
\(\Rightarrow\dfrac{OA}{ON}=\dfrac{OI}{OA}\Rightarrow OI.ON=OA^2=OC^2\) (do \(OA=OC=R\))
\(\Rightarrow\dfrac{OC}{ON}=\dfrac{OI}{OC}\)
Xét hai tam giác OCN và OIC có:
\(\left\{{}\begin{matrix}\dfrac{OC}{ON}=\dfrac{OI}{OC}\\\widehat{CON}\text{ chung}\end{matrix}\right.\) \(\Rightarrow\Delta OCN\sim\Delta OIC\left(g.g\right)\)
\(\Rightarrow\widehat{OCN}=\widehat{OIC}=90^0\) hay tam giác ACN vuông tại C
\(\widehat{ABC}\) là góc nt chắn nửa đường tròn \(\Rightarrow BC\perp AB\)
Áp dụng hệ thức lượng trong tam giác vuông ACN với đường cao BC:
\(BC^2=BN.BA=BN.2BH=2BN.BH\) (1)
O là trung điểm AC, H là trung điểm AB \(\Rightarrow OH\) là đường trung bình tam giác ABC
\(\Rightarrow OH=\dfrac{1}{2}BC\)
Xét hai tam giác OHN và EBC có:
\(\left\{{}\begin{matrix}\widehat{OHN}=\widehat{EBC}=90^0\\\widehat{ONH}=\widehat{ECB}\left(\text{cùng phụ }\widehat{IEB}\right)\end{matrix}\right.\) \(\Rightarrow\Delta OHN\sim\Delta EBC\left(g.g\right)\)
\(\Rightarrow\dfrac{OH}{EB}=\dfrac{HN}{BC}\Rightarrow HN.EB=OH.BC=\dfrac{1}{2}BC^2\)
\(\Rightarrow BC^2=2HN.EB\) (2)
(1);(2) \(\Rightarrow BN.BH=HN.BE\)
\(\Rightarrow BN.BH=\left(BN+BH\right).BE\)
\(\Rightarrow\dfrac{1}{BE}=\dfrac{BN+BH}{BN.BH}=\dfrac{1}{BH}+\dfrac{1}{BN}\) (đpcm)

Mình không thấy câu nào cả thì giúp kiểu gì lỗi ảnh hay sao ý

Chắc câu c quá, tại tổng 2 ô vuông của hình chữ nhật có 10 chấm tròn. =)
Em nghĩ là câu c vì thấy tổng của các chấm tròn ở mỗi miếng đều là 10.

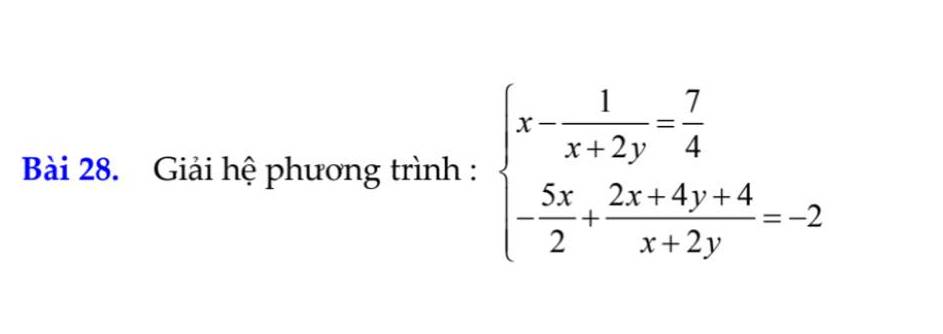
ĐKXĐ: \(x+2y\ne0\)
\(\left\{{}\begin{matrix}x-\dfrac{1}{x+2y}=\dfrac{7}{4}\\-\dfrac{5}{2}x+2+\dfrac{4}{x+2y}=-2\end{matrix}\right.\)
Đặt \(\dfrac{1}{x+2y}=z\) ta được hệ:
\(\left\{{}\begin{matrix}x-z=\dfrac{7}{4}\\-\dfrac{5}{2}x+4z=-4\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x=2\\z=\dfrac{1}{4}\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x=2\\\dfrac{1}{x+2y}=\dfrac{1}{4}\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}x=2\\x+2y=4\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x=2\\y=1\end{matrix}\right.\)

Bài 2 :
a ) \(\sqrt{4x-8}+\sqrt{x-2}=4+\dfrac{1}{3}\sqrt{9x-18}\) ( ĐKXĐ : \(x\ge2\) )
\(\Leftrightarrow2\sqrt{x-2}+\sqrt{x-2}=4+\dfrac{1}{3}.3\sqrt{x-2}\)
\(\Leftrightarrow3\sqrt{x-2}-\sqrt{x-2}=4\)
\(\Leftrightarrow2\sqrt{x-2}=4\)
\(\Leftrightarrow\sqrt{x-2}=2\)
\(\Leftrightarrow x-2=4\)
\(\Leftrightarrow x=2\) ( thỏa mãn ĐKXĐ )
Vậy phương trình có nghiệm x = 2 .
Bài 2 :
b ) \(\sqrt{x^2-6x+9}-\dfrac{\sqrt{6}+\sqrt{3}}{\sqrt{2}+1}=0\)
\(\Leftrightarrow\sqrt{\left(x-3\right)^2}-\dfrac{\sqrt{3}\left(\sqrt{2}+1\right)}{\sqrt{2}+1}=0\)
\(\Leftrightarrow|x-3|-\sqrt{3}=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-3-\sqrt{3}=0\left(x\ge3\right)\\3-x-\sqrt{3}=0\left(x< 3\right)\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=3+\sqrt{3}\\x=3-\sqrt{3}\end{matrix}\right.\)
Vậy phương trình cón nghiệm \(x=3+\sqrt{3}\) hoặc \(x=3-\sqrt{3}\) .

Gọi số xe dự định tham gia chở hàng là x (xe) với x>4, x nguyên dương
Mỗi xe dự định chở khối lượng hàng là: \(\dfrac{20}{x}\) (tấn)
Số xe thực tế tham gia chở hàng là: \(x-4\) (xe)
Thực tế mỗi xe phải chở số hàng là: \(\dfrac{20}{x-4}\) (tấn)
Do thực tế mỗi xe phải chở nhiều hơn dự định là 5/6 tấn hàng nên ta có pt:
\(\dfrac{20}{x-4}-\dfrac{20}{x}=\dfrac{5}{6}\)
\(\Rightarrow24x-24\left(x-4\right)=x\left(x-4\right)\)
\(\Leftrightarrow x^2-4x-96=0\)
\(\Rightarrow\left[{}\begin{matrix}x=12\\x=-8\left(loại\right)\end{matrix}\right.\)
Vậy thực tế có \(12-4=8\) xe tham gia vận chuyển

b) \(\sqrt{x^2}=\left|-8\right|\)
\(\Rightarrow\left|x\right|=8\)
\(\Rightarrow\left[{}\begin{matrix}x=8\\x=-8\end{matrix}\right.\)
d) \(\sqrt{9x^2}=\left|-12\right|\)
\(\Rightarrow\sqrt{\left(3x\right)^2}=12\)
\(\Rightarrow\left|3x\right|=12\)
\(\Rightarrow\left[{}\begin{matrix}3x=12\\3x=-12\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=\dfrac{12}{3}\\x=-\dfrac{12}{3}\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}x=4\\x=-4\end{matrix}\right.\)

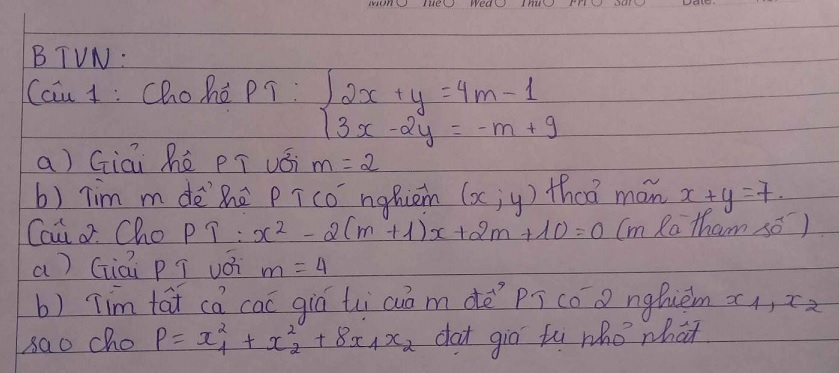

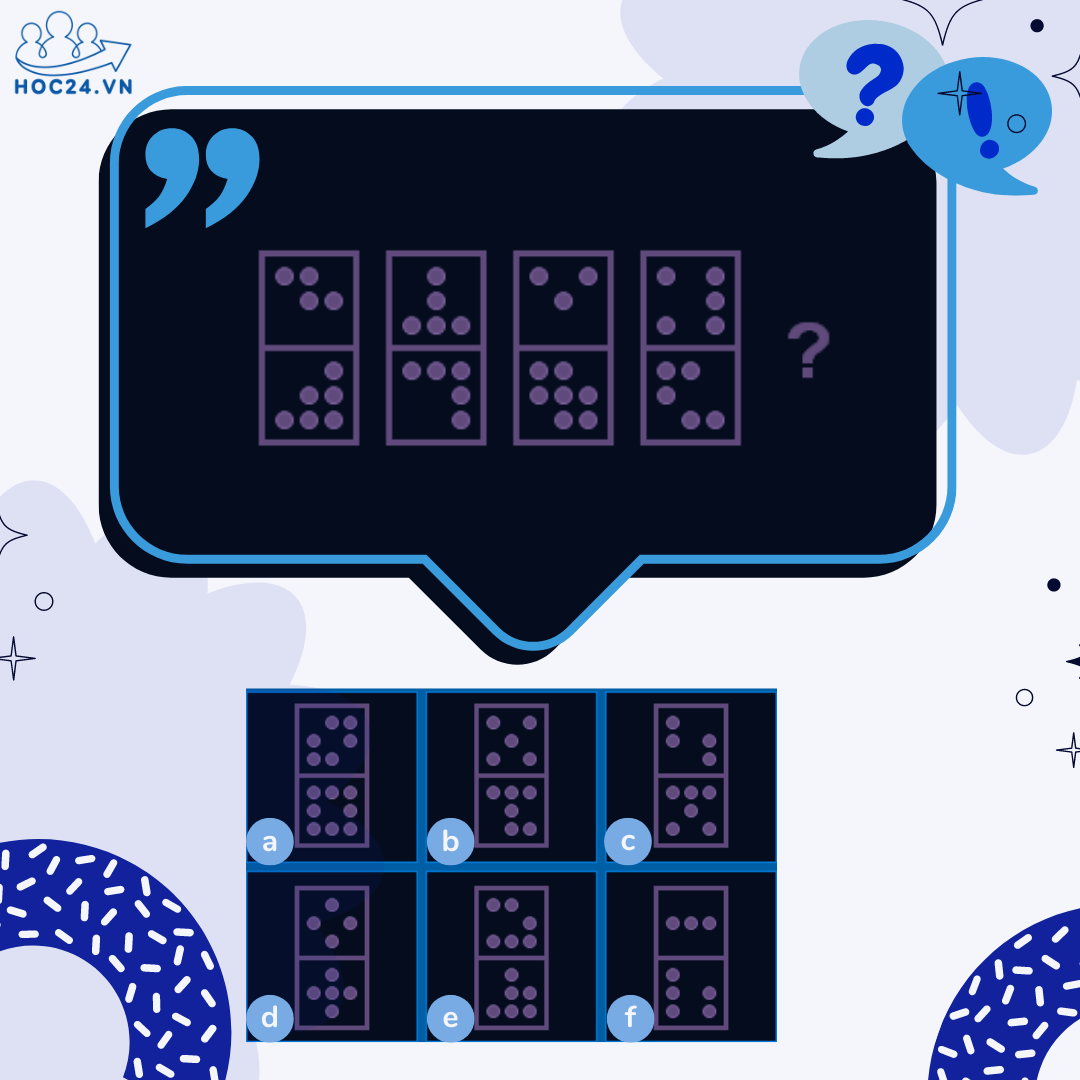

 giúp vs ạ
giúp vs ạ


Câu 5:
\(\left\{{}\begin{matrix}x^2+y^2=4\left('\right)\\x-y-xy=2\left(''\right)\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x-y\right)^2+2xy=4\\x-y-xy=2\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}\left(x-y\right)^2+2xy=4\left(1\right)\\2\left(x-y\right)-2xy=4\left(2\right)\end{matrix}\right.\)
Lấy \(\left(1\right)+\left(2\right)\) ta được:
\(\left(x-y\right)^2+2\left(x-y\right)=8\)
\(\Leftrightarrow\left(x-y\right)^2+2\left(x-y\right)+1-9=0\)
\(\Leftrightarrow\left(x-y+1\right)^2-9=0\)
\(\Leftrightarrow\left(x-y-2\right)\left(x-y+4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-y=2\\x-y=-4\end{matrix}\right.\)
Với \(x-y=2\) Thay vào \(\left(''\right)\) ta được:
\(2-xy=2\Rightarrow xy=0\)
\(\Rightarrow\left[{}\begin{matrix}x=0\Rightarrow y=-2\\y=0\Rightarrow x=2\end{matrix}\right.\)
Với \(x-y=4\Rightarrow x=4+y\) Thay vào \(\left('\right)\) ta được:
\(\left(4+y\right)^2+y^2=4\)
\(\Leftrightarrow y^2+8y+16+y^2-4=0\)
\(\Leftrightarrow2y^2+8y+12=0\)
\(\Leftrightarrow y^2+4y+6=0\)
\(\Leftrightarrow\left(y+2\right)^2+2=0\) (phương trình vô nghiệm).
Vậy hệ phương trình đã cho có nghiệm \(\left(x,y\right)\in\left\{\left(2;0\right),\left(0;-2\right)\right\}\)
Câu 6: \(\left\{{}\begin{matrix}2xy+y^2=3\left('\right)\\x^2+5xy=6\left(''\right)\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}4xy+2y^2=6\left(1\right)\\x^2+5xy=6\left(2\right)\end{matrix}\right.\)
Lấy \(\left(2\right)-\left(1\right)\) ta được:
\(x^2+xy-2y^2=0\)
\(\Leftrightarrow x^2-y^2+xy-y^2=0\)
\(\Leftrightarrow\left(x-y\right)\left(x+y\right)+y\left(x-y\right)=0\)
\(\Leftrightarrow\left(x-y\right)\left(x+2y\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=y\\x=-2y\end{matrix}\right.\)
Với \(x=y\) Thay vào \(\left('\right)\) ta được:
\(2y.y+y^2=3\)
\(\Leftrightarrow y=\pm1\Rightarrow x=\pm1\).
Với \(x=-2y\) Thay vào \(\left('\right)\) ta được:
\(2.\left(-2y\right).y+y^2=3\)
\(\Leftrightarrow y^2=-1\) (phương trình vô nghiệm)
Vậy hệ phương trình đã cho có nghiệm \(\left(x,y\right)\in\left\{\left(1;1\right),\left(-1;-1\right)\right\}\)