
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


Hướng dẫn:
Ta có:
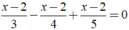
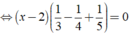
⇔ ( x - 2 )17/60 = 0 ⇔ x - 2 = 0 ⇔ x = 2.
Vậy phương trình có tập nghiệm là S = { 2 }.


(x+1).(x+2).(x+3).(x+4) - 24 = 0
(x2 + 5x + 4).(x2 + 5x + 6) - 24 = 0
(x2 + 5x + 5-1).(x2 + 5x + 5 + 1) - 24 = 0
(x2 + 5x + 5)2 - 1 - 24 = 0
(x2 + 5x + 5 - 5).(x2 + 5x + 5 + 5) = 0
x.(x+5) .(x2 + 5x + 10) = 0
=> x = 0
x+ 5 = 0 => x = -5
\(x^2+5x+10>0\)
KL:..
(x+1)(x+2)(x+3)(x+4) - 24 = 0
<=> [(x+1)(x+4)][(x+2)(x+3)] - 24 =0
<=> (x^2+4x+x+4)(x^2+3x+2x+6) - 24 = 0
<=> (x^2+5x+4)(x^2+5x+6) - 24 = 0
Đặt x^2+5x+5 = a, ta có
(a-1)(a+1) - 24 = 0
<=> a^2 - 1 - 24 = 0
<=> a^2 - 25 =0
<=> a = 5
hay x^2 + 5x + 5 = 5
<=> x(x+5) = 5 - 5 = 0
<=> x=0 hoặc x+5 = 0 <=> x= -5
Vậy tập ngh của p.tr là S = { 0; -5 }

(x + 2)(x + 3)(x + 4)(x + 5) - 24 = 02
\(\Leftrightarrow\) [(x + 2)(x + 5)][(x + 3)(x + 4)] - 24 = 0
\(\Leftrightarrow\) (x2 + 7x + 10)(x2 + 7x + 12) - 24 = 0
Đặt x2 + 7x + 11 = a. Thay vào ta có :
(a - 1)(a + 1) - 24 = 0
\(\Leftrightarrow\) a2 - 25 = 0
\(\Leftrightarrow\) (a - 5)(a + 5) = 0
\(\Leftrightarrow\left[{}\begin{matrix}a-5=0\\a+5=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}a=5\\a=-5\end{matrix}\right.\)
TH1 : a = 5
\(\Leftrightarrow\) x2 + 7x + 11 = 5
\(\Leftrightarrow\) x2 + 7x + 6 = 0
\(\Leftrightarrow\) (x + 1)(x + 6) = 0
\(\Leftrightarrow\left[{}\begin{matrix}x+1=0\\x+6=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=-1\\x=-6\end{matrix}\right.\)
TH2 : x2 + 7x + 11 = -5
\(\Leftrightarrow\) x2 + 7x + 16 = 0
\(\Leftrightarrow\) (x2 + 2.\(\frac{7}{2}\).x + \(\frac{49}{4}\)) + \(\frac{15}{4}\) = 0
\(\Leftrightarrow\) (x + \(\frac{7}{2}\))2 + \(\frac{15}{4}\) = 0 mà \(\left(x+\frac{7}{2}\right)^2\ge0\) \(\Rightarrow\) Vô lý
Vậy S = \(\left\{-1,-6\right\}\)

\(\Leftrightarrow\left(x^2+5x+4\right)\left(x^2+5x+6\right)-24=0\)
\(Đặt\) \(t=x^2+5x+5\). PT thành
\(\left(t-1\right)\left(t+1\right)-24=0\Leftrightarrow t^2-25=0\Leftrightarrow\left(t-5\right)\left(t+5\right)=0\Rightarrow\left[{}\begin{matrix}x^2+5x+5=5\\x^2+5x+5=-5\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x\left(x+5\right)=0\\\left(x+\frac{5}{2}\right)^2+\frac{15}{4}>0\end{matrix}\right.\)
Vậy x=0 hoặc x=-5

(x2 + 7x)2 - 2(x2 + 7x) - 24 = 0
<=> (x2 + 7x)(x2 + 7x - 2) - 24 = 0 (1)
Đặt t = x2 + 7x - 1 = \(=\left(x+\frac{7}{2}\right)^2-\frac{53}{4}\)
(1) trở thành (t + 1)(t - 1) - 24 = 0
<=> t2 - 1 - 24 = 0
<=> t2 - 25 = 0
<=> t2 = 25
<=> t = 5 hoặc t = -5
+) t =\(\left(x+\frac{7}{2}\right)^2-\frac{53}{4}\) = 5
\(\Leftrightarrow\left(x+\frac{7}{2}\right)^2=\frac{73}{4}\)
\(\Leftrightarrow x=\frac{-7+\sqrt{73}}{2};x=\frac{-7-\sqrt{73}}{2}\)
+) t = \(\left(x+\frac{7}{2}\right)^2-\frac{53}{4}=-5\)
\(\Leftrightarrow\left(x+\frac{7}{2}\right)^2=\frac{33}{4}\)
\(\Leftrightarrow x=\frac{-7+\sqrt{33}}{2};x=\frac{-7-\sqrt{33}}{2}\)
Vậy ...

1. Ta có \(\left(x+2\right)\left(x+4\right)\left(x+6\right)\left(x+8\right)+16=0\)
\(\Rightarrow\)\(\left[\left(x+2\right)\left(x+8\right)\right].\left[\left(x+4\right)\left(x+6\right)\right]+16=0\)
\(\Rightarrow\left(x^2+10x+16\right)\left(x^2+10x+24\right)+16=0\)
Đặt \(x^2+10x=t\)
Pt \(\Leftrightarrow\left(t+16\right)\left(t+24\right)+16=0\Leftrightarrow t^2+40t+400=0\Leftrightarrow t=-20\)
\(\Rightarrow x^2+10x+20=0\Rightarrow\orbr{\begin{cases}x=-5+\sqrt{5}\\x=-5-\sqrt{5}\end{cases}}\)
2. Ta có \(\left(x+2\right)\left(x+3\right)\left(x+4\right)\left(x+5\right)-24=0\)
\(\Rightarrow\left[\left(x+2\right)\left(x+5\right)\right].\left[\left(x+3\right)\left(x+4\right)\right]-24=0\)\(\Rightarrow\left(x^2+7x+10\right)\left(x^2+7x+12\right)-24=0\)
Đặt \(x^2+7x=t\Rightarrow\left(t+10\right)\left(t+12\right)-24=0\Rightarrow t^2+22t+96=0\)\(\Rightarrow\orbr{\begin{cases}t=-6\\t=-16\end{cases}}\)
Với \(t=-6\Rightarrow x^2+7x+6=0\Rightarrow\orbr{\begin{cases}x=-6\\x=-1\end{cases}}\)
Với \(t=-16\Rightarrow x^2+7x+16=0\left(l\right)\)
Vậy pt có 2 nghiệm là \(\orbr{\begin{cases}x=-6\\x=-1\end{cases}}\)
Quản lí Hoàng Thị Lan Hương giúp em giải bài toán vừa đăng lên đc ko ạ.??? ^^

Đặt x2 + 10x + 24 = y
pt đã cho trở thành ( y + 4x ).y - 165x2 = 0
<=> y2 + 4xy - 165x2 = 0
<=> y2 - 11xy + 15xy - 165x2 = 0
<=> y( y - 11x ) + 15x( y - 11x ) = 0
<=> ( y - 11x )( y + 15x ) = 0
=> ( x2 + 10x + 24 - 11x )( x2 + 10x + 24 + 15x ) = 0
<=> ( x2 - x + 24 )( x2 + 25x + 24 ) = 0
<=> ( x2 - x + 24 )( x2 + 24x + x + 24 ) = 0
<=> ( x2 - x + 24 )[ x( x + 24 ) + ( x + 24 ) ] = 0
<=> ( x2 - x + 24 )( x + 24 )( x + 1 ) = 0
Vì x2 - x + 24 > 0 ∀ x
nên pt <=> ( x + 24 )( x + 1 ) = 0 <=> x = -24 hoặc x = -1
Vậy ...
Đặt t = \(x^2+14x+24\)
\(\Rightarrow\)\(t\left(t-4x\right)-165x^{^2}=0\)
\(\Leftrightarrow t^2-4xt-165x^2=0\)
\(\Leftrightarrow t^2+11xt-15xt-165x^2=0\)
\(\Leftrightarrow t\left(t+11x\right)-15x\left(t+11x\right)=0\)
\(\Leftrightarrow\left(t+11x\right)\left(t-15x\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}t+11x=0\\t-15x=0\end{cases}\Leftrightarrow\orbr{\begin{cases}t=-11x\\t=15x\end{cases}}}\)
với t= -11x
\(\Rightarrow x^2+14x+24=-11x\)
\(\Leftrightarrow x^2+25x+24=0\)
\(\Leftrightarrow x^2+x+24x+24=0\)
\(\Leftrightarrow x\left(x+1\right)+24\left(x+1\right)=0\)
\(\Leftrightarrow\left(x+1\right)\left(x+24\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x+1=0\\x+24=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=-1\\x=-24\end{cases}}}\)
với t=15x
\(\Rightarrow x^2+14x+24=15x\)
\(\Leftrightarrow x^2-x+24=0\)
\(\Leftrightarrow\left(x-\frac{1}{2}\right)^2+\frac{95}{4}=0\)(Vô Lí)
vậy....

Sửa đề: \(\dfrac{74-x}{26}+\dfrac{75-x}{25}+\dfrac{76-x}{24}+\dfrac{77-x}{23}+\dfrac{78-x}{22}=-5\)Ta có: \(\dfrac{74-x}{26}+\dfrac{75-x}{25}+\dfrac{76-x}{24}+\dfrac{77-x}{23}+\dfrac{78-x}{22}=-5\)
\(\Leftrightarrow\dfrac{74-x}{26}+1+\dfrac{75-x}{25}+1+\dfrac{76-x}{24}+1+\dfrac{77-x}{23}+1+\dfrac{78-x}{22}+1=0\)
\(\Leftrightarrow\dfrac{100-x}{26}+\dfrac{100-x}{25}+\dfrac{100-x}{24}+\dfrac{100-x}{23}+\dfrac{100-x}{22}=0\)
\(\Leftrightarrow\left(100-x\right)\left(\dfrac{1}{26}+\dfrac{1}{25}+\dfrac{1}{24}+\dfrac{1}{23}+\dfrac{1}{22}\right)=0\)
mà \(\dfrac{1}{26}+\dfrac{1}{25}+\dfrac{1}{24}+\dfrac{1}{23}+\dfrac{1}{22}>0\)
nên 100-x=0
hay x=100
Vậy: S={100}
Ta có : \(\dfrac{74-x}{26}+\dfrac{75-x}{25}+\dfrac{76-x}{24}+\dfrac{77-x}{23}+\dfrac{78-x}{22}=-5\)
\(\Leftrightarrow\dfrac{74-x}{26}+\dfrac{75-x}{25}+\dfrac{76-x}{24}+\dfrac{77-x}{23}+\dfrac{78-x}{22}+5=0\)
\(\Leftrightarrow\dfrac{74-x}{26}+1+\dfrac{75-x}{25}+1+\dfrac{76-x}{24}+1+\dfrac{77-x}{23}+1+\dfrac{78-x}{22}+1=0\)
\(\Leftrightarrow\dfrac{100-x}{26}+\dfrac{100-x}{25}+\dfrac{100-x}{24}+\dfrac{100-x}{23}+\dfrac{100-x}{22}=0\)
\(\Leftrightarrow\left(100-x\right)\left(\dfrac{1}{26}+\dfrac{1}{25}+\dfrac{1}{24}+\dfrac{1}{23}+\dfrac{1}{22}\right)=0\)
Thấy : \(\dfrac{1}{26}+\dfrac{1}{25}+\dfrac{1}{24}+\dfrac{1}{23}+\dfrac{1}{22}\ne0\)
\(\Rightarrow100-x=0\)
\(\Leftrightarrow x=100\)
Vậy ...
\(x^3-x+24=0\)
\(\Leftrightarrow x^3+3x^2-3x^2-9x+8x+24=0\)
\(\Leftrightarrow x^2\left(x+3\right)-3x\left(x+3\right)+8\left(x+3\right)=0\)
\(\Leftrightarrow\left(x+3\right)\left(x^2-3x+8\right)=0\)
\(\Leftrightarrow x=-3\)
Ta có: \(x^3-x+24=0\)
\(\Leftrightarrow x^3+3x^2-3x^2-9x+8x+24=0\)
\(\Leftrightarrow\left(x+3\right)\left(x^2-3x+8\right)=0\)
\(\Leftrightarrow x+3=0\)
hay x=-3