

Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.





a) \(\cos \left( {3x - \frac{\pi }{4}} \right) = - \frac{{\sqrt 2 }}{2}\;\;\;\; \Leftrightarrow \cos \left( {3x - \frac{\pi }{4}} \right) = \cos \frac{{3\pi }}{4}\;\;\; \Leftrightarrow \left[ {\begin{array}{*{20}{c}}{3x - \frac{\pi }{4} = \frac{{3\pi }}{4} + k2\pi }\\{3x - \frac{\pi }{4} = - \frac{{3\pi }}{4} + k2\pi }\end{array}} \right.\;\;\;\; \Leftrightarrow \left[ {\begin{array}{*{20}{c}}{3x = \pi + k2\pi }\\{3x = - \frac{\pi }{2} + k2\pi }\end{array}} \right.\)
\( \Leftrightarrow \;\left[ {\begin{array}{*{20}{c}}{x = \frac{\pi }{3} + \frac{{k2\pi }}{3}}\\{x = - \frac{\pi }{6} + \frac{{k2\pi }}{3}}\end{array}} \right.\;\;\left( {k \in \mathbb{Z}} \right)\)
b) \(2{\sin ^2}x - 1 + \cos 3x = 0\;\;\;\;\; \Leftrightarrow \cos 2x + \cos 3x = 0\;\; \Leftrightarrow 2\cos \frac{{5x}}{2}\cos \frac{x}{2} = 0\;\; \Leftrightarrow \left[ {\begin{array}{*{20}{c}}{\cos \frac{{5x}}{2} = 0}\\{\cos \frac{x}{2} = 0}\end{array}} \right.\)
\( \Leftrightarrow \left[ {\begin{array}{*{20}{c}}{\frac{{5x}}{2} = \frac{\pi }{2} + k\pi }\\{\frac{{5x}}{2} = - \frac{\pi }{2} + k\pi }\\{\frac{x}{2} = \frac{\pi }{2} + k\pi }\\{\frac{x}{2} = - \frac{\pi }{2} + k\pi }\end{array}} \right.\;\;\;\;\;\;\; \Leftrightarrow \left[ {\begin{array}{*{20}{c}}{x = \frac{\pi }{5} + \frac{{k2\pi }}{5}}\\{x = - \frac{\pi }{5} + \frac{{k2\pi }}{5}}\\{x = \pi + k2\pi }\\{x = - \pi + k2\pi }\end{array}} \right.\;\;\;\left( {k \in \mathbb{Z}} \right)\)
c) \(\tan \left( {2x + \frac{\pi }{5}} \right) = \tan \left( {x - \frac{\pi }{6}} \right)\;\; \Leftrightarrow 2x + \frac{\pi }{5} = x - \frac{\pi }{6} + k\pi \;\;\; \Leftrightarrow x = - \frac{{11\pi }}{{30}} + k\pi \;\;\left( {k \in \mathbb{Z}} \right)\)

a) Đặt t = cos![]() , t ∈ [-1 ; 1] thì phương trình trở thành
, t ∈ [-1 ; 1] thì phương trình trở thành
(1 - t2) - 2t + 2 = 0 ⇔ t2 + 2t -3 = 0 ⇔ ![]()
Phương trình đã cho tương đương với
cos![]() = 1 ⇔
= 1 ⇔ ![]() = k2π ⇔ x = 4kπ, k ∈ Z.
= k2π ⇔ x = 4kπ, k ∈ Z.
b) Đặt t = sinx, t ∈ [-1 ; 1] thì phương trình trở thành
8(1 - t2) + 2t - 7 = 0 ⇔ 8t2 - 2t - 1 = 0 ⇔ t ∈ {![]() }.
}.
Các nghiệm của phương trình đã cho là nghiệm của hai phương trình sau :
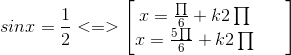
và ![]()
Đáp số : x = ![]() + k2π; x =
+ k2π; x = ![]() + k2π;
+ k2π;
x = arcsin(![]() ) + k2π; x = π - arcsin(
) + k2π; x = π - arcsin(![]() ) + k2π, k ∈ Z.
) + k2π, k ∈ Z.
c) Đặt t = tanx thì phương trình trở thành 2t2 + 3t + 1 = 0 ⇔ t ∈ {-1 ; ![]() }.
}.
Vậy ![]()
d) Đặt t = tanx thì phương trình trở thành
t - ![]() + 1 = 0 ⇔ t2 + t - 2 = 0 ⇔ t ∈ {1 ; -2}.
+ 1 = 0 ⇔ t2 + t - 2 = 0 ⇔ t ∈ {1 ; -2}.
Vậy ![]()



a) \(\sin x = \frac{{\sqrt 3 }}{2}\;\; \Leftrightarrow \sin x = \sin \frac{\pi }{3}\;\;\; \Leftrightarrow \left[ {\begin{array}{*{20}{c}}{x = \frac{\pi }{3} + k2\pi }\\{x = \pi - \frac{\pi }{3} + k2\pi }\end{array}} \right.\;\;\; \Leftrightarrow \left[ {\begin{array}{*{20}{c}}{x = \frac{\pi }{3} + k2\pi }\\{x = \frac{{2\pi }}{3} + k2\pi \;}\end{array}\;} \right.\left( {k \in \mathbb{Z}} \right)\)
b) \(2\cos x = - \sqrt 2 \;\; \Leftrightarrow \cos x = - \frac{{\sqrt 2 }}{2}\;\;\; \Leftrightarrow \cos x = \cos \frac{{3\pi }}{4}\;\;\; \Leftrightarrow \left[ {\begin{array}{*{20}{c}}{x = \frac{{3\pi }}{4} + k2\pi }\\{x = - \frac{{3\pi }}{4} + k2\pi }\end{array}\;\;\left( {k \in \mathbb{Z}} \right)} \right.\)
c) \(\sqrt 3 \;\left( {\tan \frac{x}{2} + {{15}^0}} \right) = 1\;\;\; \Leftrightarrow \tan \left( {\frac{x}{2} + \frac{\pi }{{12}}} \right) = \frac{1}{{\sqrt 3 }}\;\; \Leftrightarrow \tan \left( {\frac{x}{2} + \frac{\pi }{{12}}} \right) = \tan \frac{\pi }{6}\)
\( \Leftrightarrow \frac{x}{2} + \frac{\pi }{{12}} = \frac{\pi }{6} + k\pi \;\;\;\; \Leftrightarrow \frac{x}{2} = \frac{\pi }{{12}} + k\pi \;\;\; \Leftrightarrow x = \frac{\pi }{6} + k\pi \;\left( {k \in \mathbb{Z}} \right)\)
d) \(\cot \left( {2x - 1} \right) = \cot \frac{\pi }{5}\;\;\;\; \Leftrightarrow 2x - 1 = \frac{\pi }{5} + k\pi \;\;\;\; \Leftrightarrow 2x = \frac{\pi }{5} + 1 + k\pi \;\; \Leftrightarrow x = \frac{\pi }{{10}} + \frac{1}{2} + \frac{{k\pi }}{2}\;\;\left( {k \in \mathbb{Z}} \right)\)