
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a: góc BEC=góc BFC=90 độ
=>BFEC nội tiếp
góc AEH+góc AFH=180 độ
=>AEHF nội tiếp
b: góc ABT=1/2*180=90 độ
=>BT vuông góc AB
=>BT//CH
góc ACT=1/2*180=90 độ
=>AC vuông góc CT
=>CT//BH
mà BT//CH
nên BHCT là hình bình hành

\(x^2+4=3x-1
\)
<=> \(x^2+4-3x+2=0\)
<=> \(x^2-3x+6=0\)
<=>\(\left(x-3\right)^2=0\)
<=> x-3=0
<=> x=3
Vậy S= {3}
ĐKXĐ: \(x\in R\)
\(\sqrt{x^2+4}=3x-2\)
=>\(\left\{{}\begin{matrix}3x-2>=0\\x^2+4=\left(3x-2\right)^2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=\dfrac{2}{3}\\9x^2-12x+4-x^2-4=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=\dfrac{2}{3}\\8x^2-12x=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>=\dfrac{2}{3}\\2x^2-3x=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x>=\dfrac{2}{3}\\x\left(2x-3\right)=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x>=\dfrac{2}{3}\\\left[{}\begin{matrix}x=0\\x=\dfrac{3}{2}\end{matrix}\right.\end{matrix}\right.\)
=>x=3/2

ĐKXĐ: \(x\ge3\)
\(pt\Leftrightarrow5\sqrt{x-3}+3\sqrt{x-3}-\sqrt{x-3}=7\)
\(\Leftrightarrow7\sqrt{x-3}=7\Leftrightarrow\sqrt{x-3}=1\)
\(\Leftrightarrow x-3=1\Leftrightarrow x=4\left(tm\right)\)


Bạn ơi, làm như vậy thì quá ngắn rồi ạ, với lại bạn làm thiếu mất đề bài của mình rồi

Câu 6: Để hàm số y=(1-m)x+3 nghịch biến trên R thì 1-m<0
=>m>1
=>Chọn B
Câu 7: D
Câu 10: (D)//(D')
=>\(\left\{{}\begin{matrix}3m+1=2\left(m+1\right)\\-2\ne-2\left(loại\right)\end{matrix}\right.\Leftrightarrow m\in\varnothing\)
=>Chọn D
Câu 11: \(x^2+2x+2=\left(x+1\right)^2+1>=1>0\forall x\)
=>\(\sqrt{x^2+2x+2}\) luôn xác định với mọi số thực x
=>Chọn A
Câu 12: Để hai đường thẳng y=x+3m+2 và y=3x+2m+3 cắt nhau tại một điểm trên trục tung thì \(\left\{{}\begin{matrix}1\ne3\left(đúng\right)\\3m+2=2m+3\end{matrix}\right.\)
=>3m+2=2m+3
=>m=1
=>Chọn C

a) \(\Leftrightarrow A=\dfrac{\left(\sqrt{x}+3\right)\left(\sqrt{x}-1\right)}{\sqrt{x}\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}-\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\)
\(\Leftrightarrow A=\dfrac{x+2\sqrt{x}-3-x+2\sqrt{x}+3}{\sqrt{x}\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}\)
\(\Leftrightarrow A=\dfrac{4\sqrt{x}}{\sqrt{x}\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}=\dfrac{4}{x-1}\)
b) \(A=7\) \(\Leftrightarrow\dfrac{4}{x-1}=7\)
\(\Leftrightarrow7x-7=4\) \(\Leftrightarrow7x=11\Leftrightarrow x=\dfrac{11}{7}\left(TM\right)\)
\(a,A=\dfrac{x+2\sqrt{x}-3-x+2\sqrt{x}+3}{\sqrt{x}\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}=\dfrac{4\sqrt{x}}{\sqrt{x}\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}=\dfrac{4}{x-1}\\ b,A=7\Leftrightarrow x-1=\dfrac{4}{7}\Leftrightarrow x=\dfrac{11}{7}\left(tm\right)\)

Lời giải:
a. ĐKXĐ: $x>0; x\neq 4$
b.
\(M=\sqrt{x}.\left[\frac{1}{\sqrt{x}-2}+\frac{1}{\sqrt{x}+2}\right].\frac{x-4}{2\sqrt{x}}\)
\(=\frac{2\sqrt{x}}{(\sqrt{x}-2)(\sqrt{x}+2)}.\frac{x-4}{2}=\frac{2\sqrt{x}}{x-4}.\frac{x-4}{2}=\sqrt{x}\)
c. Để $M>3\Leftrightarrow \sqrt{x}>3\Leftrightarrow x>9$
Kết hợp đkxđ suy ra $x>9$ thì $M>3$
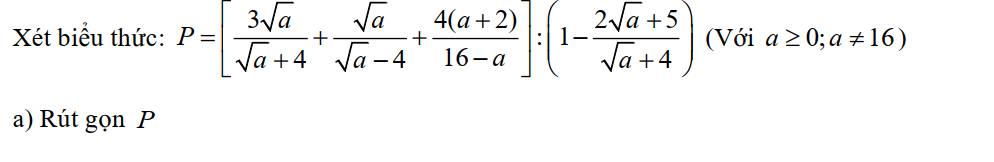


 Nhãn
Nhãn

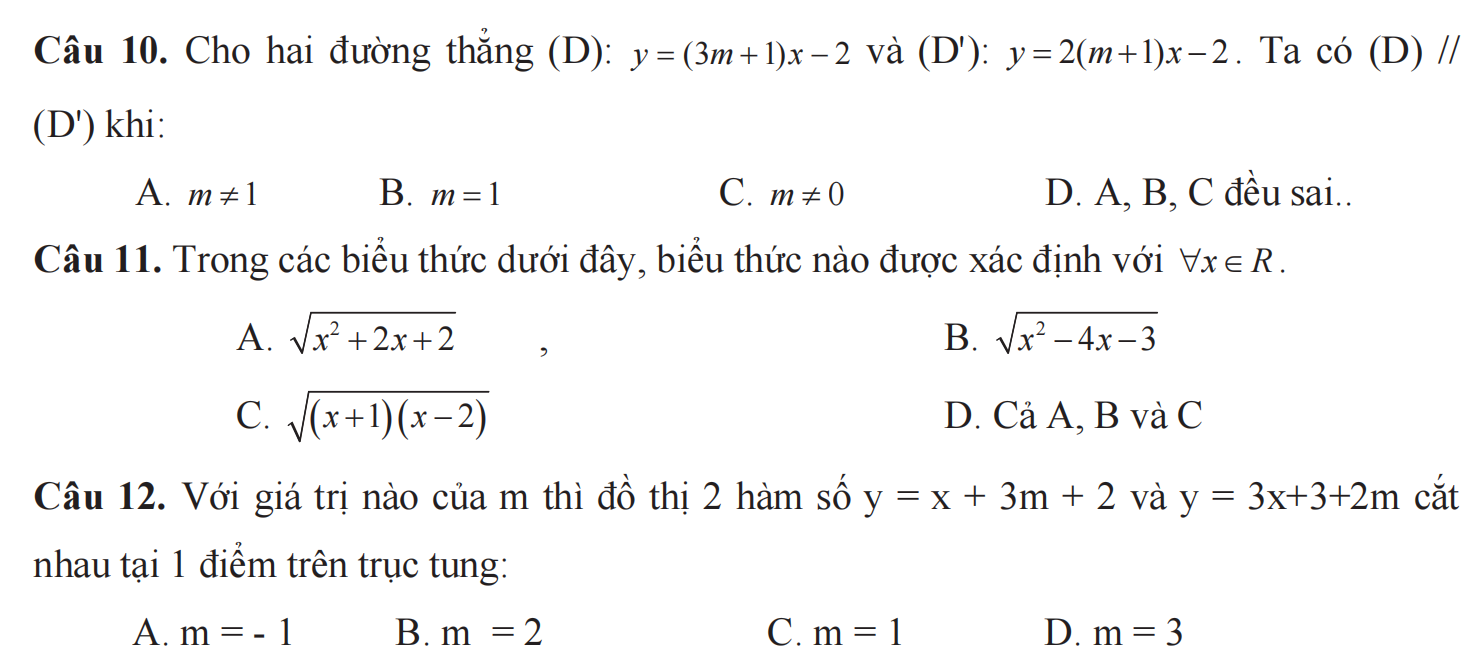

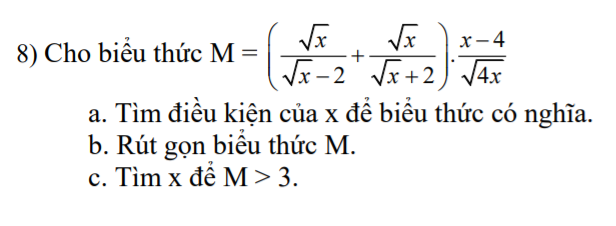
\(a,P=\left[\dfrac{3\sqrt{a}}{\sqrt{a}+4}+\dfrac{\sqrt{a}}{\sqrt{a}-4}+\dfrac{4\left(a+2\right)}{16-a}\right]:\left(1-\dfrac{2\sqrt{a}+5}{\sqrt{a}+4}\right)\left(dk:a\ge0,a\ne16\right)\\ =\left[\dfrac{3\sqrt{a}}{\sqrt{a}+4}+\dfrac{\sqrt{a}}{\sqrt{a}-4}-\dfrac{4a+8}{a-16}\right]:\left(\dfrac{\sqrt{a}+4-2\sqrt{a}-5}{\sqrt{a}+4}\right)\)
\(=\left[\dfrac{3\sqrt{a}\left(\sqrt{a}-4\right)+\sqrt{a}\left(\sqrt{a}+4\right)-\left(4a+8\right)}{\left(\sqrt{a}-4\right)\left(\sqrt{a}+4\right)}\right].\dfrac{\sqrt{a}+4}{-\sqrt{a}-1}\)
\(=\dfrac{3a-12\sqrt{a}+a+4\sqrt{a}-4a-8}{\sqrt{a}-4}.\left(-\sqrt{a}-1\right)\\ =\dfrac{-8\sqrt{a}-8}{-\left(\sqrt{a}-4\right)\left(\sqrt{a}+1\right)}\\ =\dfrac{-8\left(\sqrt{a}+1\right)}{-\left(\sqrt{a}-4\right)\left(\sqrt{a}+1\right)}\\ =\dfrac{8}{\sqrt{a}-4}\)
\(\dfrac{8}{\sqrt{a-4}}\)