
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


14, \(\frac{-7\sqrt{x}+7}{5\sqrt{x}-1}+\frac{2\sqrt{x}-2}{\sqrt{x}+2}+\frac{39\sqrt{x}+12}{5x+9\sqrt{x}-2}\)
\(=\frac{-7\sqrt{x}+7}{5\sqrt{x}-1}+\frac{2\sqrt{x}-2}{\sqrt{x}+2}+\frac{39\sqrt{x}+12}{\left(\sqrt{x}+2\right)\left(5\sqrt{x}-1\right)}\)
\(=\frac{\left(-7\sqrt{x}+7\right)\left(\sqrt{x}+2\right)+\left(2\sqrt{x}-2\right)\left(5\sqrt{x}-1\right)+39\sqrt{x}+12}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\frac{-7x-14\sqrt{x}+7\sqrt{x}+14+10x-2\sqrt{x}-10\sqrt{x}+2+39\sqrt{x}+12}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\frac{3x+20\sqrt{x}+28}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\frac{\left(3\sqrt{x}+14\right)\left(\sqrt{x}+2\right)}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
\(=\frac{3\sqrt{x}+14}{5\sqrt{x}-1}\)

16. \(\dfrac{2\sqrt{x}-4}{3\sqrt{x}-4}-\dfrac{4+2\sqrt{x}}{\sqrt{x}-2}+\dfrac{x+13\sqrt{x}-20}{3x-10\sqrt{x}+8}\)
=\(\dfrac{\left(2\sqrt{x}-4\right)\left(\sqrt{x}-2\right)-\left(4+2\sqrt{x}\right)\left(3\sqrt{x}-4\right)+x+13\sqrt{x}-20}{\left(3\sqrt{x}-4\right)\left(\sqrt{x}-2\right)}\)
=\(\dfrac{2x-8\sqrt{x}+8-\left(4\sqrt{x}+6x-16\right)+x+13\sqrt{x}-20}{\left(3\sqrt{x}-4\right)\left(\sqrt{x}-2\right)}\)
=\(\dfrac{2x-8\sqrt{x}+8-4\sqrt{x}-6x+16+x+13\sqrt{x}-20}{\left(3\sqrt{x}-4\right)\left(\sqrt{x}-2\right)}\)
=\(\dfrac{-3x+\sqrt{x}+4}{\left(3\sqrt{x}-4\right)\left(x+2\right)}\)
=\(\dfrac{-\left(3x+3\sqrt{x}-4\sqrt{x}-4\right)}{\left(3\sqrt{x}-4\right)\left(x+2\right)}\)
=\(\dfrac{-\left(3\sqrt{x}-4\right)\left(\sqrt{x}+1\right)}{\left(3\sqrt{x}-4\right)\left(\sqrt{x}+2\right)}\)=\(\dfrac{-\sqrt{x}-1}{\sqrt{x}+2}\)
14.
=\(\dfrac{-\left(7\sqrt{x}+7\right)\left(\sqrt{x}+2\right)}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)+\(\dfrac{\left(2\sqrt{x}-2\right)\left(5\sqrt{x}-1\right)}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)+\(\dfrac{39\sqrt{x}+12}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
=\(\dfrac{-7x-21\sqrt{x}-14}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)+\(\dfrac{10x-12\sqrt{x}+2}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)+\(\dfrac{39\sqrt{x}+12}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
=\(\dfrac{-7x-21\sqrt{x}-14+10x-12\sqrt{x}+2+39\sqrt{x}+12}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
=\(\dfrac{3x-6\sqrt{x}}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)=\(\dfrac{3\sqrt{x}\left(\sqrt{x}-2\right)}{\left(5\sqrt{x}-1\right)\left(\sqrt{x}+2\right)}\)
17.

14a) \(M=\sqrt{9+4\sqrt{5}}-\sqrt{9-4\sqrt{5}}\)
\(=\sqrt{\left(\sqrt{5}\right)^2+2.\sqrt{2}.2+2^2}-\sqrt{\left(\sqrt{5}\right)^2-2.\sqrt{2}.2+2^2}\)
\(=\sqrt{\left(\sqrt{5}+2\right)^2}-\sqrt{\left(\sqrt{5}-2\right)^2}=\left|\sqrt{5}+2\right|-\left|\sqrt{5}-2\right|\)
\(=\sqrt{5}+2-\sqrt{5}+2=4\)
b) \(N=\sqrt{8-2\sqrt{7}}-\sqrt{8+2\sqrt{7}}\)
\(=\sqrt{\left(\sqrt{7}\right)^2-2.\sqrt{7}.1+1^2}-\sqrt{\left(\sqrt{7}\right)^2+2.\sqrt{7}.1+1^2}\)
\(=\sqrt{\left(\sqrt{7}-1\right)^2}-\sqrt{\left(\sqrt{7}+1\right)^2}=\left|\sqrt{7}-1\right|-\left|\sqrt{7}+1\right|\)
\(=\sqrt{7}-1-\sqrt{7}-1=-2\)
15a) \(P=\sqrt{11+6\sqrt{2}}-\sqrt{11-6\sqrt{2}}\)
\(=\sqrt{3^2+2.3.\sqrt{2}+\left(\sqrt{2}\right)^2}-\sqrt{3^2-2.3.\sqrt{2}+\left(\sqrt{2}\right)^2}\)
\(=\sqrt{\left(3+\sqrt{2}\right)^2}-\sqrt{\left(3-\sqrt{2}\right)^2}=\left|3+\sqrt{2}\right|-\left|3-\sqrt{2}\right|\)
\(=3+\sqrt{2}-3+\sqrt{2}=2\sqrt{2}\)
b) \(Q=\sqrt{17+12\sqrt{2}}+\sqrt{17-12\sqrt{2}}\)
\(=\sqrt{3^2+2.3.2\sqrt{2}+\left(2\sqrt{2}\right)^2}+\sqrt{3^2-2.3.2\sqrt{2}+\left(2\sqrt{2}\right)^2}\)
\(=\sqrt{\left(3+2\sqrt{2}\right)^2}+\sqrt{\left(3-2\sqrt{2}\right)^2}=\left|3+2\sqrt{2}\right|+\left|3-2\sqrt{2}\right|\)
\(=3+2\sqrt{2}+3-2\sqrt{2}=6\)

`16)(2sqrtx-4)/(3sqrtx-4)-(4+2sqrtx)/(sqrtx-2)+(x+13sqrtx-20)/(3x-10sqrtx+8)`
`=((2sqrtx-4)(sqrtx-2)-(4+2sqrtx)(3sqrtx-4)+x+13sqrtx-20)/((3sqrtx-4)(sqrtx-2)`
`=(2x-8sqrtx+8-6x-4sqrtx+16+x+13sqrtx-20)/((3sqrtx-4)(sqrtx-2)`
`=(-3x+sqrtx+4)/((3sqrtx-4)(sqrtx-2)`
`=(-(3sqrtx-4)(sqrtx+1))/((3sqrtx-4)(sqrtx-2)`
`=(-(sqrtx+1))/(sqrtx-2)`

`15)(-5sqrtx+4)/(3sqrtx-2)+(6sqrtx+4)/(2sqrtx+3)+(29sqrtx-28)/(3(6x+5sqrtx-6))`
`=(3(-5sqrtx+4)(2sqrtx+3)+3(6sqrtx+4)(3sqrtx-2)+29sqrtx-28)/(3(3sqrtx-2)(2sqrtx+3))`
`=(-30x-21sqrtx+36+54x-24+29sqrtx-28)/(3(3sqrtx-2)(2sqrtx+3))`
`=(24x+8sqrtx-16)/(3(3sqrtx-2)(2sqrtx+3))`
`=(8(3sqrtx-2)(sqrtx+1))/(3(3sqrtx-2)(2sqrtx+3))`
`=(8(sqrtx+1))/(3(2sqrtx+3))`

11.
\(=\frac{\sqrt{x}-3}{2-\sqrt{x}}+\frac{\sqrt{x}-2}{3+\sqrt{x}}+\frac{9-x}{(2-\sqrt{x})(\sqrt{x}+3)}\)
\(=\frac{(\sqrt{x}-3)(\sqrt{x}+3)}{(2-\sqrt{x})(\sqrt{x}+3)}+\frac{\sqrt{x}-2}{3+\sqrt{x}}+\frac{9-x}{(2-\sqrt{x})(\sqrt{x}+3)}\)
\(=\frac{x-9}{(2-\sqrt{x})(\sqrt{x}+3)}+\frac{\sqrt{x}-2}{3+\sqrt{x}}+\frac{9-x}{(2-\sqrt{x})(\sqrt{x}+3)}\)
\(=\frac{\sqrt{x}-2}{3+\sqrt{x}}\)
12.
\(=\frac{(3-\sqrt{x})(3\sqrt{x}-2)+(5\sqrt{x}+7)(3\sqrt{x}+4)}{(5\sqrt{x}+7)(3\sqrt{x}-2)}-\frac{42\sqrt{x}+34}{(5\sqrt{x}+7)(3\sqrt{x}-2)}\)
\(=\frac{12x+52\sqrt{x}+22}{(5\sqrt{x}+7)(3\sqrt{x}-2)}-\frac{42\sqrt{x}+34}{(5\sqrt{x}+7)(3\sqrt{x}-2)}\)
\(=\frac{12x+10\sqrt{x}-12}{(5\sqrt{x}+7)(3\sqrt{x}-2)}=\frac{2(3\sqrt{x}-2)(2\sqrt{x}+3)}{(5\sqrt{x}+7)(3\sqrt{x}-2)}=\frac{2(2\sqrt{x}+3)}{5\sqrt{x}+7}\)

ĐKXĐ : \(\left\{{}\begin{matrix}x\ge0\\x\ne49\end{matrix}\right.\)
Ta có : \(\dfrac{7\sqrt{x}-1}{\sqrt{x}-7}-\dfrac{6\sqrt{x}+1}{\sqrt{x}+1}+\dfrac{1-55\sqrt{x}}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{\left(\sqrt{x}+1\right)\left(7\sqrt{x}-1\right)-\left(\sqrt{x}-7\right)\left(6\sqrt{x}+1\right)+1-55\sqrt{x}}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{7x+7\sqrt{x}-\sqrt{x}-1-\left(6x-42\sqrt{x}+\sqrt{x}-7\right)+1-55\sqrt{x}}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{7x+7\sqrt{x}-\sqrt{x}-1-6x+42\sqrt{x}-\sqrt{x}+7+1-55\sqrt{x}}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x-8\sqrt{x}+7}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}=\dfrac{\left(\sqrt{x}-7\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}=\dfrac{\sqrt{x}-1}{\sqrt{x}+1}\)
19) Ta có: \(\dfrac{7\sqrt{x}-1}{\sqrt{x}-7}-\dfrac{6\sqrt{x}+1}{\sqrt{x}+1}+\dfrac{1-55\sqrt{x}}{x-6\sqrt{x}-7}\)
\(=\dfrac{\left(7\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}-\dfrac{\left(6\sqrt{x}+1\right)\left(\sqrt{x}-7\right)}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}+\dfrac{1-55\sqrt{x}}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{7x+7\sqrt{x}-\sqrt{x}-1-6x+42\sqrt{x}-\sqrt{x}+7+1-55\sqrt{x}}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{x-8\sqrt{x}+7}{\left(\sqrt{x}-7\right)\left(\sqrt{x}+1\right)}\)
\(=\dfrac{\sqrt{x}-1}{\sqrt{x}+1}\)

Bài 14:
a)
Sửa đề: \(AE\cdot AB=AD\cdot AC\)
Xét ΔADB vuông tại D và ΔAEC vuông tại E có
\(\widehat{BAD}\) chung
Do đó: ΔADB\(\sim\)ΔAEC(g-g)
Suy ra: \(\dfrac{AD}{AE}=\dfrac{AB}{AC}\)
hay \(AE\cdot AB=AD\cdot AC\)(đpcm)
b) Ta có: \(\dfrac{AD}{AE}=\dfrac{AB}{AC}\)(cmt)
nên \(\dfrac{AD}{AB}=\dfrac{AE}{AC}\)
Xét ΔADB vuông tại D có
\(\cos\widehat{A}=\dfrac{AD}{AB}\)
Xét ΔAED và ΔACB có
\(\dfrac{AD}{AB}=\dfrac{AE}{AC}\)(cmt)
\(\widehat{A}\) chung
Do đó: ΔAED∼ΔACB(c-g-c)
Suy ra: \(\dfrac{AD}{AB}=\dfrac{ED}{CB}\)(Các cặp cạnh tương ứng tỉ lệ)
hay \(\dfrac{AD}{AB}\cdot BC=DE\)
\(\Leftrightarrow DE=BC\cdot\cos\widehat{A}\)(đpcm)
c) Ta có: \(DE=BC\cdot\cos\widehat{A}\)(cmt)
nên \(DE=BC\cdot\cos60^0=\dfrac{1}{2}BC\)(1)
Ta có: ΔEBC vuông tại E(gt)
mà EM là đường trung tuyến ứng với cạnh huyền BC(M là trung điểm của BC)
nên \(EM=\dfrac{1}{2}BC\)(2)
Ta có: ΔDBC vuông tại D(gt)
mà DM là đường trung tuyến ứng với cạnh huyền BC(M là trung điểm của BC)
nên \(DM=\dfrac{1}{2}BC\)(3)
Từ (1), (2) và (3) suy ra ME=MD=DE
hay ΔMDE đều(đpcm)
 giải giúp em bài 14 15 16 17 của bài 14 nha
giải giúp em bài 14 15 16 17 của bài 14 nha giải giúp em bài 14 15 16 17 của bài 14 nha
giải giúp em bài 14 15 16 17 của bài 14 nha giải giúp bài 14 15 16 17 của bài 14
giải giúp bài 14 15 16 17 của bài 14 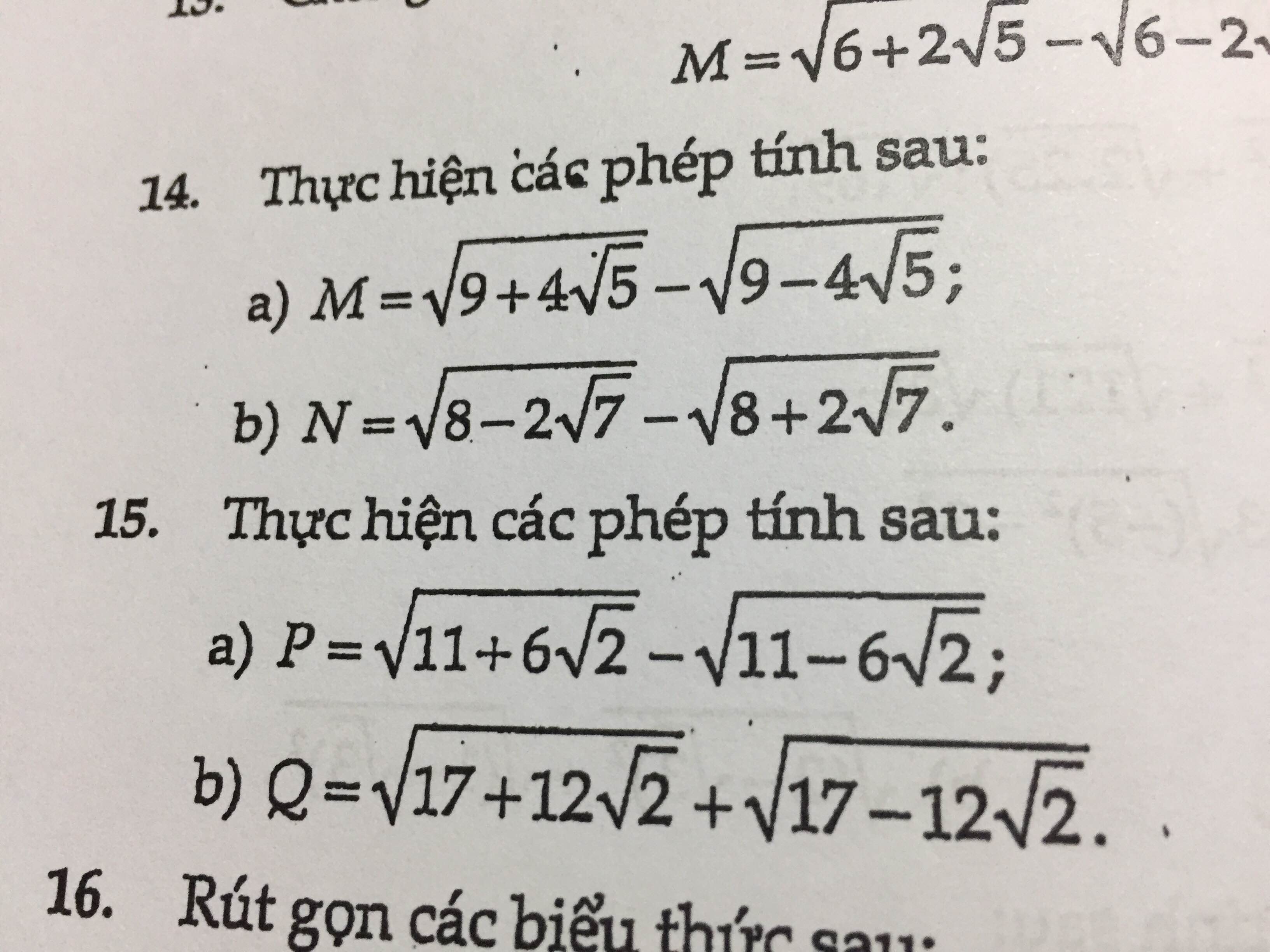
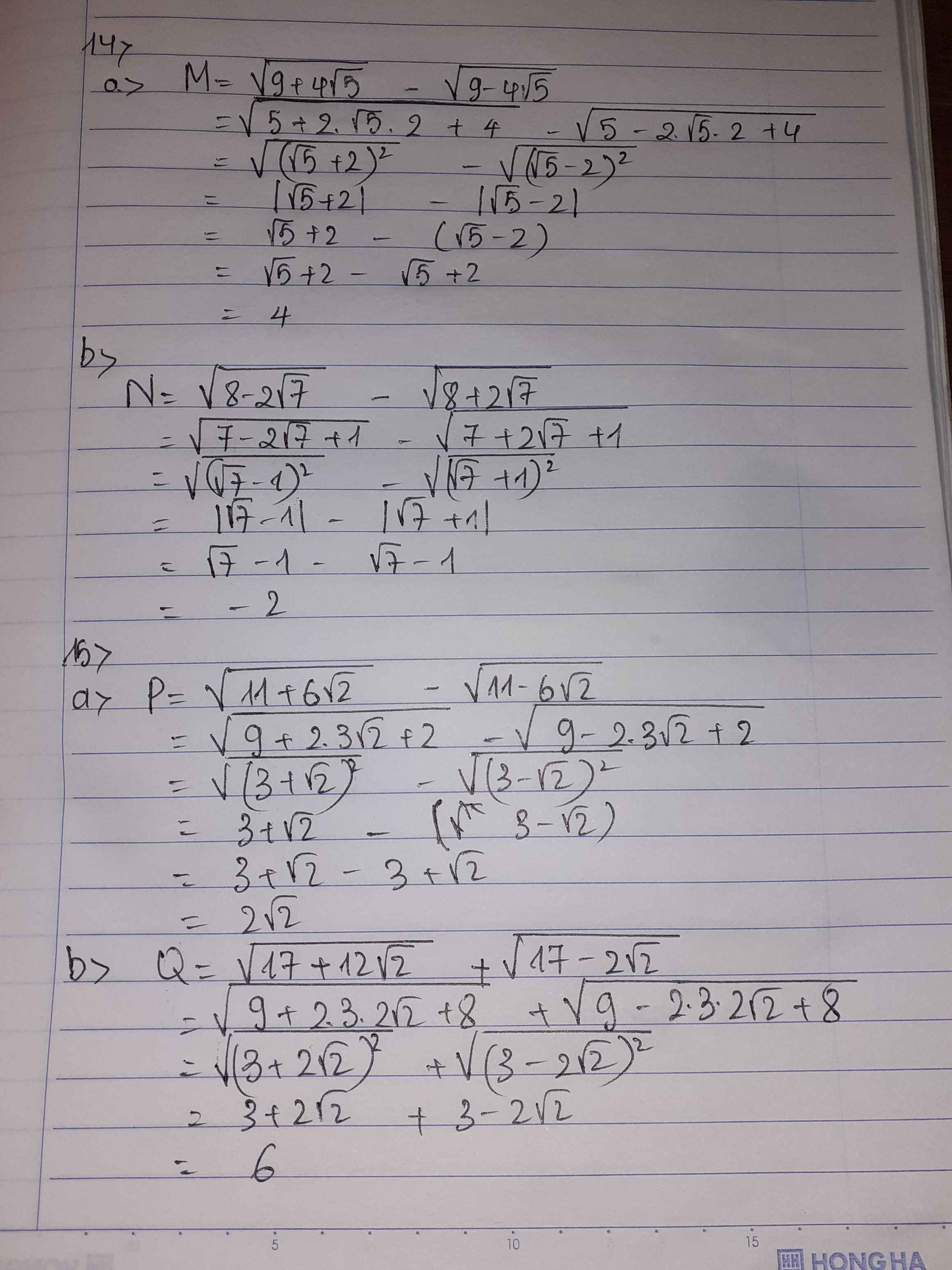
 giải giúp câu 16 của bài 14 i
giải giúp câu 16 của bài 14 i Giải giúp câu 15 của bài 14 i
Giải giúp câu 15 của bài 14 i giải giúp em bài 11 12 13 của bài 14 đi ạ
giải giúp em bài 11 12 13 của bài 14 đi ạ giải giúp bài 19 của bài 14 i
giải giúp bài 19 của bài 14 i
\(\dfrac{3}{1-\sqrt{2}}+\dfrac{\sqrt{2}-1}{\sqrt{2}+1}=\dfrac{3\left(\sqrt{2}+1\right)-\left(\sqrt{2}-1\right)^2}{-1}=-\left(3\sqrt{2}+3-3+2\sqrt{2}\right)=-5\sqrt{2}\)
\(\dfrac{\sqrt{5}-1}{\sqrt{5}+1}+\dfrac{6}{1-\sqrt{5}}=\dfrac{\left(\sqrt{5}-1\right).\left(1-\sqrt{5}\right)+6.\left(\sqrt{5}+1\right)}{-4}=\dfrac{6-2\sqrt{5}-6\sqrt{5}-6}{4}=\dfrac{-8\sqrt{5}}{4}=-2\sqrt{5}\)
\(\dfrac{\sqrt{2}-\sqrt{3}}{2-\sqrt{6}}+\dfrac{\sqrt{3}-\sqrt{2}}{\sqrt{6}+2}=\dfrac{\left(\sqrt{2}-\sqrt{3}\right).\left(\sqrt{6}+2\right)+\left(\sqrt{3}-\sqrt{2}\right).\left(2-\sqrt{6}\right)}{-2}=\dfrac{2\left(\sqrt{12}-\sqrt{18}\right)}{-2}=\sqrt{18}-\sqrt{12}\)
\(\dfrac{-31+8\sqrt{x}-x}{x-8\sqrt{x}+15}-\dfrac{\sqrt{x}+5}{\sqrt{x}-3}-\dfrac{3\sqrt{x}-1}{5-\sqrt{x}}\)
\(=\dfrac{-31+8\sqrt{x}-x}{\left(\sqrt{x}-5\right)\left(\sqrt{x}-3\right)}-\dfrac{\sqrt{x}+5}{\sqrt{x}-3}+\dfrac{3\sqrt{x}-1}{\sqrt{x}-5}\)
\(=\dfrac{-31+8\sqrt{x}-x-x+25+3x-9\sqrt{x}-\sqrt{x}+3}{\left(\sqrt{x}-5\right)\left(\sqrt{x}-3\right)}=\dfrac{x-2\sqrt{x}-3}{\left(\sqrt{x}-5\right)\left(\sqrt{x}-3\right)}=\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-5\right)\left(\sqrt{x}-3\right)}=\dfrac{\sqrt{x}+1}{\sqrt{x}-5}\)
bài 14 mà bạn