K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
CM
26 tháng 1 2017
cosx.tan3x = sin5x
Điều kiện: cos3x ≠ 0. Khi đó,
(3)⇔ cosx.sin3x = cos3x.sin5x
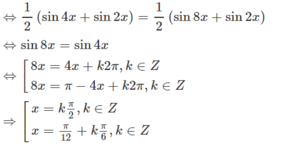
Kết hợp với điều kiện ta được nghiệm của phương trình là:
PT
1
CM
8 tháng 11 2017
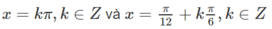
sin 6 x + cos 6 x = 4 cos 2 2 x ⇔ sin 2 x + cos 2 x 3 - 3 sin 2 x . cos 2 x ( sin 2 x + cos 2 x ) = 4 cos 2 2 x
PT
1
CM
16 tháng 2 2017
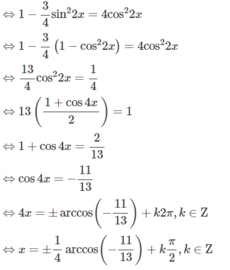
cos 2 x = 3 sin 2 x + 3
Ta thấy cosx = 0 không thỏa mãn phương trình. Với cosx ≠ 0, chia hai vế của phương trình cho cos2x ta được:
PT
1
CM
23 tháng 8 2018
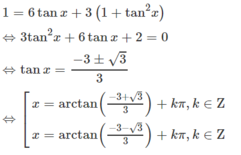
cosx.cos2x = 1 + sinx.sin2x
⇔ cosx.cos2x - sinx.sin2x = 1
⇔ cos3x = 1 ⇔ 3x = k2π
PT
1
CM
13 tháng 1 2019
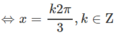
sin 2 x - cos 2 x = cos 4 x ⇔ - cos 2 x = cos 4 x ⇔ 2 cos 3 x . cos x = 0
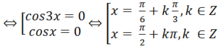
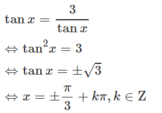
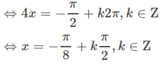
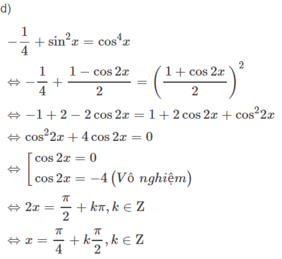
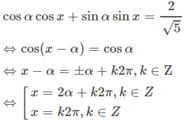
d, \(cosx-cos2x=sin3x\)
\(\Leftrightarrow2sin\dfrac{3x}{2}.sin\dfrac{x}{2}=2sin\dfrac{3x}{2}.cos\dfrac{3x}{2}\)
\(\Leftrightarrow sin\dfrac{3x}{2}.\left(sin\dfrac{x}{2}-cos\dfrac{3x}{2}\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}sin\dfrac{3x}{2}=0\\sin\dfrac{x}{2}=cos\dfrac{3x}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\dfrac{3x}{2}=k\pi\\cos\left(\dfrac{\pi}{2}-\dfrac{x}{2}\right)=cos\dfrac{3x}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{k2\pi}{3}\\x=\dfrac{\pi}{4}-k\pi\\x=-\dfrac{\pi}{2}+k2\pi\end{matrix}\right.\)
e, \(sinx.cosx+\sqrt{3}sin2x=0\)
\(\Leftrightarrow\dfrac{1}{2}sin2x+\sqrt{3}sin2x=0\)
\(\Leftrightarrow\left(\sqrt{3}+\dfrac{1}{2}\right)sin2x=0\)
\(\Leftrightarrow sin2x=0\)
\(\Leftrightarrow2x=k\pi\)
\(\Leftrightarrow x=\dfrac{k\pi}{2}\)