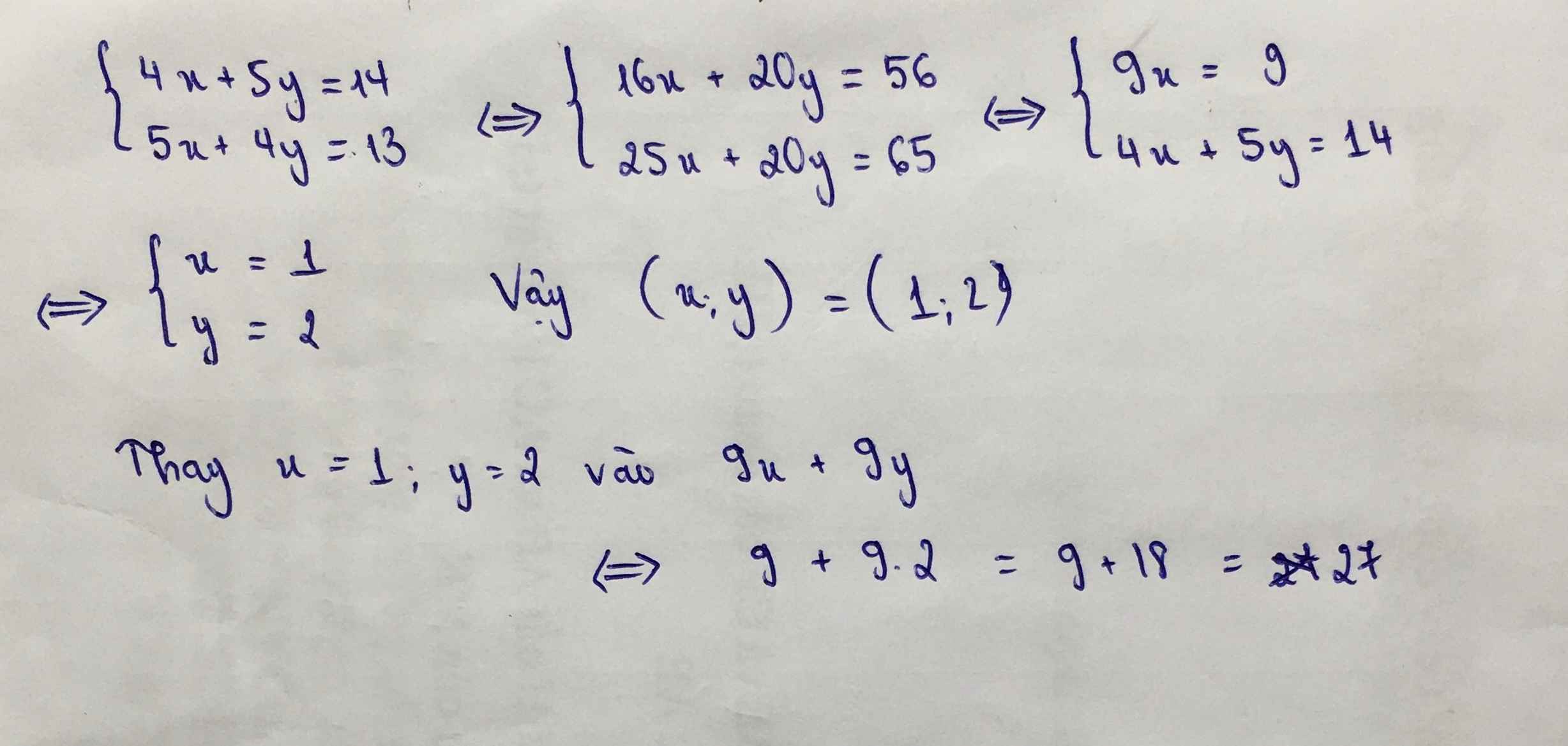Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(\left\{{}\begin{matrix}4x+5y=14\\5x+4y=13\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{14-5y}{4}\\\dfrac{70-25y}{4}+4y=13\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{14-5y}{4}\\\dfrac{70-9y}{4}=13\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{14-5y}{4}\\y=\dfrac{70-\left(13.4\right)}{9}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{14-5.2}{4}\\y=2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=1\\y=2\end{matrix}\right.\)
thay x = 1 ; y=2 và 9x + 9y ta đc
\(\Leftrightarrow9+9.2=9+18=27\)


Ta có:
\(x^2y^2-2x\left(y+2\right)+4=0\)
\(\Leftrightarrow x^2y^2-2xy+4=4x\)
\(\Leftrightarrow\left(xy-1\right)^2+3=4x\)
Mà \(\left(xy-1\right)^2+3>0\)
Nên 4x>0
x>0
Ta có:
\(x^2y^2-2x\left(y+2\right)+4=0\)
\(\Leftrightarrow x^2y^2+4=2x\left(y+2\right)\)
Mà \(x^2y^2+4>0\forall x,y\)
Nên \(2x\left(y+2\right)>0\)
Mặt khác x>0
nên y+2>0
=> y>-2 (1)
Áp dụng bđt Cosi ta có:
\(x^2y^2+4\ge4xy\)
Mà \(\Leftrightarrow x^2y^2+4=2x\left(y+2\right)\)
Nên \(2x\left(y+2\right)\ge4xy\)
\(\Rightarrow y+2\ge2y\)
\(\Leftrightarrow y\le2\) (2)
Do y \(\in Z\) và ta đã có (1), (2)
Nên \(y\in\left\{-1;0;1;2\right\}\)
Th1: y = -1
\(\Rightarrow x^2-2x\left(-1+2\right)+4=0\)
\(\Leftrightarrow x^2-2x+4=0\)
\(\Leftrightarrow\left(x-1\right)^2+3=0\left(vl\right)\)
Th2: y = 0
\(\Rightarrow x^2-2x\left(0+2\right)+4=0\)
\(\Leftrightarrow x^2-4x+4=0\)
\(\Rightarrow x=2\) (nhận)
Th3: y = 1
\(\Rightarrow x^2-2x\left(1+2\right)+4=0\)
\(\Leftrightarrow x^2-6x+4=0\)
\(\Leftrightarrow\left(x-3\right)^2=5\)
\(\Rightarrow\left[{}\begin{matrix}x=\sqrt{5}+3\\x=-\sqrt{5}+3\end{matrix}\right.\)
Loại do x \(\in Z\)
Th4: y = 2
\(\Rightarrow x^2-2x\left(2+2\right)+4=0\)
\(\Leftrightarrow x^2-8x+4=0\)
\(\Rightarrow\left[{}\begin{matrix}x=\sqrt{12}+3\\x=-\sqrt{12}+3\end{matrix}\right.\)
Loại do x \(\in Z\)
Vậy \(\left(x;y\right)\in\left\{2;0\right\}\)

a)
\(\Delta'=\left(-2\right)^2-\left(4m-m^2\right)=4-4m+m^2=\left(m-2\right)^2\ge0\)
Vì \(\Delta'\ge0\) nên phương trình có nghiệm với mọi m
b) Theo Vi-ét có
\(\left\{{}\begin{matrix}x_1+x_2=4\\x_1x_2=4m-m^2\end{matrix}\right.\)
Lấy phương trình đầu của hệ, kết hợp với đề bài, có
\(\left\{{}\begin{matrix}x_1+x_2=4\\x_2=x_1^2-5x_1\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_2=4-x_1\\x_2=x_1^2-5x_1\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_2=4-x_1\\x_1^2-5x_1=4-x_1\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_2=4-x_1\\x^2-4x_1+4=8\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_2=4-x_1\\\left(x_1-2\right)^2=8\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}x_2=4-x_1\\\left[{}\begin{matrix}x_1=2+2\sqrt{2}\\x_1=2-2\sqrt{2}\end{matrix}\right.\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}x_1=2+2\sqrt{2}\\x_2=2+2\sqrt{2}\end{matrix}\right.\\\left\{{}\begin{matrix}x_1=2-2\sqrt{2}\\x_2=2-2\sqrt{2}\end{matrix}\right.\end{matrix}\right.\)
Ta có
\(x_1x_2=4m-m^2\)
Đã tìm được \(x_1\) và \(x_2\) , thay vào để tìm m

\(\left\{{}\begin{matrix}4x+3x=-6\\\dfrac{x+3y}{3}-\dfrac{y-2}{5}=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}7x=-6\\\dfrac{5\left(x+3y\right)-3\left(y-2\right)}{15}=1\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=-\dfrac{6}{7}\\5x+15y-3y+6=15\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=-\dfrac{6}{7}\\12y=9-5x=9+5\cdot\dfrac{6}{7}=9+\dfrac{30}{7}=\dfrac{93}{7}\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=-\dfrac{6}{7}\\y=\dfrac{93}{7\cdot12}=\dfrac{93}{84}=\dfrac{31}{28}\end{matrix}\right.\)