Question

[Linh Lê]

Đổi biến u=lnx thì tích phân từ 1 đến e của (1-lnx)/x^2 thành

Answer 1

[Akai Haruma]

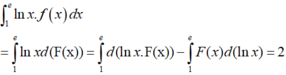
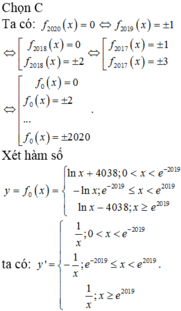
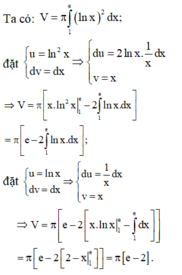
Lời giải:

\(I=\int ^{e}_{1}\frac{1-\ln x}{x^2}dx=\int ^{e}_{1}\frac{dx}{x^2}-\int ^{e}_{1}\frac{\ln x}{x^2}dx\)

Ta có \(\int ^{e}_{1}\frac{dx}{x^2}=\left.\begin{matrix} e\\ 1\end{matrix}\right|\frac{-1}{x}=\frac{-1}{e}+1\)

Đặt \(\left\{\begin{matrix} u=\ln x\\ dv=\frac{dx}{x^2}\end{matrix}\right.\)\(\Rightarrow \left\{\begin{matrix} du=\frac{dx}{x}\\ v=\frac{-1}{x}\end{matrix}\right.\)

\(\Rightarrow \int ^{e}_{1}\frac{\ln xdx}{x^2}=\frac{-\ln x}{x}+\int ^{e}_{1}\frac{dx}{x^2}=\left.\begin{matrix} e\\ 1\end{matrix}\right|\left ( \frac{-\ln x}{x}-\frac{1}{x} \right )=1-\frac{2}{e}\)

Do đó mà \(I=1-\frac{1}{e}-(1-\frac{2}{e})=\frac{1}{e}\)