Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Do \(x\ge0\Rightarrow2x+2+5\sqrt{x}\ge0+2+0=2>0\Rightarrow\dfrac{1}{2x+2+5\sqrt{x}}>0\)
\(2\sqrt{x^2+4x+1}+\sqrt{x}\ge2\sqrt{0+4.0+1}+0=2>0\Rightarrow\dfrac{1}{2\sqrt{x^2+4x+1}+\sqrt{x}}>0\)
\(\Rightarrow\dfrac{1}{2x+2+5\sqrt{x}}+\dfrac{1}{2\sqrt{x^2+4x+1}+\sqrt{x}}>0\)

Bài 2
b, `\sqrt{3x^2}=x+2` ĐKXĐ : `x>=0`
`=>(\sqrt{3x^2})^2=(x+2)^2`
`=>3x^2=x^2+4x+4`
`=>3x^2-x^2-4x-4=0`
`=>2x^2-4x-4=0`
`=>x^2-2x-2=0`
`=>(x^2-2x+1)-3=0`
`=>(x-1)^2=3`
`=>(x-1)^2=(\pm \sqrt{3})^2`
`=>` $\left[\begin{matrix} x-1=\sqrt{3}\\ x-1=-\sqrt{3}\end{matrix}\right.$
`=>` $\left[\begin{matrix} x=1+\sqrt{3}\\ x=1-\sqrt{3}\end{matrix}\right.$
Vậy `S={1+\sqrt{3};1-\sqrt{3}}`

a: Khi x=25 thì \(A=\dfrac{7\cdot5-2}{5-2}=\dfrac{33}{3}=11\)
b: P=A*B
\(=\left(\dfrac{\sqrt{x}}{\sqrt{x}+1}+\dfrac{2}{\sqrt{x}-1}-\dfrac{4\sqrt{x}}{x-1}\right)\cdot\dfrac{7\sqrt{x}-2}{\sqrt{x}-2}\)
\(=\dfrac{x-\sqrt{x}+2\sqrt{x}+2-4\sqrt{x}}{x-1}\cdot\dfrac{7\sqrt{x}-2}{\sqrt{x}-2}\)
\(=\dfrac{x-3\sqrt{x}+2}{x-1}\cdot\dfrac{7\sqrt{x}-2}{\sqrt{x}-2}\)
\(=\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)\cdot\left(7\sqrt{x}-2\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{7\sqrt{x}-2}{\sqrt{x}+1}\)

\(a,x=16\Rightarrow A=\dfrac{\sqrt{16}+2}{\sqrt{16}-3}=\dfrac{4+2}{4-3}=6\)
\(b,B=\dfrac{\sqrt{x}+5}{\sqrt{x}+1}+\dfrac{\sqrt{x}-7}{1-x}\left(dk:x\ge0,x\ne1,x\ne9\right)\\ =\dfrac{\sqrt{x}+5}{\sqrt{x}+1}-\dfrac{\sqrt{x}-7}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ =\dfrac{\left(\sqrt{x}+5\right)\left(\sqrt{x}-1\right)-\left(\sqrt{x}-7\right)}{x-1}\\ =\dfrac{x+4\sqrt{x}-5-\sqrt{x}+7}{x-1}\\ =\dfrac{x+3\sqrt{x}+2}{x-1}\\ =\dfrac{\left(\sqrt{x}+2\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\\ =\dfrac{\sqrt{x}+2}{\sqrt{x}-1}\left(dpcm\right)\)
\(c,\dfrac{4A}{A}\le\dfrac{x}{\sqrt{x}-3}\Leftrightarrow\dfrac{4\left(\sqrt{x}+2\right)}{\sqrt{x}-3}:\dfrac{\sqrt{x}+2}{\sqrt{x}-3}\le\dfrac{x}{\sqrt{x}-3}\)
\(\Leftrightarrow\dfrac{4\left(\sqrt{x}+2\right)}{\sqrt{x}-3}.\dfrac{\sqrt{x}-3}{\sqrt{x}+2}\le\dfrac{x}{\sqrt{x}-3}\)
\(\Leftrightarrow4-\dfrac{x}{\sqrt{x}-3}\le0\)
\(\Leftrightarrow\dfrac{4\sqrt{x}-12-x}{\sqrt{x}-3}\le0\)
\(\Leftrightarrow\) Pt vô nghiệm
Vậy không có giá trị x thỏa yêu cầu đề bài.

a: \(A=\dfrac{2x-6\sqrt{x}+x+4\sqrt{x}+3-3+11\sqrt{x}}{x-9}\)
\(=\dfrac{3\sqrt{x}}{\sqrt{x}-3}\)

a, Khi x = 2, ta được:
\(A=\dfrac{4}{2\sqrt{2}-2}=2+2\sqrt{2}\)
b, \(B=\dfrac{\sqrt{x}-4}{x-2\sqrt{x}}+\dfrac{3}{\sqrt{x}-2}\\ \Rightarrow B=\dfrac{\sqrt{x}-4+3\sqrt{x}}{\sqrt{x}\left(\sqrt{x}-1\right)}\\ \Rightarrow B=\dfrac{4\left(\sqrt{x}-1\right)}{\sqrt{x}\left(\sqrt{x}-1\right)}\)
\(P=B:A=\dfrac{4\left(\sqrt{x}-1\right)}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\sqrt{x}\left(2-\sqrt{x}\right)}{4}=-\left(\sqrt{x}-1\right)=1-\sqrt{x}\) (đpcm)

1, Thay x = 16 vào ta được \(A=\dfrac{4}{4+3}=\dfrac{4}{7}\)
2, \(A+B=\dfrac{\sqrt{x}}{\sqrt{x}+3}+\dfrac{2\sqrt{x}\left(\sqrt{x}+3\right)-3x-9}{x-9}=\dfrac{\sqrt{x}}{\sqrt{x}+3}+\dfrac{-x+6\sqrt{x}-9}{x-9}=\dfrac{\sqrt{x}}{\sqrt{x}+3}-\dfrac{\sqrt{x}-3}{\sqrt{x}+3}=\dfrac{3}{\sqrt{x}+3}\)
Ta có đpcm


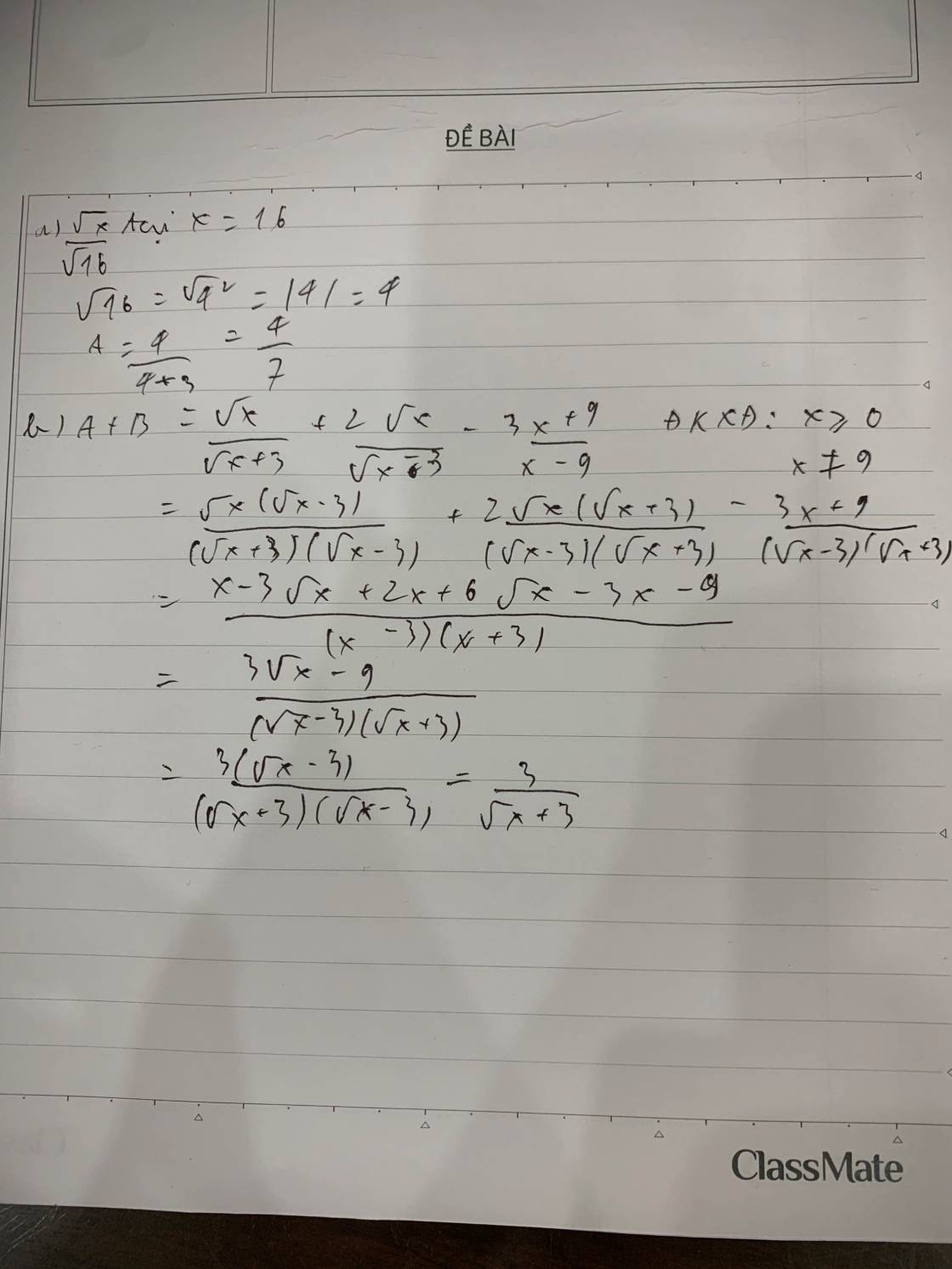
`x=4=>B=(4+3)/(4-1)=7/3>0`
Vậy đề bài sai