Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(\lim\limits_{x\rightarrow0^+}f\left(x\right)=\lim\limits_{x\rightarrow0^+}\dfrac{\sqrt{x+4}-2}{x}=\lim\limits_{x\rightarrow0^+}\dfrac{x}{x\left(\sqrt{x+4}+2\right)}=\lim\limits_{x\rightarrow0^+}\dfrac{1}{\sqrt{x+4}+2}=\dfrac{1}{4}\)
\(f\left(0\right)=\lim\limits_{x\rightarrow0^-}f\left(x\right)=\lim\limits_{x\rightarrow0^-}\left(mx^2+2m+\dfrac{1}{4}\right)=2m+\dfrac{1}{4}\)
Hàm liên tục tại x=0 khi: \(\lim\limits_{x\rightarrow0^+}f\left(x\right)=\lim\limits_{x\rightarrow0^-}f\left(x\right)=f\left(0\right)\)
\(\Leftrightarrow2m+\dfrac{1}{4}=\dfrac{1}{4}\Leftrightarrow m=0\)

\(\Leftrightarrow\left|x^2-4\left|x\right|+2\right|=m\) (1) có 8 nghiệm phân biệt
Đặt \(x^2-4\left|x\right|+2=t\) (2)
Từ đồ thị của hàm \(y=x^2-4\left|x\right|+2\) ta thấy:
- Với \(t< -2\Rightarrow\) (2) vô nghiệm
- Với \(\left[{}\begin{matrix}t=-2\\t>2\end{matrix}\right.\Rightarrow\) (2) có 2 nghiệm
- Với \(-2< t< 2\Rightarrow\) (2) có 4 nghiệm
- Với \(t=2\Rightarrow\) (2) có 3 nghiệm
Khi đó (1) trở thành: \(\left|t\right|=m\) (3) có tối đa 2 nghiệm
\(\Rightarrow\)Phương trình đã cho có 8 nghiệm pb khi và chỉ khi (3) có 2 nghiệm t phân biệt thỏa mãn \(-2< t< 2\)
\(\Rightarrow0< m< 2\)
Không có phương án nào đúng

\(y'=4mx^3+2mx=2mx\left(2x^2+1\right)\)
Do \(2x\left(x^2+1\right)>0\) ;\(\forall x>0\)
\(\Rightarrow y'\ge0\) ;\(\forall x>0\) khi và chỉ khi \(m>0\)

\(y'=x^2-2x+m\)
\(y'\ge0\) ; \(\forall x\in\left(1;3\right)\Leftrightarrow x^2-2x+m\ge0\) ;\(\forall x\in\left(1;3\right)\)
\(\Leftrightarrow m\ge\max\limits_{\left(1;3\right)}\left(-x^2+2x\right)\)
Xét hàm \(f\left(x\right)=-x^2+2x\) trên \(\left(1;3\right)\)
\(-\dfrac{b}{2a}=1\) ; \(f\left(1\right)=1\) ; \(f\left(3\right)=-3\)
\(\Rightarrow m\ge1\)

\(f'\left(x\right)=4x\Rightarrow y=2x^2+1-4x\)
\(y'\left(x\right)=4x-4=0\Rightarrow x=1\)

\(f^3\left(2-x\right)-2f^2\left(2+3x\right)+x^2g\left(x\right)+36x=0\) (1)
Thay \(x=0\Rightarrow f^3\left(2\right)-2f^2\left(2\right)=0\Rightarrow\left[{}\begin{matrix}f\left(2\right)=0\\f\left(2\right)=2\end{matrix}\right.\)
Đạo hàm 2 vế của (1):
\(\Rightarrow-3f^2\left(2-x\right).f'\left(2-x\right)-12f\left(2+3x\right).f'\left(2+3x\right)+2x.g\left(x\right)+x^2.g'\left(x\right)+36=0\)
Thay \(x=0\)
\(\Rightarrow-3f^2\left(2\right).f'\left(2\right)-12f\left(2\right).f'\left(2\right)+36=0\)
TH1: \(f\left(2\right)=0\Rightarrow36=0\) (ktm)
TH2: \(f\left(2\right)=2\)
\(\Rightarrow-3.2^2.f'\left(2\right)-12.2.f'\left(2\right)+36=0\Rightarrow f'\left(2\right)=1\)
\(\Rightarrow A=3.2+4.1=10\)
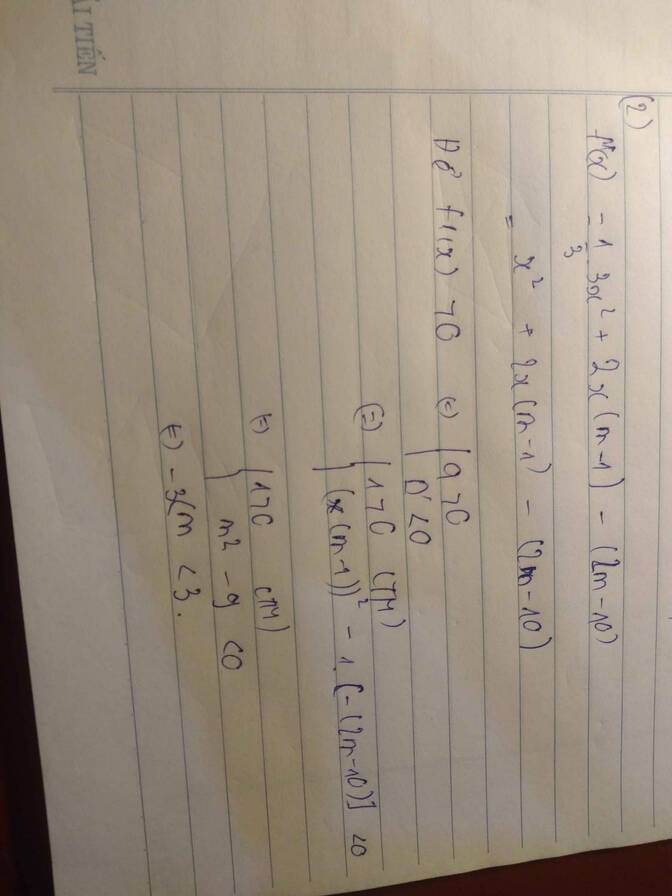
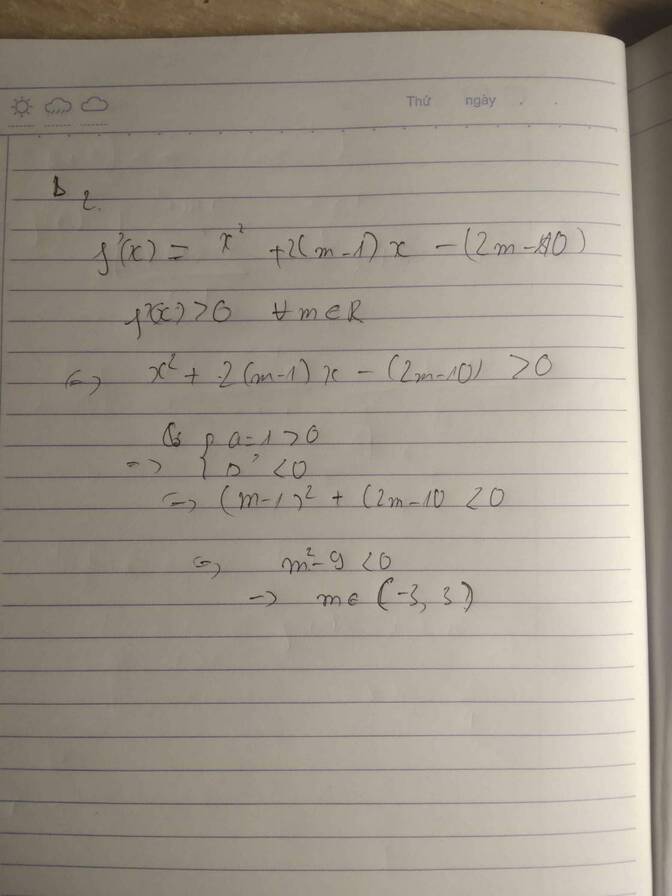
TH1: \(m=3\Rightarrow f\left(x\right)=-5< 0\) với mọi x(ktm)
TH2: \(m>3\Rightarrow f\left(x\right)\) đồng biến trên R
\(\Rightarrow\min\limits_{\left[3;4\right]}f\left(x\right)=f\left(3\right)=3\left(m-3\right)-2m+1=m-8\)
\(m-8>0\Rightarrow m>8\)
TH3: \(m< 3\Rightarrow f\left(x\right)\) nghịch biến trên R
\(\Rightarrow\min\limits_{\left[3;4\right]}=f\left(4\right)=4\left(m-3\right)-2m+1=2m-11\)
\(2m-11>0\Rightarrow m>\dfrac{11}{2}\) (ktm điều kiện \(m< 3\))
Kết hợp lại ta được \(m>8\)