Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Tham khảo:
a) Ta có: \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \overrightarrow 0 \Leftrightarrow \overrightarrow {MA} + \left( {\overrightarrow {MA} + \overrightarrow {AB} } \right) + 2\left( {\overrightarrow {MA} + \overrightarrow {AC} } \right) = \overrightarrow 0 \)
\(\begin{array}{l} \Leftrightarrow 4\overrightarrow {MA} + \overrightarrow {AB} + 2\overrightarrow {AC} = \overrightarrow 0 \\ \Leftrightarrow 4\overrightarrow {AM} = \overrightarrow {AB} + 2\overrightarrow {AC} \\ \Leftrightarrow \overrightarrow {AM} = \frac{1}{4}\overrightarrow {AB} + \frac{1}{2}\overrightarrow {AC} \end{array}\)
Trên cạnh AB, AC lấy điểm D, E sao cho \(AD = \frac{1}{4}AB;\;\,AE = \frac{1}{2}AC\)
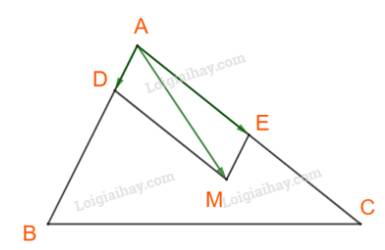
Khi đó \(\overrightarrow {AM} = \overrightarrow {AD} + \overrightarrow {AE} \) hay M là đỉnh thứ tư của hình bình hành AEMD.
b) Chứng minh rằng với mọi điểm O, ta có \(\overrightarrow {OA} + \overrightarrow {OB} + 2\overrightarrow {OC} = 4\overrightarrow {OM} \)
Với mọi điểm O, ta có: \(\left\{ \begin{array}{l}\overrightarrow {OA} = \overrightarrow {OM} + \overrightarrow {MA} ;\;\\\overrightarrow {OB} = \overrightarrow {OM} + \overrightarrow {MB} ;\;\,\\\overrightarrow {OC} = \overrightarrow {OM} + \overrightarrow {MC} \end{array} \right.\)
\(\begin{array}{l} \Rightarrow \overrightarrow {OA} + \overrightarrow {OB} + 2\overrightarrow {OC} = \left( {\overrightarrow {OM} + \overrightarrow {MA} } \right) + \left( {\overrightarrow {OM} + \overrightarrow {MB} } \right) + 2\left( {\overrightarrow {OM} + \overrightarrow {MC} } \right)\\ = 4\overrightarrow {OM} + \left( {\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} } \right) = 4\overrightarrow {OM} + \overrightarrow 0 = 4\overrightarrow {OM} .\end{array}\)
Vậy với mọi điểm O, ta có \(\overrightarrow {OA} + \overrightarrow {OB} + 2\overrightarrow {OC} = 4\overrightarrow {OM} \).
Tham khảo cách 2 câu a:
Cách 2:
Ta có: \(\overrightarrow {MA} + \overrightarrow {MB} + 2\overrightarrow {MC} = \overrightarrow 0 \Leftrightarrow \left( {\overrightarrow {MC} + \overrightarrow {CA} } \right) + \left( {\overrightarrow {MC} + \overrightarrow {CB} } \right) + 2\overrightarrow {MC} = \overrightarrow 0 \)
\(\begin{array}{l} \Leftrightarrow 4\overrightarrow {MC} + \overrightarrow {CA} + \overrightarrow {CB} = \overrightarrow 0 \\ \Leftrightarrow 4.\overrightarrow {CM} = \overrightarrow {CA} + \overrightarrow {CB} \end{array}\)
Gọi D là đỉnh thứ tư của hình bình hành ACBD.
Khi đó: \(\overrightarrow {CD} = \overrightarrow {CA} + \overrightarrow {CB} \)\( \Rightarrow 4.\overrightarrow {CM} = \overrightarrow {CD} \)
\( \Leftrightarrow \overrightarrow {CM} = \frac{1}{4}\overrightarrow {CD} \Leftrightarrow \overrightarrow {CM} = \frac{1}{2}\overrightarrow {CO} \)
Với O là tâm hình bình hành ACBD, cũng là trung điểm đoạn AB.
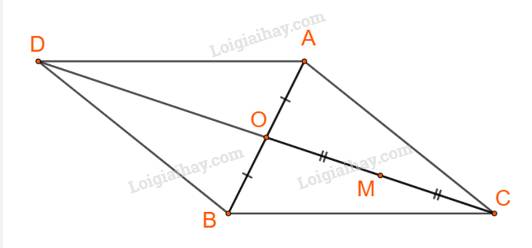
Vậy M là trung điểm của trung tuyến kẻ từ C của tam giác ABC.

a) gọi I là trung điểm của đoạn thẳng AB
=> IA+ IB=0
| 2MI|= |BA|
|MI|= 1/2|BA|
=> M thuộc đường tròn tâm I, bán kính =1/2 BA
B) gọi G là trọng tâm của tam giác ABC
=> GA+ GB+ GC=0
gọi I là trung điểm của đoạn thẳng AB
=> IA+ IB=0
| 3MG|= 3/2| 2 MI|
3| MG|= 3| MI|
| MG|= | MI|
=> M thuộc đường trung trực của đoạn thẳng GI

Gọi G là trọng tâm của tam giác ABC, I là trung điểm BC.
Dễ dàng chứng minh \(\left\{{}\begin{matrix}\left|\overrightarrow{MA}+\overrightarrow{MB}+\overrightarrow{MC}\right|=\left|3\overrightarrow{MG}\right|=3MG\\\dfrac{3}{2}\left|\overrightarrow{MB}+\overrightarrow{MC}\right|=\dfrac{3}{2}\left|2\overrightarrow{MI}\right|=3MI\end{matrix}\right.\)
Kết hợp điều kiện đề bài, ta có \(MG=MI\). Do đó M nằm trên đường trung trực của GI (cố định).
Vậy tập hợp điểm M thoả điều kiện đề bài là trung trực của đoạn GI.

\(\overrightarrow{AM}=\overrightarrow{AB}+\overrightarrow{BM}\)
\(=\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{BC}\)
\(=\overrightarrow{AB}+\dfrac{2}{3}\left(\overrightarrow{BA}+\overrightarrow{AC}\right)\)
\(=\dfrac{1}{3}\overrightarrow{AB}+\dfrac{2}{3}\overrightarrow{AC}\)

Chắc chắn là đề bài sai rồi
Vế trái là 1 đại lượng vô hướng
Vế phải là 1 đại lượng có hướng (vecto)
Hai vế không thể bằng nhau được

a: \(\overrightarrow{AM}+\overrightarrow{BN}=\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{BC}=\dfrac{1}{2}\overrightarrow{AC}\)
b: \(=\dfrac{1}{2}\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{BA}\)
\(=\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{BA}\)
c: \(\overrightarrow{AM}+\overrightarrow{BN}+\overrightarrow{CP}\)
\(=\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{BC}+\dfrac{1}{2}\overrightarrow{CA}\)
\(=\dfrac{1}{2}\left(\overrightarrow{AC}+\overrightarrow{CA}\right)=\overrightarrow{0}\)

1)\(VT=\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}+\overrightarrow{OD}=\overrightarrow{CO}+\overrightarrow{DO}+\overrightarrow{OC}+\overrightarrow{OC}=\overrightarrow{CO}+\overrightarrow{OC}+\overrightarrow{DO}+\overrightarrow{OD}=\overrightarrow{0}\)
2)\(VT=\overrightarrow{DA}-\overrightarrow{DB}+\overrightarrow{DC}=\overrightarrow{BA}+\overrightarrow{DC}=\overrightarrow{0}\)
3)\(VT=\overrightarrow{DO}+\overrightarrow{AO}=\overrightarrow{OB}+\overrightarrow{AO}=\overrightarrow{AB}\)
4)\(\overrightarrow{MA}+\overrightarrow{MC}=\overrightarrow{MB}+\overrightarrow{BA}+\overrightarrow{MD}+\overrightarrow{DC}=\overrightarrow{MB}+\overrightarrow{MD}\left(đpcm\right)=\overrightarrow{MO}+\overrightarrow{OB}+\overrightarrow{MO}+\overrightarrow{OD}=2\overrightarrow{MO}\left(đpcm\right)\)
Chúc bạn học tốt!!!!!
Đăng kí kênh Youtube 'Ban Mai Anime' giúp mình nhé!!!!