LL
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
AH
Akai Haruma
Giáo viên
14 tháng 8 2023
Lời giải:
Ta có:
$\frac{5}{13}=\cos A=\frac{AC}{AB}$
$\Rightarrow AB=\frac{13}{5}AC$
Áp dụng định lý Pitago:
$AC^2+BC^2=AB^2$
$\Leftrightarrow AC^2+10^2=(\frac{13}{5}AC)^2$
$\Leftrightarrow 100=\frac{144}{25}AC^2$
$\Leftrightarrow AC^2=\frac{625}{36}$
$\Rightarrow AC=\frac{25}{6}$ (cm)
Vậy......
NY
1
ML
28 tháng 6 2015
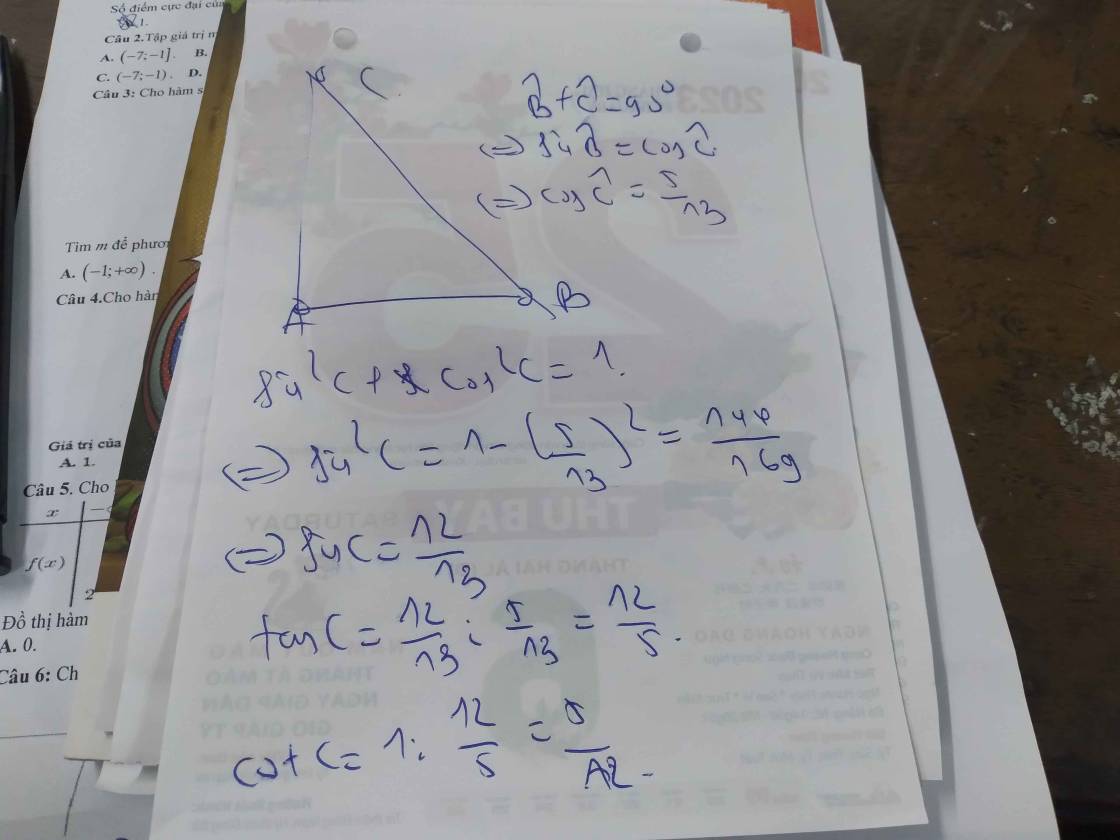
Tam giác ABC vuông tại C
=> \(\sin B=\cos A=\frac{5}{15}\)
\(\sin^2B+\cos^2B=1\Rightarrow\cos B=\sqrt{1-\sin^2B}=\sqrt{1-\frac{5}{13}}=\sqrt{\frac{8}{13}}\)
\(\tan B=\frac{\sin B}{\cos B}=\frac{\frac{5}{13}}{\sqrt{\frac{8}{13}}}=\frac{\sqrt{26}}{12}\) \(\cot B=\frac{1}{\tan B}=\frac{12}{\sqrt{26}}\)
PN
1
TP
21 tháng 9 2021
ta có:cosB=\(\dfrac{AB}{BC}=\dfrac{5}{13}\)⇒BC=39
AC=\(\sqrt{BC^2-AB^2}=\sqrt{39^2-15^2}\)=36
9 tháng 10 2021
\(\sin^2\widehat{A}+\cos^2\widehat{A}=1\Leftrightarrow\cos^2\widehat{A}=1-\left(\dfrac{3}{5}\right)^2=1-\dfrac{9}{25}=\dfrac{16}{25}\\ \Leftrightarrow\cos\widehat{A}=\dfrac{4}{5}\\ \tan\widehat{A}=\dfrac{\sin\widehat{A}}{\cos\widehat{A}}=\dfrac{3}{4}\\ \Rightarrow\cot\widehat{A}=\dfrac{1}{\tan\widehat{A}}=\dfrac{4}{3}\)
D
1
CM
9 tháng 8 2018
Vì tam giác ABC vuông tại A nên B ^ + C ^ = 90 o
=> cot C = tan B = 4
Mà cot C. tan C = 1 => tan C = 1 4
Đáp án cần chọn là: A
\(\sin\widehat{A}=\sqrt{1-\left(\dfrac{5}{13}\right)^2}=\dfrac{12}{13}\)
\(\cot\widehat{A}=\dfrac{5}{13}:\dfrac{12}{13}=\dfrac{5}{12}\)
\(\tan\widehat{B}=\dfrac{5}{12}\)