Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

c) Theo hệ thức Vi- et ta có:
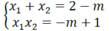
A = x 1 2 + x 2 2 - 6 x 1 x 2 = x 1 + x 2 2 - 8 x 1 x 2
= 2 - m 2 - 8(-m + 1) = m 2 - 4m + 4 + 8m - 8
= m 2 + 4m - 4 = m + 2 2 - 8
Ta có: (m + 2)2 ≥ 0 ∀ m
⇒ m + 2 2 - 8 ≥ -8 ∀ m ⇔ A ≥ -8 ∀ m
Dấu bằng xảy ra khi m + 2 2 = 0 ⇔ m= -2
Vậy GTNN của A là -8, đạt được khi m = -2

b) Theo định lí Vi-et ta có:
x 1 + x 2 = m + 1 và x 1 . x 2 = m - 2
Do đó A = x 1 2 + x 2 2 - 6 x 1 x 2 = x 1 + x 2 2 - 8 x 1 x 2
= m + 1 2 - 8(m – 2) = m 2 + 2m + 1 – 8m + 16
= m 2 - 6m + 17 = m - 3 2 + 8 ≥ 8
Vậy giá trị nhỏ nhất của A bẳng 8 khi m – 3 = 0 hay m = 3.

Theo định lý Vi-ét, ta có:
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m+1\right)\\x_1.x_2=2m-2\end{matrix}\right.\)
Ta có: \(x_1^2+2\left(m+1\right)x_2+2m-2\)\(=x1^2+x_1+x_2.x_2+x_1.x_2\)
\(=x_1^2+2x_1x_2+x_2^2=\left(x_1+x_2\right)^2\) \(=\left[2\left(m+1\right)\right]^2=4\left(m+1\right)^2\)
Ta có: \(4\left(m+1\right)^2=9\Leftrightarrow\left(m+1\right)^2=\dfrac{9}{4}\) \(\Leftrightarrow\left[{}\begin{matrix}m+1=\dfrac{3}{2}\\m+1=\dfrac{-3}{2}\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}m=\dfrac{1}{2}\\m=\dfrac{-5}{2}\end{matrix}\right.\)
Vậy \(m=\dfrac{1}{2};m=\dfrac{-5}{2}\) thoả mãn yêu cầu đề bài
Dấu bằng thứ nhất sau chữ ta có đầu tiên sửa thành: \(x_1^2+\left(x_1+x_2\right).x_2+x_1x_2\)

a: Δ=(2m+2)^2-4(m-2)
=4m^2+8m+4-4m+8
=4m^2+4m+12
=(2m+1)^2+11>=11>0
=>Phương trình luôn cóhai nghiệm phân biệt
b: x1^2+2(m+1)x2-5m+2
=x1^2+x2(x1+x2)-4m-m+2
=x1^2+x1x2+x2^2-5m+2
=(x1+x2)^2-2x1x2+x1x2-5m+2
=(2m+2)^2-(m-2)-5m+2
=4m^2+8m+4-m+2-5m+2
=4m^2+2m+8
=4(m^2+1/2m+2)
=4(m^2+2*m*1/4+1/16+31/16)
=4(m+1/4)^2+31/4>=31/4
Dấu = xảy ra khi m=-1/4

a/ Xét pt :
\(x^2-2\left(m-1\right)+2m-5=0\)
\(\Delta'=\left(m-1\right)^2-\left(2m-5\right)=m^2-2m+1-2m+5=m^2-4m+6=\left(m-2\right)^2+2>0\forall m\)
\(\Leftrightarrow\) pt luôn có 2 nghiệm pb với mọi m
b/ Phương trình cớ 2 nghiệm trái dấu
\(\Leftrightarrow2m-5< 0\)
\(\Leftrightarrow m< \dfrac{5}{2}\)
c/ Theo định lí Vi - et ta có :
\(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1.x_2=2m-5\end{matrix}\right.\)
\(A=x_1^2+x_2^2\)
\(=\left(x_1+x_2\right)^2-2x_1.x_2\)
\(=4\left(m-1\right)^2-2\left(2m-5\right)\)
\(=4m^2-8m+4-4m+10\)
\(=4m^2-12m+14=4\left(m^2-3m+\dfrac{9}{4}\right)+5=4\left(m-\dfrac{3}{2}\right)^2+5\ge5\)
\(A_{min}=5\Leftrightarrow m=\dfrac{3}{2}\)
1, \(\Delta'=\left(m-1\right)^2-\left(2m-5\right)=m^2-4m+6=\left(m-2\right)^2+2>0\)
Vậy pt luôn có 2 nghiệm pb với mọi m
2, Vì pt có 2 nghiệm trái dấu
\(x_1x_2=\dfrac{c}{a}=2m-5< 0\Leftrightarrow m< \dfrac{5}{2}\)
3, Theo Vi et \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1x_2=2m-5\end{matrix}\right.\)
\(A=\left(x_1+x_2\right)^2-2x_1x_2=4\left(m-1\right)^2-2\left(2m-5\right)\)
\(=4m^2-12m+14=4m^2-2.2m.3+9+6\)
\(=\left(2m-3\right)^2+6\ge6\forall m\)
Dấu ''='' xảy ra khi m = 3/2
Vậy với m = 3/2 thì A đạt GTNN tại 6

a, Với x=2
PT<=> 4+2(m-2)-m+1=0
<=> m=-1
Vậy m=-1 thì phương trình có 1 nghiệm x=2
Ý sau dùng hệ thức Vi-et là ra

a: \(\Delta=\left(2m+2\right)^2-4\left(m^2-2m-3\right)\)
\(=4m^2+8m+4-4m^2+8m+12\)
=16m+16
Để phương trình luôn có nghiệm thì 16m+16>=0
hay m>=-1
b: Theo đề, ta có: \(\left(x_1+x_2\right)^2-2x_1x_2-x_1x_2=28\)
\(\Leftrightarrow\left(2m+2\right)^2-3\left(m^2-2m-3\right)=28\)
\(\Leftrightarrow4m^2+8m+4-3m^2+6m+9=28\)
\(\Leftrightarrow m^2+14m-15=0\)
=>(m+15)(m-1)=0
=>m=1

a: Khi m=2 thì (1) sẽ là x^2-5x+4=0
=>x=1; x=4
b: Δ=(-5)^2-4(m+2)=25-4m-8=17-4m
Để (1) có hai nghiệm phân biệt thì 17-4m>0
=>m<17/4

\(\Delta'=\left(m-1\right)^2+m+3=m^2-m+4=\left(m-\dfrac{1}{2}\right)^2+\dfrac{7}{2}>0;\forall m\)
\(\Rightarrow\) Phương trình luôn có 2 nghiệm với mọi m
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-1\right)\\x_1x_2=-m-3\end{matrix}\right.\)
a.
\(A=x_1^2+x_2^2=\left(x_1+x_2\right)^2-2x_1x_2\)
\(=4\left(m-1\right)^2+2\left(m+3\right)=4m^2-6m+10\)
\(=4\left(m-\dfrac{3}{4}\right)^2+\dfrac{31}{4}\ge\dfrac{3}{4}\)
Dấu = xảy ra khi \(m=\dfrac{3}{4}\)
b.
\(x_1^2+x_2^2=8m^3-8m^2\)
\(\Leftrightarrow4m^2-6m+10=8m^3-8m^2\)
\(\Leftrightarrow8m^3-12m^2+6m-1=9\)
\(\Leftrightarrow\left(2m-1\right)^3=9\)
\(\Leftrightarrow2m-1=\sqrt[3]{9}\)
\(\Rightarrow m=\dfrac{1+\sqrt[3]{9}}{2}\)
a: Δ=(2m-2)^2-4(-m-3)
=4m^2-8m+4+4m+12
=4m^2-4m+16
=4m^2-4m+1+15=(2m-1)^2+15>0
=>Phương trình luôn có 2 nghiệm pb
A=x1^2+x2^2
=(x1+x2)^2-2x1x2
=(2m-2)^2-2(-m-3)
=4m^2-8m+4+2m+6
=4m^2-6m+10
=4(m^2-3/2m+5/2)
=4(m^2-2*m*3/4+9/16+31/16)
=4(m-3/4)^2+31/4>=31/4
Dấu = xảy ra khi m=3/4
b: x1^2+x2^=8m^3-8m^2
=>4m^2-6m+10=8m^3-8m^2
=>8m^3-8m^2-4m^2+6m-10=0
=>8m^3-12m^2+6m-10=0
=>\(m\simeq1,54\)
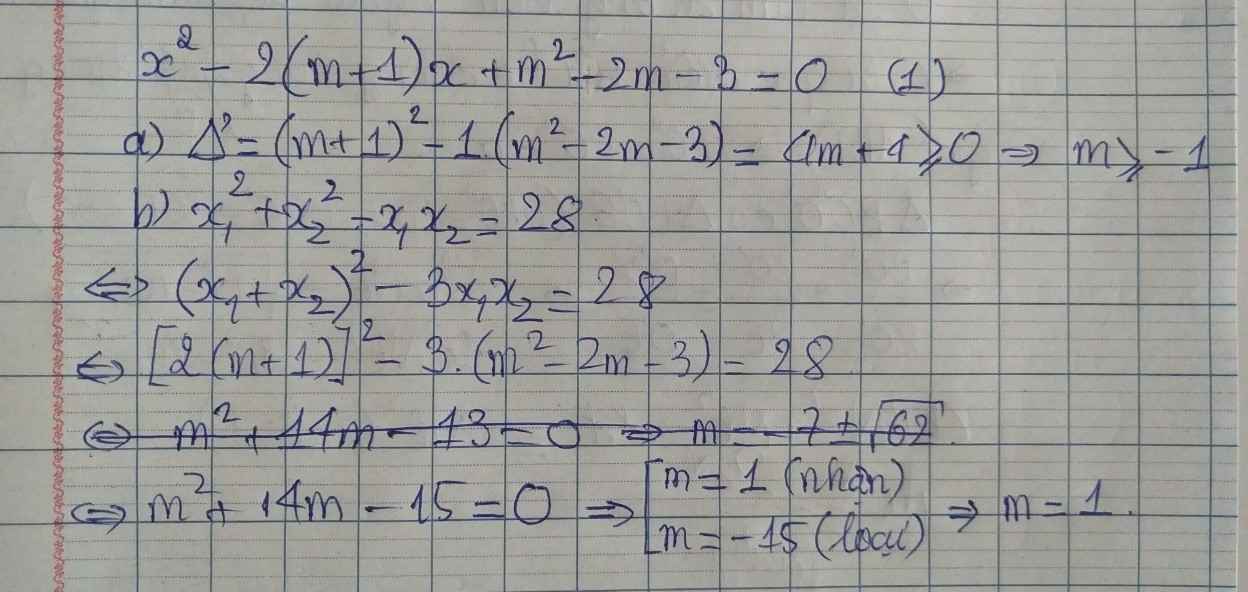
\(x^2-\left(m+2\right)x+m=0\left(1\right)\)
Để phương trình (1) có nghiệm thì:
\(\Delta\ge0\Rightarrow\left(m+2\right)^2-4m\ge0\)
\(\Leftrightarrow m^2+4\ge0\) (luôn đúng)
Vậy \(\forall m\) thì phương trình (1) luôn có nghiệm.
Theo định lí Viete cho phương trình (1) ta có:
\(\left\{{}\begin{matrix}x_1+x_2=m+2\\x_1x_2=m\end{matrix}\right.\)
\(A=x_1^3-\left(m+1\right)x_1^2+mx_1-5m\)
\(=x_1^3-\left(x_1+x_2-1\right)x_1^2+x_1\left(m-5\right)\)
\(=x_1^3-x_1^3-x_1^2x_2+x_1^2+x_1\left(x_1x_2-5\right)\)
\(=-x_1^2x_2+x_1^2+x_1^2x_2-5x_1\)
\(=x_1^2-5x_1=\left(x_1^2-5x_1+\dfrac{25}{4}\right)-\dfrac{25}{4}=\left(x_1-\dfrac{5}{2}\right)^2-\dfrac{25}{4}\ge-\dfrac{25}{4}\)
Vậy \(MinA=-\dfrac{25}{4}\).