Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

(a) Khi \(m=2,\left(1\right)\Leftrightarrow x^2-4x-5=0\left(2\right)\).
Phương trình (2) có \(a-b+c=1-\left(-4\right)+\left(-5\right)=0\)
\(\Rightarrow\left[{}\begin{matrix}x=-1\\x=-\dfrac{c}{a}=5\end{matrix}\right.\).
Vậy: Khi \(m=2,S=\left\{-1;5\right\}\).
(b) Điều kiện: \(x_1,x_2\ne0\Rightarrow m\in R\)
Phương trình có nghiệm khi:
\(\Delta'=\left(-m\right)^2-1\cdot\left(-m^2-1\right)\ge0\)
\(\Leftrightarrow2m^2+1\ge0\left(LĐ\right)\)
Suy ra, phương trình (1) có nghiệm với mọi \(m\).
Theo định lí Vi-ét: \(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2m\\x_1x_2=\dfrac{c}{a}=-m^2-1\end{matrix}\right.\)
Theo đề: \(\dfrac{x_1}{x_2}+\dfrac{x_2}{x_1}=-\dfrac{5}{2}\)
\(\Leftrightarrow\dfrac{x_1^2+x_2^2}{x_1x_2}=-\dfrac{5}{2}\Leftrightarrow\dfrac{\left(x_1+x_2\right)^2-2x_1x_2}{x_1x_2}=-\dfrac{5}{2}\)
\(\Leftrightarrow2\left(x_1+x_2\right)^2+x_1x_2=0\)
\(\Leftrightarrow2\left(2m\right)^2+\left(-m^2-1\right)=0\)
\(\Leftrightarrow7m^2=1\Leftrightarrow m=\pm\dfrac{\sqrt{7}}{7}\) (thỏa mãn).
Vậy: \(m=\pm\dfrac{\sqrt{7}}{7}.\)

a. Khi m=2 thì (1) có dạng :
\(x^2-6\left(2-1\right)x+9\left(2-3\right)=0\\ \Leftrightarrow x^2-6x-9=0\\ \Leftrightarrow\left(x-3\right)^2=18\Leftrightarrow x-3=\pm\sqrt{18}\\ \Leftrightarrow x=3\pm3\sqrt{2}\)
Vậy với m=2 thì tập nghiệm của phương trình là \(S=\left\{3\pm3\sqrt{2}\right\}\)
b. Coi (1) là phương trình bậc 2 ẩn x , ta có:
\(\text{Δ}'=\left(-3m+3\right)^2-1\cdot9\left(m-3\right)=9m^2-18m+9-9m+27\\ =9m^2-27m+36=\left(3m-\dfrac{9}{2}\right)^2+\dfrac{63}{4}>0\)
Nên phương trình (1) luôn có 2 nghiệm x1,x2 thỏa mãn:
\(\left\{{}\begin{matrix}x_1+x_2=6\left(m-1\right)\\x_1x_2=9\left(m-3\right)\end{matrix}\right.\left(2\right)\)
Vì
\(x_1+x_2=2x_1x_2\\ \Leftrightarrow6\left(m-1\right)=18\left(m-3\right)\Leftrightarrow m-1=3m-9\\ \Leftrightarrow2m=8\Leftrightarrow m=4\)
Vậy m=4
b) Ta có: \(\text{Δ}=\left[-6\left(m-1\right)\right]^2-4\cdot1\cdot9\left(m-3\right)\)
\(=\left(6m-6\right)^2-36\left(m-3\right)\)
\(=36m^2-72m+36-36m+108\)
\(=36m^2-108m+144\)
\(=\left(6m\right)^2-2\cdot6m\cdot9+81+63\)
\(=\left(6m-9\right)^2+63>0\forall m\)
Suy ra: Phương trình luôn có hai nghiệm phân biệt với mọi m
Áp dụng hệ thức Vi-et, ta có:
\(\left\{{}\begin{matrix}x_1+x_2=6\left(m-1\right)=6m-6\\x_1\cdot x_2=9\left(m-3\right)=9m-27\end{matrix}\right.\)
Ta có: \(x_1+x_2=2x_1\cdot x_2\)
\(\Leftrightarrow6m-6=2\left(9m-27\right)\)
\(\Leftrightarrow6m-6-18m+54=0\)
\(\Leftrightarrow-12m+48=0\)
\(\Leftrightarrow-12m=-48\)
hay m=4
Vậy: m=4

a, với =-3
\(=>x^2-6x+6=0\)
\(\Delta=\left(-6\right)^2-4.6=12>0\)
=>pt có 2 nghiệm phân biệt x3,x4
\(=>\left[{}\begin{matrix}x3=\dfrac{6+\sqrt{12}}{2}=3+\sqrt{3}\\x4=\dfrac{6-\sqrt{12}}{2}=3-\sqrt{3}\end{matrix}\right.\)
b, \(\Delta=\left(2m\right)^2-4\left(m^2+m\right)=4m^2-4m^2-4m=-4m\)
pt đã cho đề bài có 2 nghiệm phân biệt x1,x2 khi
\(-4m>0< =>m< 0\)
theo vi ét \(=>\left\{{}\begin{matrix}x1+x2=-2m\\x1x2=m^2+m\end{matrix}\right.\)
có \(\left(x1-x2\right)\left(x1^2-x2^2\right)=32\)
\(< =>\left(x1-x2\right)^2\left(x1+x2\right)=32\)
\(< =>\left[x1^2-2x1x2+x2^2\right]\left(-2m\right)=32\)
\(< =>\left[\left(x1+x2\right)^2-4x1x2\right]\left(-2m\right)=32\)
\(< =>\left[\left(-2m\right)^2-4\left(m^2+m\right)\right]\left(-2m\right)=32< =>m=2\)(loại)
Vậy \(m\in\varnothing\)
Lời giải:
a. Với $m=-3$ thì pt trở thành:
$x^2-6x+6=0\Leftrightarrow x=3\pm \sqrt{3}$
b. Để pt có 2 nghiệm thì: $\Delta'=m^2-(m^2+m)=-m\geq 0$
$\Leftrightarrow m\leq 0$
Áp dụng định lý Viet: $x_1+x_2=-2m; x_1x_2=m^2+m$
Khi đó:
$(x_1-x_2)(x_1^2-x_2^2)=32$
$\Leftrightarrow (x_1-x_2)^2(x_1+x_2)=32$
$\Leftrightarrow [(x_1+x_2)^2-4x_1x_2](x_1+x_2)=32$
$\Leftrightarrow [(-2m)^2-4(m^2+m)](-2m)=32$
$\Leftrightarrow 8m^2=32$
$\Leftrightarrow m^2=4$
$\Rightarrow m=-2$ (do $m\leq 0$)
Vây.........

Ta nhận thấy tổng các hệ số của pt bậc 2 đã cho là \(1-a+a-1=0\) nên pt này có 1 nghiệm là 1, nghiệm kia là \(a-1\), nhưng do không được giải pt nên ta sẽ làm theo cách sau:
Ta thấy pt này luôn có 2 nghiệm phân biệt. Theo hệ thức Viète:
\(\left\{{}\begin{matrix}x_1+x_2=a\\x_1x_2=a-1\end{matrix}\right.\)
Vậy, \(M=\dfrac{3\left(x_1^2+x_2^2\right)-3}{x_1x_2\left(x_1+x_2\right)}\)
\(M=\dfrac{3\left[\left(x_1+x_2\right)^2-2x_1x_2\right]-3}{a\left(a-1\right)}\)
\(M=\dfrac{3\left(a^2-2\left(a-1\right)\right)-3}{a\left(a-1\right)}\)
\(M=\dfrac{3\left[\left(a-1\right)^2-1\right]}{a\left(a-1\right)}\)
\(M=\dfrac{3a\left(a+2\right)}{a\left(a-1\right)}\)
\(M=\dfrac{3a+6}{a-1}\)
b) Ta có \(P=\left(x_1+x_2\right)^2-2x_1x_2=a^2-2\left(a-1\right)=\left(a-1\right)^2\ge0\)
Dấu "=" xảy ra \(\Leftrightarrow a=1\). Vậy để P đạt GTNN thì \(a=1\)

a) Điều kiện để phương trình có hai nghiệm trái dấu là :
\(\left\{{}\begin{matrix}m\ne0\\\Delta phẩy>0\\x_1.x_2< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m\ne0\\m^2+4m+4-m^2+3m>0\\\dfrac{m-3}{m}< 0\end{matrix}\right.\)
\(\Rightarrow0< m< 3\)
b) Để phương trình có 2 nghiệm phân biệt thì : \(\Delta\) phẩy > 0
\(\Rightarrow m< 4\)
Ta có : \(\dfrac{1}{x_1^2}+\dfrac{1}{x_2^2}=2\)
\(\Leftrightarrow x_1^2+x_2^2=2x_1^2.x_2^2\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1.x_2=2x_1^2.x_2^2\)
Theo Vi-ét ta có : \(x_1+x_2=\dfrac{-2\left(m-2\right)}{m};x_1.x_2=\dfrac{m-3}{m}\)
\(\Rightarrow\dfrac{4\left(m-2\right)^2}{m^2}-2.\dfrac{m-3}{m}=2.\dfrac{\left(m-3\right)^2}{m^2}\)
\(\Leftrightarrow m=1\left(tm\right)\)
Vậy...........
a) \(mx^2+2\left(m-2\right)x+m-3=0\left(1\right)\)
Để \(\left(1\right)\) có hai nghiệm trái dấu \(\Leftrightarrow\left\{{}\begin{matrix}\Delta'=\left(m-2\right)^2-m\left(m-3\right)>0\\\dfrac{m-3}{m}< 0\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m^2-4m+4-m^2-3m>0\\0< m< 3\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}7m+4>0\\0< m< 3\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}m>-\dfrac{4}{7}\\0< m< 3\end{matrix}\right.\) \(\Leftrightarrow0< m< 3\)
b) \(\dfrac{1}{x^2_1}+\dfrac{1}{x^2_2}=2\Leftrightarrow\dfrac{x^2_1+x_2^2}{x^2_1.x^2_2}=2\) \(\Leftrightarrow\dfrac{\left(x_1+x_2\right)^2-4x_1.x_2}{x^2_1.x^2_2}=2\)
\(\Leftrightarrow\left(\dfrac{x_1+x_2}{x_1.x_2}\right)^2-\dfrac{4}{x_1.x_2}=2\)
\(\Leftrightarrow\left(\dfrac{\dfrac{2\left(2-m\right)}{m}}{\dfrac{m-3}{m}}\right)^2-\dfrac{4}{\dfrac{m-3}{m}}=2\)
\(\Leftrightarrow\left(\dfrac{2\left(2-m\right)}{m-3}\right)^2-\dfrac{4m}{m-3}=2\)
\(\Leftrightarrow4\left(2-m\right)^2-4m\left(m-3\right)=2.\left(m-3\right)^2\)
\(\Leftrightarrow4\left(4-4m+m^2\right)-4m^2+12=2.\left(m^2-6m+9\right)\)
\(\Leftrightarrow16-16m+4m^2-4m^2+12=2m^2-12m+18\)
\(\Leftrightarrow2m^2+4m-10=0\)
\(\Leftrightarrow m^2+2m-5=0\)
\(\Leftrightarrow\left[{}\begin{matrix}m=-1+\sqrt[]{6}\\m=-1-\sqrt[]{6}\end{matrix}\right.\) \(\Leftrightarrow m=-1+\sqrt[]{6}\left(\Delta>0\Rightarrow m>-\dfrac{4}{7}\right)\)

\(x^2+\left(4m+1\right)x+2\left(m-4\right)=0\)
\(\Delta=\left(4m+1\right)^2-4\cdot1\cdot2\left(m-4\right)=16m^2+8m+1-8m+32=16m^2+33\ge33>0\forall m\)
\(\Leftrightarrow\left\{{}\begin{matrix}x_1=\dfrac{-\left(4m+1\right)+\sqrt{16m^2+33}}{2}\\x_2=\dfrac{-\left(4m+1\right)-\sqrt{16m^2+33}}{2}\end{matrix}\right.\)
Mà: \(x_2-x_1=17\)
\(\Leftrightarrow\dfrac{-\left(4m+1\right)-\sqrt{16m^2+33}}{2}-\dfrac{-\left(4m+1\right)+\sqrt{16m^2+33}}{2}=17\)
\(\Leftrightarrow\dfrac{-\left(4m+1\right)-\sqrt{16m^2+33}+\left(4m+1\right)-\sqrt{16m^2+33}}{2}=17\)
\(\Leftrightarrow\dfrac{-2\sqrt{16m^2+33}}{2}=17\)
\(\Leftrightarrow\sqrt{16m^2+33}=-17< 0\)
Vậy không có m thỏa mãn
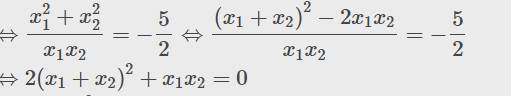
\(\text{Δ}=\left(-2m\right)^2-4\left(m^2-1\right)=4m^2-4m^2+1=1>0\)
Vậy: Phương trình luôn có hai nghiệm phân biệt với mọi m
Theo đề, ta có:
\(\left\{{}\begin{matrix}3x_1-x_2=0\\x_1+x_2=2m\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}4x_1=2m\\x_1+x_2=2m\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x_1=\dfrac{1}{2}m\\x_2=\dfrac{3}{2}m\end{matrix}\right.\)
Ta có: \(x_1\cdot x_2=m^2-1\)
\(\Leftrightarrow m^2\cdot\dfrac{3}{4}-m^2=-1\)
\(\Leftrightarrow m^2=4\)
hay \(m\in\left\{2;-2\right\}\)