
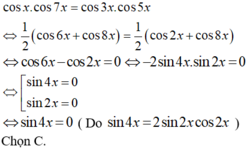
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

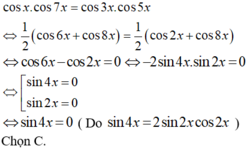

Đáp án B

Nghiệm thứ nhất có 4 họ nghiệm , nhưng có 1 nghiệm trùng với nghiệm thứ 2, như vậy
có tất cả 6 họ nghiệm thỏa mãn đề bài

\(cosx+cos3x+cos2x+cos4x=0\)
\(\Leftrightarrow2cos2x.cosx+2cos3x.cosx=0\)
\(\Leftrightarrow cosx.\left(cos2x+cos3x\right)=0\)
\(\Leftrightarrow cosx.cos\frac{5x}{2}.cos\frac{x}{2}=0\)
\(\Rightarrow\left[{}\begin{matrix}cosx=0\\cos\frac{5x}{2}=0\\cos\frac{x}{2}=0\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{2}+k\pi\\\frac{5x}{2}=\frac{\pi}{2}+k\pi\\\frac{x}{2}=\frac{\pi}{2}+k\pi\end{matrix}\right.\) \(\Rightarrow\left[{}\begin{matrix}x=\frac{\pi}{2}+k\pi\\x=\frac{\pi}{5}+\frac{k2\pi}{5}\\x=\pi+k2\pi\end{matrix}\right.\)
\(sinx+sin7x+sin3x+sin5x=0\)
\(\Leftrightarrow2sin4x.cos3x+2sin4x.cosx=0\)
\(\Leftrightarrow sin4x\left(cos3x+cosx\right)=0\)
\(\Leftrightarrow sin4x.cos2x.cosx=0\)
\(\Leftrightarrow sin4x=0\)
\(\Rightarrow4x=k\pi\Rightarrow x=\frac{k\pi}{4}\)
Lý do chỉ cần 1 pt sin4x=0 do sin4x bao hàm cả cosx và cos2x ở trong đó