Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Do M là trung điểm BC nên: \(\overrightarrow{AM}=\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{AC}\)
Tương tự: \(\overrightarrow{BN}=\dfrac{1}{2}\overrightarrow{BA}+\dfrac{1}{2}\overrightarrow{BC}\) ; \(\overrightarrow{CP}=\dfrac{1}{2}\overrightarrow{CA}+\dfrac{1}{2}\overrightarrow{CB}\)
Cộng vế:
\(\overrightarrow{AM}+\overrightarrow{BN}+\overrightarrow{CP}=\dfrac{1}{2}\overrightarrow{AB}+\dfrac{1}{2}\overrightarrow{AC}+\dfrac{1}{2}\overrightarrow{BA}+\dfrac{1}{2}\overrightarrow{BC}+\dfrac{1}{2}\overrightarrow{CA}+\dfrac{1}{2}\overrightarrow{CB}\)
\(=\dfrac{1}{2}\left(\overrightarrow{AB}+\overrightarrow{BA}\right)+\dfrac{1}{2}\left(\overrightarrow{AC}+\overrightarrow{CA}\right)+\dfrac{1}{2}\left(\overrightarrow{BC}+\overrightarrow{CB}\right)=\overrightarrow{0}\)
b. Từ câu a ta có:
\(\overrightarrow{AM}+\overrightarrow{BN}+\overrightarrow{CP}=\overrightarrow{0}\)
\(\Leftrightarrow\overrightarrow{AO}+\overrightarrow{OM}+\overrightarrow{BO}+\overrightarrow{ON}+\overrightarrow{CO}+\overrightarrow{OP}=\overrightarrow{0}\)
\(\Leftrightarrow-\overrightarrow{OA}+\overrightarrow{OM}-\overrightarrow{OB}+\overrightarrow{ON}-\overrightarrow{OC}+\overrightarrow{OP}=\overrightarrow{0}\)
\(\Leftrightarrow\overrightarrow{OA}+\overrightarrow{OB}+\overrightarrow{OC}=\overrightarrow{OM}+\overrightarrow{ON}+\overrightarrow{OP}\) (đpcm)

Vì ABCD là hình chữ nhật
nên \(\overrightarrow{AB}+\overrightarrow{AD}=\overrightarrow{AC};\overrightarrow{BA}+\overrightarrow{BC}=\overrightarrow{BD}\)
=>\(\left|\overrightarrow{AB}+\overrightarrow{AD}\right|=\left|\overrightarrow{AC}\right|=AC;\left|\overrightarrow{BA}+\overrightarrow{BC}\right|=\left|\overrightarrow{BD}\right|=BD\)
mà AC=BD(ABCD là hình chữ nhật)
nên \(\left|\overrightarrow{AB}+\overrightarrow{AD}\right|=\left|\overrightarrow{BA}+\overrightarrow{BC}\right|\)

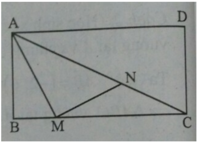
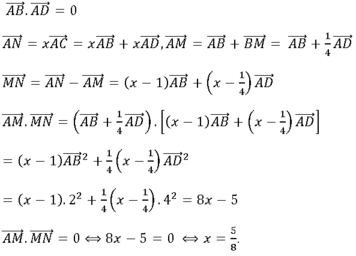
Chọn A.
Chú ý: Nếu có đúng bốn phương án như trong đề thi thì có thể dự đoán ngay phương án A sau khi vẽ hình
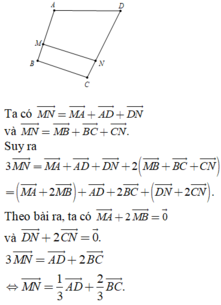

\(\overrightarrow{AC}+\overrightarrow{BM}=\left(\overrightarrow{AM}+\overrightarrow{MC}\right)+\left(\overrightarrow{BC}+\overrightarrow{CM}\right)=\overrightarrow{AM}+\overrightarrow{BC}+\left(\overrightarrow{MC}+\overrightarrow{CM}\right)=\overrightarrow{AM}+\overrightarrow{BC}\)