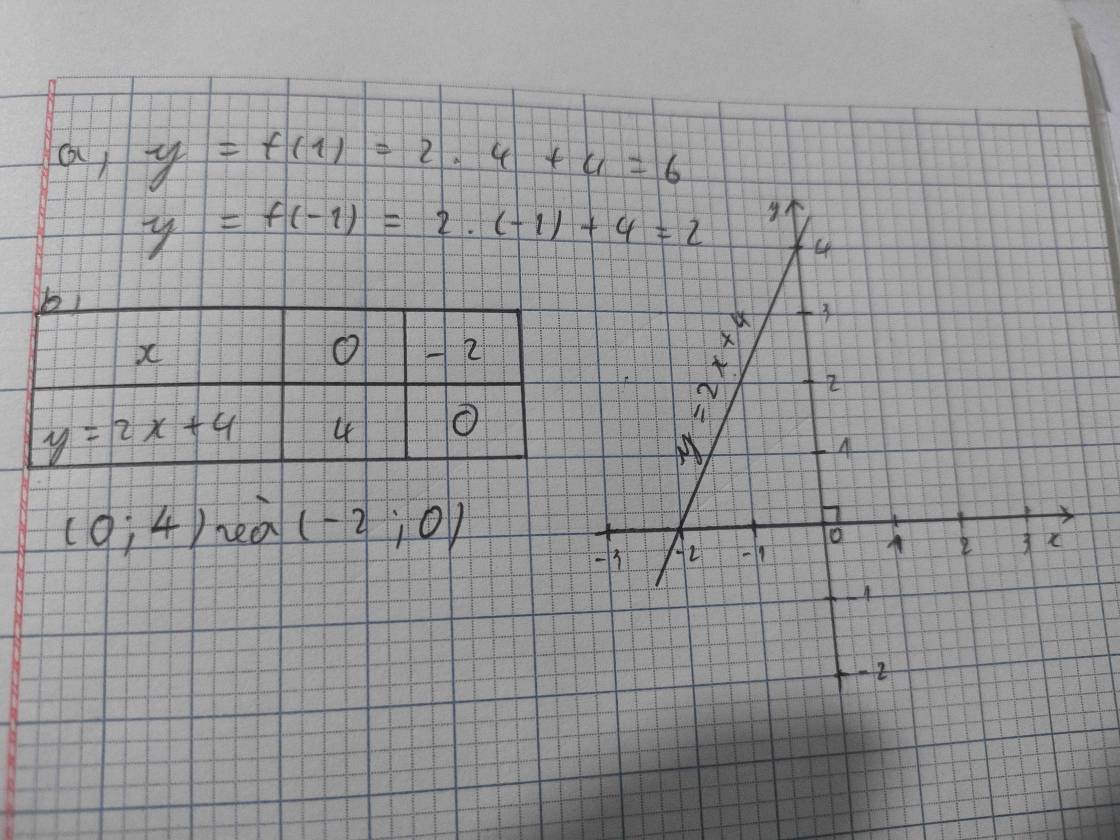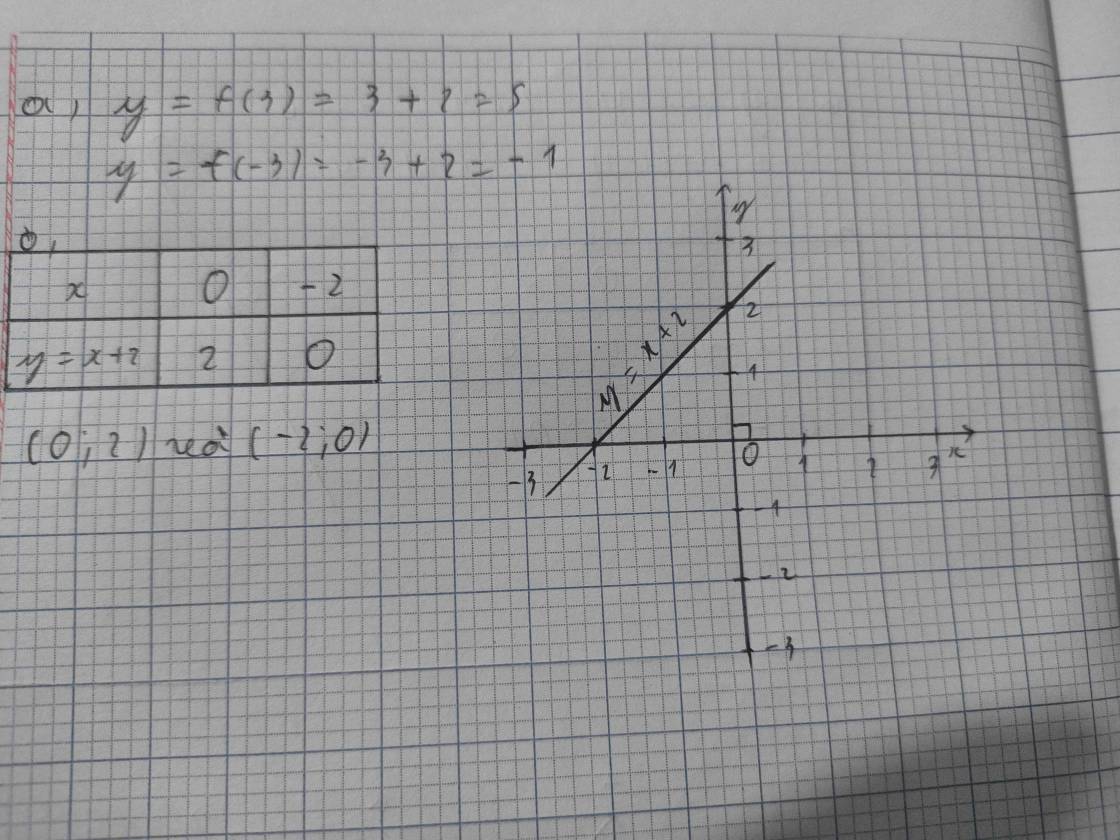NT
0
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
23 tháng 12 2023
Câu 5:
a: Khi m=3 thì \(f\left(x\right)=\left(2\cdot3+1\right)x-3=7x-3\)
\(f\left(-3\right)=7\cdot\left(-3\right)-3=-21-3=-24\)
\(f\left(0\right)=7\cdot0-3=-3\)
b: Thay x=2 và y=3 vào f(x)=(2m+1)x-3, ta được:
\(2\left(2m+1\right)-3=3\)
=>2(2m+1)=6
=>2m+1=3
=>2m=2
=>m=1
c: Thay m=1 vào hàm số, ta được:
\(y=\left(2\cdot1+1\right)x-3=3x-3\)
*Vẽ đồ thị
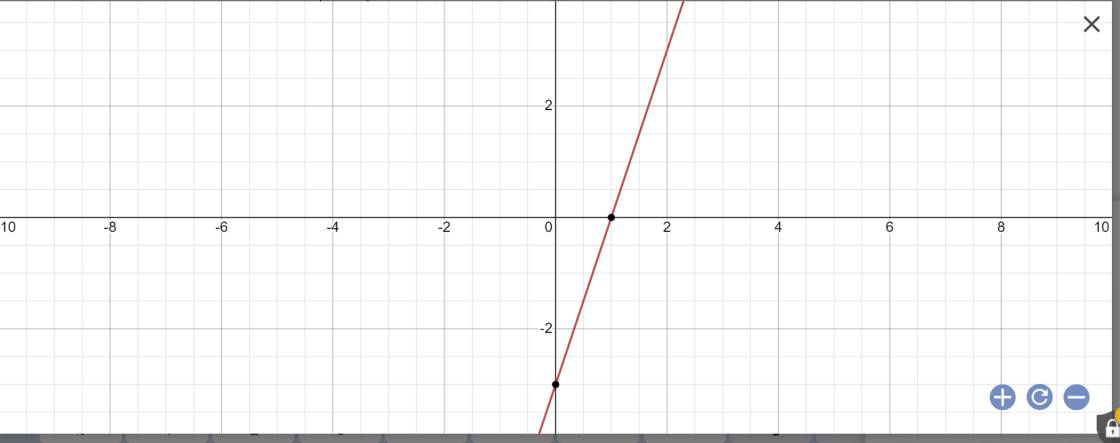
d: Để hàm số y=(2m+1)x-3 là hàm số bậc nhất thì \(2m+1\ne0\)
=>\(2m\ne-1\)
=>\(m\ne-\dfrac{1}{2}\)
e: Để đồ thị hàm số y=(2m+1)x-3 song song với đường thẳng y=5x+1 thì \(\left\{{}\begin{matrix}2m+1=5\\-3\ne1\end{matrix}\right.\)
=>2m+1=5
=>2m=4
=>m=2
19 tháng 1
a: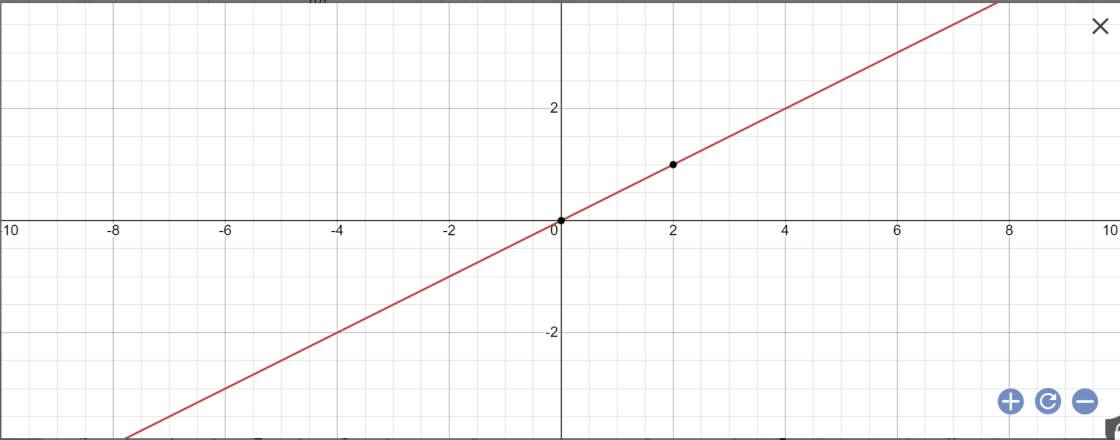 
b: \(f\left(2\right)=\dfrac{1}{2}\cdot2=1\)
\(f\left(1\right)=\dfrac{1}{2}\cdot1=\dfrac{1}{2}\)
\(f\left(-2\right)=\dfrac{1}{2}\cdot\left(-2\right)=-1\)
\(f\left(-1\right)=\dfrac{1}{2}\cdot\left(-1\right)=-\dfrac{1}{2}\)
\(f\left(0\right)=\dfrac{1}{2}\cdot0=0\)
c: f(x)=2
=>\(\dfrac{1}{2}x=2\)
=>x=2*2=4
f(x)=1
=>\(\dfrac{1}{2}x=1\)
=>\(x=1:\dfrac{1}{2}=2\)
f(x)=-1
=>\(\dfrac{1}{2}x=-1\)
=>\(x=-1\cdot2=-2\)
d: \(f\left(-1\right)=\dfrac{1}{2}\cdot\left(-1\right)=-\dfrac{1}{2}\ne\dfrac{1}{2}=y_A\)
=>A(-1;1/2) không thuộc đồ thị hàm số y=1/2x
\(f\left(-1\right)=\dfrac{1}{2}\cdot\left(-1\right)=-\dfrac{1}{2}=y_B\)
=>\(B\left(-1;-\dfrac{1}{2}\right)\) thuộc đồ thị hàm số y=1/2x
19 tháng 1
a: \(f\left(0\right)=3\cdot0=0\)
\(f\left(1\right)=3\cdot1=3\)
b:
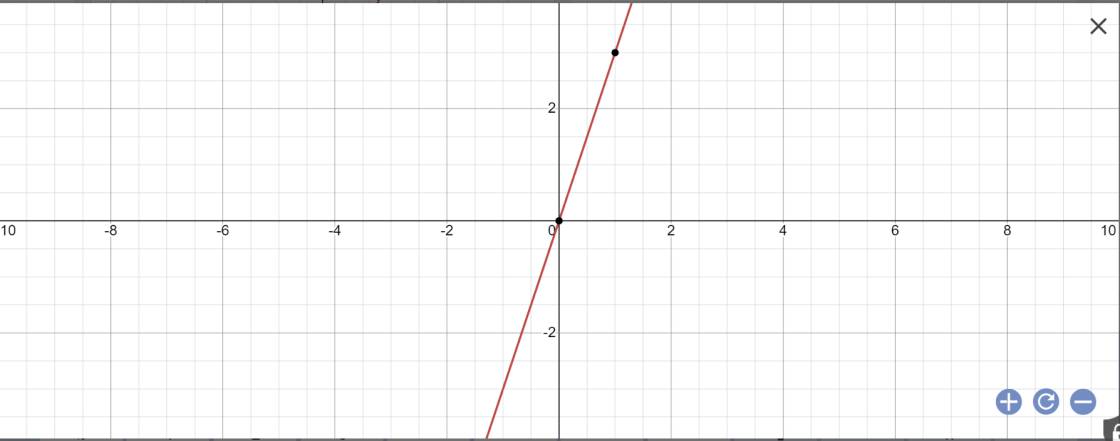
c: \(f\left(-2\right)=3\cdot\left(-2\right)=-6=y_A\)
=>A(-2;-6) thuộc đồ thị hàm số y=3x
\(f\left(2\right)=3\cdot2=6\ne-6=y_B\)
=>B(2;-6) không thuộc đồ thị hàm số y=3x
2 tháng 12 2023
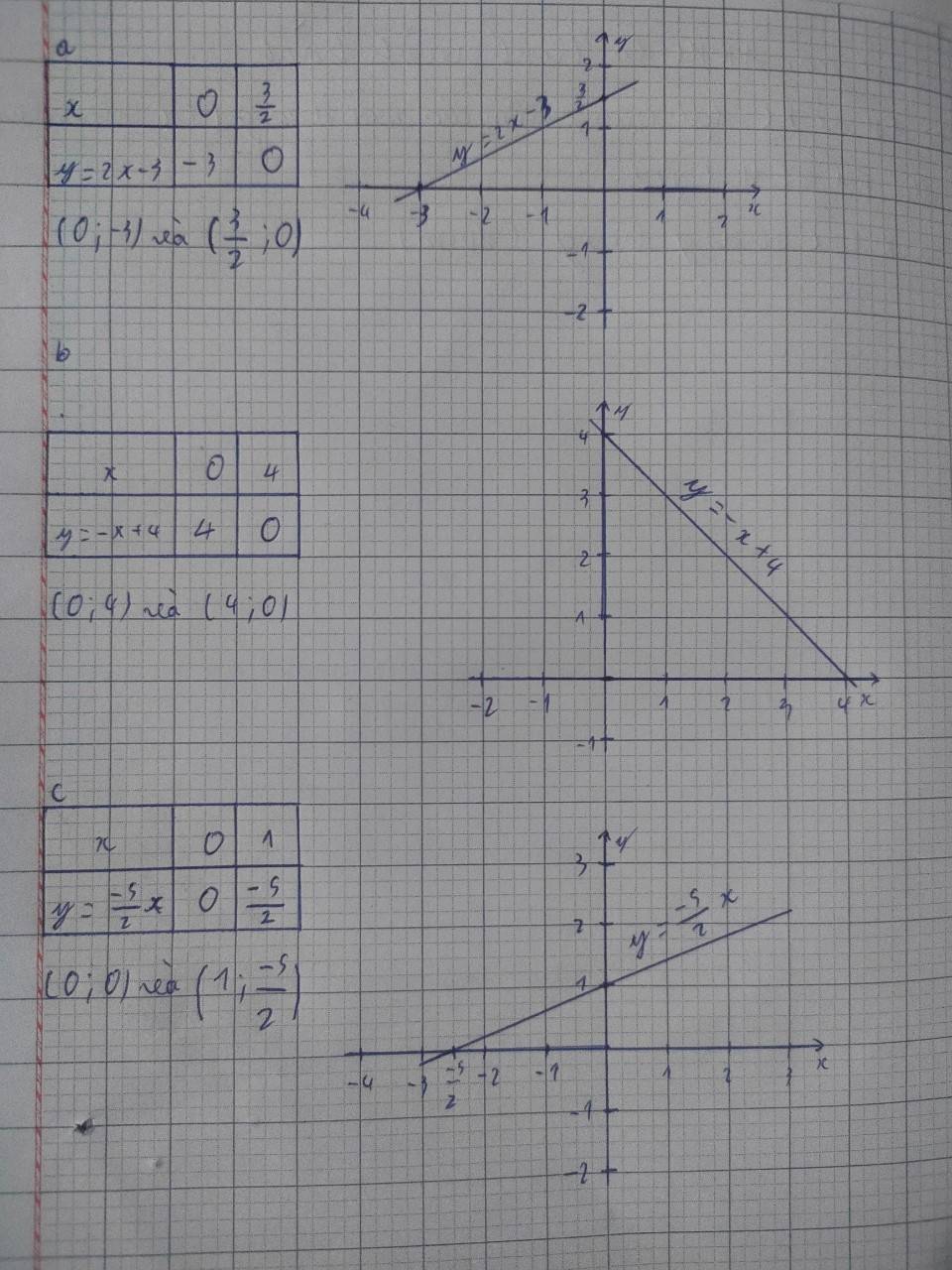
a:Đặt (d1): y=2x-3
Tọa độ giao điểm của (d1) với trục Ox là:
\(\left\{{}\begin{matrix}y=0\\2x-3=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=0\\2x=3\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=\dfrac{3}{2}\\y=0\end{matrix}\right.\)
Tọa độ giao điểm của (d1) với trục Oy là:
\(\left\{{}\begin{matrix}x=0\\y=2x-3\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=0\\y=2\cdot0-3=0-3=-3\end{matrix}\right.\)
b: Đặt (d2): \(y=-\dfrac{3}{4}x\)
Tọa độ giao điểm của (d2) với trục Ox là:
\(\left\{{}\begin{matrix}y=0\\-\dfrac{3}{4}x=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=0\\y=0\end{matrix}\right.\)
Tọa độ giao điểm của (d2) với trục Oy là:
\(\left\{{}\begin{matrix}x=0\\y=-\dfrac{3}{4}x=-\dfrac{3}{4}\cdot0=0\end{matrix}\right.\)
c: Đặt \(\left(d3\right):y=2x^2\)
Tọa độ giao điểm của (d3) với trục Ox là:
\(\left\{{}\begin{matrix}2x^2=0\\y=2x^2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x^2=0\\y=2x^2\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=0\\y=2\cdot0^2=0\end{matrix}\right.\)
Tọa độ giao điểm của (d3) với trục Oy là:
\(\left\{{}\begin{matrix}x=0\\y=2x^2\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=0\\y=2\cdot0^2=0\end{matrix}\right.\)
d: Đặt (d4): \(y=\dfrac{x+1}{x-2}\)
ĐKXĐ: x<>2
Tọa độ giao điểm của (d4) với trục Ox là:
\(\left\{{}\begin{matrix}y=0\\y=\dfrac{x+1}{x-2}\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=0\\x+1=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x=-1\\y=0\end{matrix}\right.\)
Tọa độ giao điểm của (d4) với trục Oy là:
\(\left\{{}\begin{matrix}x=0\\y=\dfrac{x+1}{x-2}=\dfrac{0+1}{0-2}=\dfrac{1}{-2}=-\dfrac{1}{2}\end{matrix}\right.\)
e: Đặt (d5): \(y=x-2+\dfrac{1}{x}\)
ĐKXĐ: x<>0
Vì hàm số không đi qua điểm có hoành độ là x=0 nên (d5) sẽ không cắt trục Oy
Tọa độ giao điểm của (d5) với trục Ox là:
\(\left\{{}\begin{matrix}y=0\\x-2+\dfrac{1}{x}=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x^2-2x+1=0\\y=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}\left(x-1\right)^2=0\\y=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x-1=0\\y=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=1\\y=0\end{matrix}\right.\)
f: Đặt (d6): \(y=x^2+2x-5\)
Tọa độ giao điểm của (d6) với trục Oy là:
\(\left\{{}\begin{matrix}x=0\\y=x^2+2x-5=0^2+2\cdot0-5=-5\end{matrix}\right.\)
Tọa độ giao điểm của (d6) với trục Ox là:
\(\left\{{}\begin{matrix}y=0\\x^2+2x-5=0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}y=0\\x^2+2x+1-6=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=0\\\left(x+1\right)^2=6\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}\left\{{}\begin{matrix}y=0\\x+1=\sqrt{6}\end{matrix}\right.\\\left\{{}\begin{matrix}y=0\\x+1=-\sqrt{6}\end{matrix}\right.\end{matrix}\right.\)
=>\(\left[{}\begin{matrix}\left\{{}\begin{matrix}y=0\\x=\sqrt{6}-1\end{matrix}\right.\\\left\{{}\begin{matrix}y=0\\x=-\sqrt{6}-1\end{matrix}\right.\end{matrix}\right.\)
26 tháng 1
a: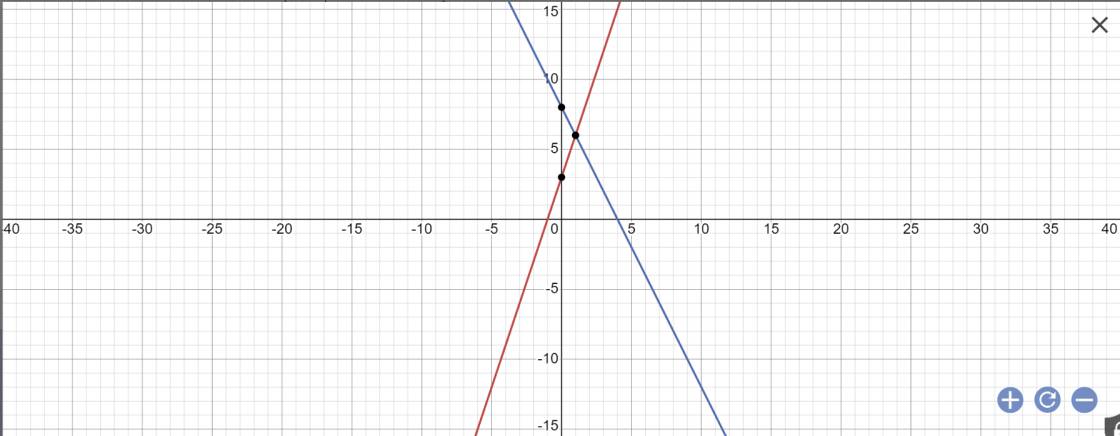 
b: Tọa độ A là:
\(\left\{{}\begin{matrix}3x+3=-2x+8\\y=-2x+8\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}5x=5\\y=-2x+8\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=1\\y=-2+8=6\end{matrix}\right.\)
Vậy: A(1;6)
Tọa độ B là:
\(\left\{{}\begin{matrix}y=0\\3x+3=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x+1=0\\y=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=-1\\y=0\end{matrix}\right.\)
Tọa độ C là:
\(\left\{{}\begin{matrix}y=0\\-2x+8=0\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}y=0\\-2x=-8\end{matrix}\right.\)
=>\(\left\{{}\begin{matrix}x=4\\y=0\end{matrix}\right.\)
Vậy: A(1;6); B(-1;0); C(4;0)
\(AB=\sqrt{\left(-1-1\right)^2+\left(0-6\right)^2}=2\sqrt{10}\)
\(AC=\sqrt{\left(4-1\right)^2+\left(0-6\right)^2}=3\sqrt{5}\)
\(BC=\sqrt{\left(4+1\right)^2+\left(0-0\right)^2}=5\)
Xét ΔABC có \(cosBAC=\dfrac{AB^2+AC^2-BC^2}{2\cdot BA\cdot AC}=\dfrac{40+45-25}{2\cdot2\sqrt{10}\cdot3\sqrt{5}}=\dfrac{\sqrt{2}}{2}\)
=>\(sinBAC=\sqrt{1-\left(\dfrac{\sqrt{2}}{2}\right)^2}=\dfrac{\sqrt{2}}{2}\)
\(S_{ABC}=\dfrac{1}{2}\cdot AB\cdot AC\cdot sinBAC\)
\(=\dfrac{1}{2}\cdot\dfrac{\sqrt{2}}{2}\cdot2\sqrt{10}\cdot3\sqrt{5}=15\)