Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

Từ giả thiết, ta có:
\(\dfrac{1}{1+a}\ge1-\dfrac{1}{1+b}+1-\dfrac{1}{1+c}+1-\dfrac{1}{1+d}=\dfrac{b}{1+b}+\dfrac{c}{c+1}+\dfrac{d}{d+1}\ge3\sqrt[3]{\dfrac{b.c.d}{\left(1+b\right)\left(1+c\right)\left(1+d\right)}}\)
Tương tự:
\(\dfrac{1}{1+b}\ge3\sqrt[3]{\dfrac{cda}{\left(1+a\right)\left(1+c\right)\left(1+d\right)}}\)
\(\dfrac{1}{1+c}\ge3\sqrt[3]{\dfrac{abd}{\left(1+a\right)\left(1+b\right)\left(1+d\right)}}\)
\(\dfrac{1}{1+d}\ge3\sqrt[3]{\dfrac{abc}{\left(1+a\right)\left(1+b\right)\left(1+c\right)}}\)
Nhân vế theo vế 4 BĐT vừa chứng minh rồi rút gọn ta được:
\(abcd\le\dfrac{1}{81}\left(đpcm\right)\)

Sửa \(\le\) thành \(\ge\) nha bạn
\(\dfrac{1}{a}+\dfrac{1}{b}+\dfrac{1}{c}=1\Leftrightarrow ab+bc+ca=abc\)
Ta có \(\dfrac{a^2}{a+bc}=\dfrac{a^3}{a^2+abc}=\dfrac{a^3}{a^2+ab+bc+ca}=\dfrac{a^3}{\left(a+b\right)\left(a+c\right)}\)
Tương tự: \(\left\{{}\begin{matrix}\dfrac{b^2}{b+ca}=\dfrac{b^3}{\left(b+a\right)\left(b+c\right)}\\\dfrac{c^2}{c+ba}=\dfrac{c^3}{\left(c+b\right)\left(c+a\right)}\end{matrix}\right.\)
Áp dụng BĐT cosi:
\(\dfrac{a^3}{\left(a+b\right)\left(a+c\right)}+\dfrac{a+b}{8}+\dfrac{a+c}{8}\ge3\sqrt[3]{\dfrac{a^3}{64}}=\dfrac{3}{4}a\)
\(\dfrac{b^3}{\left(b+a\right)\left(b+c\right)}+\dfrac{a+b}{8}+\dfrac{b+c}{8}\ge3\sqrt[3]{\dfrac{b^3}{64}}=\dfrac{3}{4}b\)
\(\dfrac{c^3}{\left(c+b\right)\left(c+a\right)}+\dfrac{b+c}{8}+\dfrac{a+c}{8}\ge3\sqrt[3]{\dfrac{c^3}{64}}=\dfrac{3}{4}c\)
Cộng VTV:
\(\Leftrightarrow VT+\dfrac{a+b}{8}+\dfrac{a+c}{8}+\dfrac{b+c}{8}\ge\dfrac{3}{4}\left(a+b+c\right)\\ \Leftrightarrow VT\ge\dfrac{3\left(a+b+c\right)}{4}-\dfrac{2\left(a+b+c\right)}{8}\\ \Leftrightarrow VT\ge\dfrac{a+b+c}{4}\)
Dấu \("="\Leftrightarrow a=b=c=3\)

Bạn tham khảo:
Bài ni hay lắm mn Cho 3 số a , b , c thỏa mãn \(0\le a\le b\le c\le1\) Tìm giá trị lớn nhất của biểu thức \(B=\lef... - Hoc24
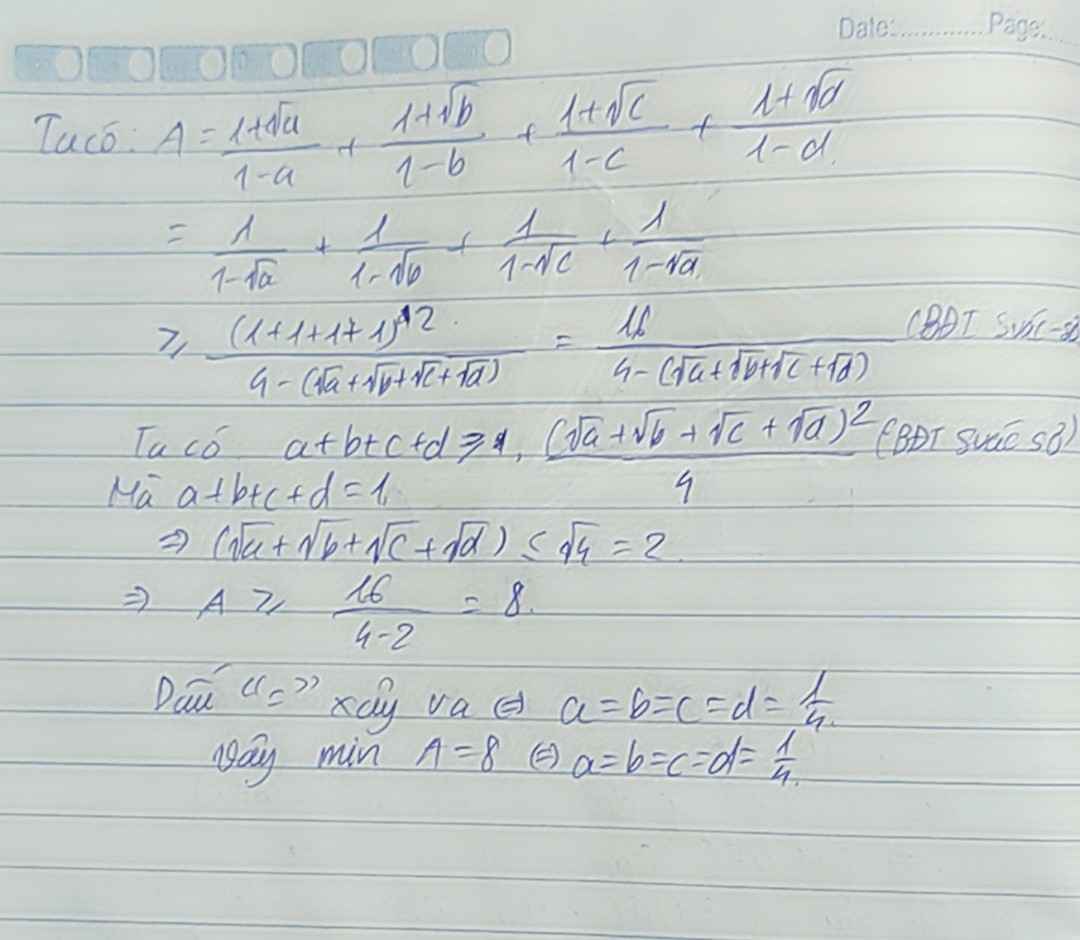
\(\dfrac{b}{1+b}+\dfrac{c}{1+c}+\dfrac{d}{1+d}\le1-\dfrac{a}{1+a}=\dfrac{1}{1+a}\)
\(\Rightarrow\dfrac{1}{1+a}\ge\dfrac{b}{1+b}+\dfrac{c}{1+c}+\dfrac{d}{1+d}\ge3\dfrac{\sqrt[3]{bcd}}{\sqrt[3]{\left(1+b\right)\left(1+c\right)\left(1+d\right)}}\)
Chứng minh tương tự ta có:
\(\dfrac{1}{1+b}\ge3\dfrac{\sqrt[3]{acd}}{\sqrt[3]{\left(1+a\right)\left(1+c\right)\left(1+d\right)}}\)
\(\dfrac{1}{1+c}\ge3\dfrac{\sqrt[3]{abd}}{\sqrt[3]{\left(1+a\right)\left(1+b\right)\left(1+d\right)}}\)
\(\dfrac{1}{1+d}\ge3\dfrac{\sqrt[3]{abc}}{\sqrt[3]{\left(1+a\right)\left(1+b\right)\left(1+c\right)}}\)
Nhân vế với vế của các BĐT trên ta được:
\(\dfrac{1}{\left(1+a\right)\left(1+b\right)\left(1+c\right)\left(1+d\right)}\ge81\dfrac{abcd}{\left(1+a\right)\left(1+b\right)\left(1+c\right)\left(1+d\right)}\)
\(\Rightarrow81abcd\le1\Rightarrow abcd\le\dfrac{1}{81}\)
Dấu "=" xảy ra khi \(a=b=c=d=\dfrac{1}{3}\)