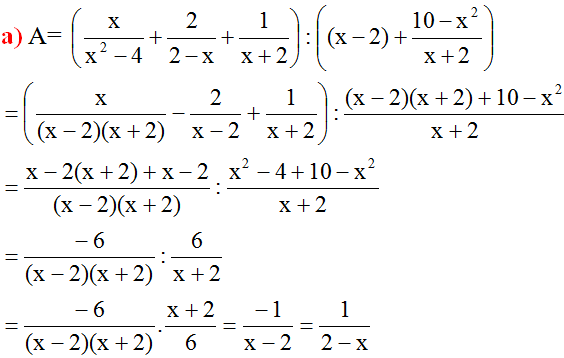Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

ĐKXĐ : \(x\ne\left\{1;0\right\}\)
a) \(P=\left(\dfrac{\left(x-1\right)^2}{3x+\left(x-1\right)^2}-\dfrac{1-2x^2+4x}{x^3-1}+\dfrac{1}{x-1}\right):\dfrac{2x}{x^3+x}\)
\(P=\left(\dfrac{\left(x-1\right)^2}{x^2+x+1}-\dfrac{1-2x^2+4x}{\left(x-1\right)\left(x^2+x+1\right)}+\dfrac{1}{x-1}\right)\cdot\dfrac{x\left(x^2+1\right)}{2x}\)
\(P=\left(\dfrac{\left(x-1\right)\left(x-1\right)^2}{\left(x-1\right)\left(x^2+x+1\right)}-\dfrac{1-2x^2+4x}{\left(x-1\right)\left(x^2+x+1\right)}+\dfrac{x^2+x+1}{\left(x-1\right)\left(x^2+x+1\right)}\right)\cdot\dfrac{x^2+1}{2}\)
\(P=\left(\dfrac{\left(x-1\right)^3-1+2x^2-4x+x^2+x+1}{\left(x-1\right)\left(x^2+x+1\right)}\right)\cdot\dfrac{x^2+1}{2}\)
\(P=\left(\dfrac{x^3-3x^2+3x-1-1+2x^2-4x+x^2+x+1}{\left(x-1\right)\left(x^2+x+1\right)}\right)\cdot\dfrac{x^2+1}{2}\)
\(P=\left(\dfrac{x^3-1}{x^3-1}\right)\cdot\dfrac{x^2+1}{2}\)
\(P=1\cdot\dfrac{x^2+1}{2}\)
\(P=\dfrac{x^2+1}{2}\)
b) Vì \(x^2\ge0\forall x\)
\(\Rightarrow P\ge\dfrac{1}{2}\)
Dấu "=" xảy ra \(\Leftrightarrow x=0\)
Mà ĐKXĐ \(x\ne0\)
=> ... đến đây ko biết làm :v ![]()
AI BIẾT LÀM HỘ ĐI
Cái này mk chưa học nên cx chưa rõ cách làm chính xác mong bạn thông cảm :)

a.
ĐKXĐ: \(x\ne2\)
b.
\(P=\left(\dfrac{2x}{x-2}+\dfrac{x}{2-x}\right):\dfrac{x^2+1}{x-2}\)
\(=\left(\dfrac{2x}{x-2}-\dfrac{x}{x-2}\right)\cdot\dfrac{x-2}{x^2+1}\)
\(=\dfrac{x}{x-2}\cdot\dfrac{x-2}{x^2+1}=\dfrac{x}{x^2+1}\)
c.
\(x=-1\Rightarrow P=-\dfrac{1}{\left(-1\right)^2+1}=-\dfrac{1}{2}\)
d.
\(P=\dfrac{x}{x^2+1}\cdot\dfrac{x^2+1}{x}-\dfrac{1}{P}\ge1-\dfrac{1}{P}\)
\(\Rightarrow\dfrac{P^2+1}{P}\ge1\)
\(\Rightarrow P^2+1\ge P\) \(\Rightarrow P\left(P-1\right)\ge1\)
\(\Rightarrow P\ge2\)
Dấu "=" khi x = ...................
Bài 2:
a: \(M=\dfrac{3x+1-2x-2}{\left(3x-1\right)\left(3x+1\right)}:\dfrac{3x+1-3x}{x\left(3x+1\right)}\)
\(=\dfrac{x-1}{\left(3x-1\right)\left(3x+1\right)}\cdot\dfrac{x\left(3x+1\right)}{1}=\dfrac{x\left(x-1\right)}{3x-1}\)
b: Để M=0 thì x(x-1)=0
=>x=1(nhận) hoặc x=0(loại)
c: \(P=M\cdot\left(3x-1\right)=x\left(x-1\right)=x^2-x+\dfrac{1}{4}-\dfrac{1}{4}=\left(x-\dfrac{1}{2}\right)^2-\dfrac{1}{4}>=-\dfrac{1}{4}\)
Dấu = xảy ra khi x=1/2

a: \(M=\left[\dfrac{x^2-2x+1}{x^2+x+1}+\dfrac{2x^2-4x-1}{\left(x-1\right)\left(x^2+x+1\right)}+\dfrac{1}{x-1}\right]\cdot\dfrac{x^2+1}{2}\)
\(=\dfrac{x^3-3x^2+3x-1+2x^2-4x-1+x^2+x+1}{\left(x-1\right)\left(x^2+x+1\right)}\cdot\dfrac{x^2+1}{2}\)
\(=\dfrac{x^2+1}{2}\)

\(A=\frac{x}{x+1}-\frac{3-3x}{x^2-x+1}+\frac{x+4}{x^3+1}\)
\(A=\frac{x\left(x^2-x+1\right)}{\left(x+1\right)\left(x^2-x+1\right)}-\frac{3-3x}{\left(x+1\right)\left(x^2-x+1\right)}+\frac{x+4}{\left(x+1\right)\left(x^2-x+1\right)}\)
\(A=\frac{x^3-x^2+x-3-3x+x+4}{\left(x+1\right)\left(x^2-x+1\right)}\)
\(A=\frac{1}{\left(x+1\right)\left(x^2-x+1\right)}=\frac{1}{x^3+1}\)

Câu 1:
a: ĐKXĐ: \(x\notin\left\{0;1;\dfrac{1}{2}\right\}\)
\(B=\dfrac{x^2+x}{x^2+x+1}-\dfrac{2x^3+x^2-x-2x^3+2-x^2-x-1}{\left(x-1\right)\left(x^2+x+1\right)}\cdot\dfrac{-x\left(x-1\right)}{2x-1}\)
\(=\dfrac{x\left(x+1\right)}{x^2+x+1}-\dfrac{-2x+1}{\left(x-1\right)\left(x^2+x+1\right)}\cdot\dfrac{-x\left(x-1\right)}{2x-1}\)
\(=\dfrac{x\left(x+1\right)}{x^2+x+1}+\dfrac{2x-1}{\left(x-1\right)\left(x^2+x+1\right)}\cdot\dfrac{-x\left(x-1\right)}{2x-1}\)
\(=\dfrac{x\left(x+1\right)}{x^2+x+1}+\dfrac{-x}{x^2+x+1}=\dfrac{x^2}{x^2+x+1}\)
b: Để \(B=\dfrac{4}{3}\) thì \(\dfrac{x^2}{x^2+x+1}=\dfrac{4}{3}\)
\(\Leftrightarrow4x^2+4x+4-3x^2=0\)
=>x=-2(nhận)

Lời giải của bạn Nhật Linh đúng rồi, tuy nhiên cần thêm điều kiện để A có nghĩa: \(x\ne\pm2\)

a: \(P=\left(\dfrac{-\left(x+2\right)}{x-2}-\dfrac{4x^2}{\left(x-2\right)\left(x+2\right)}+\dfrac{x-2}{x+2}\right)\cdot\dfrac{x^2\left(2-x\right)}{x\left(x-3\right)}\)
\(=\dfrac{-x^2-4x-4-4x^2+x^2-4x+4}{\left(x-2\right)\left(x+2\right)}\cdot\dfrac{-x\left(x-2\right)}{x-3}\)
\(=\dfrac{-4x^2-8x}{\left(x+2\right)}\cdot\dfrac{-x}{x-3}=\dfrac{4x^2}{x-3}\)
c: \(P=\dfrac{4x^2-12x+12x-36+36}{x-3}\)
\(=4x+12+\dfrac{36}{x-3}=4x-12+\dfrac{36}{x-3}+24\)
\(\Leftrightarrow P>=2\sqrt{4\left(x-3\right)\cdot\dfrac{36}{x-3}}+24=2\cdot\sqrt{144}+24=2\cdot12+24=48\)
Dấu = xảy ra khi 4(x-3)^2=36
=>(x-3)^2=9
=>x=6

\(\Leftrightarrow A=\left(\dfrac{x^2+x}{x^2-2x+1}\right):\left(\dfrac{\left(x+1\right)\left(x-1\right)+x-\left(x^2-2\right)}{x\left(x-1\right)}\right)\\ \)
\(\Leftrightarrow A=\left(\dfrac{x\left(x+1\right)}{\left(x-1\right)^2}\right).\left(\dfrac{x\left(x-1\right)}{x+1}\right)\)
\(\Leftrightarrow\left\{{}\begin{matrix}x\ne\pm1\\A=\dfrac{x^2}{\left(x-1\right)}\end{matrix}\right.\)
a) \(A>2\Leftrightarrow\left\{{}\begin{matrix}x\ne\pm1\\\dfrac{x^2-2x+2}{x-1}>0\end{matrix}\right.\) \(\Leftrightarrow x>1\)
b) \(A=\left(x-1\right)+\dfrac{1}{x-1}+2\)
\(x>1\Leftrightarrow A=\left(\sqrt{x-1}-\dfrac{1}{\sqrt{x-1}}\right)^2+4\ge4\) dang thuc x=2