
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a) Từ a + 5 < b + 5
=> a + 5 + (-5) < b + 5 + (-5) (cộng hai vế với -5)
=> a < b
a)từ a+5<b+5 ta cộng -5 vào 2 vế được a<b
b)từ -3a>-3b ta nhân 2 vế với -1/3 (tức là chia cả 2 vế cho -3) và -3a . -1/3< -3b . -1/3 sẽ được a<b

a)Do bd>0 (do b>0, d>0) nên nếu \(\frac{a}{b}< \frac{c}{d}\) thì ad<bc
b)Ngược lại, nếu ad<bc thì \(\frac{ad}{bd}< \frac{bc}{bd}\Leftrightarrow\frac{a}{b}< \frac{c}{d}\)

a. Do \(a>0,\) \(b>0\) \(\Rightarrow a,b\) là số dương
Ta có:
* \(a< b\Leftrightarrow a^2< ab\) (nhân cả hai vế với a)
* \(a< b\Leftrightarrow ab< b^2\) (nhân cả hai vế với b)
b. Từ câu a theo tính chất bắc cầu suy ra:\(a^2< b^2\)
Ta có: \(a^2< b^2\Leftrightarrow a^3< ab^2\) (nhân cả hai vế với a)
mà ab2<b3 (a<b)
\(\Rightarrow a^3< b^3\)

Thân heo vừa béo lại vừa ù
Bảy nổi ba chìm với nước lu
Chết đuối quẫy chân không ai cứu
Đứa nào mà cứu, đứa ấy ngu
a, a2+b2+c2 >= ab+bc+ca
<=>a2+b2+c2-ab-bc-ca >= 0
<=>2(a2+b2+c2-ab-bc-ca) >= 0
<=>2a2+2b2+2c2-2ab-2bc-2ca >= 0
<=>(a2-2ab+b2)+(b2-2bc+c2)+(c2-2ca+a2) >= 0
<=>(a-b)2+(b-c)2+(c-a)2 >= 0 (luôn đúng)
Dấu "=" xảy ra chỉ khi và khi \(\hept{\begin{cases}a-b=0\\b-c=0\\c-a=0\end{cases}\Leftrightarrow a=b=c}\)
Vậy...
b, a2+b2+1 >= ab+a+b
<=>a2+b2+1-ab-a-b >= 0
<=>2(a2+b2+1-ab-a-b) >= 0
<=>2a2+2b2+2-2ab-2a-2b >= 0
<=>(a2-2ab+b2)+(a2-2a+1)+(b2-2b+1) >= 0
<=>(a-b)2+(a-1)2+(b-1)2 >= 0 (luôn đúng)
Dấu "=" xảy ra chỉ khi và khi \(\hept{\begin{cases}a-b=0\\a-1=0\\b-1=0\end{cases}\Leftrightarrow a=b=1}\)
Vậy...
c, a2+b2+c2+3 >= 2(a+b+c)
<=>a2+b2+c2+3-2a-2b-2c >= 0
<=>(a2-2a+1)+(b2-2b+1)+(c2-2c+1) >= 0
<=>(a-1)2+(b-1)2+(c-1)2 >= 0 (luôn đúng)
Dấu "=" xảy ra chỉ khi và khi \(\hept{\begin{cases}a-1=0\\b-1=0\\c-1=0\end{cases}\Leftrightarrow a=b=c=1}\)
Vậy...
d, a2+b2+c2 >= 2(ab+bc-ca)
<=>a2+b2+c2-2ab-2bc+2ca >= 0
<=>(a-b-c)2 >= 0 (luôn đúng)
Dấu "=" xảy ra khi a=b=c
Vậy...
e,ta có: \(\frac{a^2+b^2}{2}\ge\left(\frac{a+b}{2}\right)^2\Leftrightarrow\frac{a^2+b^2}{2}-\left(\frac{a+b}{2}\right)^2\ge0\)
\(\Leftrightarrow\frac{2\left(a^2+b^2\right)}{4}-\frac{a^2+2ab+b^2}{4}\ge0\)
\(\Leftrightarrow\frac{2a^2+2b^2-a^2-2ab-b^2}{4}\ge0\)
\(\Leftrightarrow\frac{a^2-2ab+b^2}{4}\ge0\Leftrightarrow\left(\frac{a-b}{2}\right)^2\ge0\) (luôn đúng) (1)
Lại có: \(\left(\frac{a+b}{2}\right)^2\ge ab\Leftrightarrow\frac{a^2+2ab+b^2}{4}-\frac{4ab}{4}\ge0\)
\(\Leftrightarrow\frac{a^2+2ab+b^2-4ab}{4}\ge0\Leftrightarrow\left(\frac{a-b}{2}\right)^2\ge0\) (luôn đúng) (2)
Từ (1) và (2) => \(ab\le\left(\frac{a+b}{2}\right)^2\le\frac{a^2+b^2}{2}\)
Dấu "=" xảy ra khi a = b

1)Ta co
n5-5n3+4n
=n(n4-5n2+4)
=n(n4-n2-4n2+4)
=n(n2(n2-1)-4(n2-1)
=n(n2-4)(n2-1)
=n(n-1)(n+1)(n+2)(n-2)
vi n(n-1)(n+1)(n-2)(n+2) la h 5 so tu nhien lien tiep nen chia het cho 3,5,8 ma 3.5.8=120
=>n5-5n3+4n chia het 120

Ta có : \(b\ge a\left(gt\right)\) \(\Leftrightarrow\frac{b}{c}\ge\frac{a}{c}\left(\text{ c dương}\right)\Leftrightarrow\frac{c}{b}\ge\frac{c}{a}\) (1)
\(c\ge b\left(gt\right)\) \(\Leftrightarrow\frac{c}{a}\ge\frac{b}{a}\left(a\text{ }dương\right)\) (2)
\(c\ge a\left(gt\right)\) \(\Leftrightarrow\frac{c}{b}\ge\frac{a}{b}\left(b\text{ }\text{ dương}\right)\Leftrightarrow\frac{b}{c}\ge\frac{b}{a}\) (3)
Từ (1) , (2) và (3) ta có : \(\frac{c}{a}+\frac{b}{c}\ge\frac{b}{a}+\frac{a}{b}\)

sai đề rồi bạn.\(\frac{a}{b}>\frac{a+c}{b+c}\) với \(a>b\) mới đúng nha.
Ta có:\(A=\frac{10^{17}+1}{10^{16}+1}>\frac{10^{17}+1+9}{10^{16}+1+9}=\frac{10^{17}+10}{10^{16}+10}=\frac{10\left(10^{16}+1\right)}{10\left(10^{15}+1\right)}=\frac{10^{16}+1}{10^{15}+1}\)
\(\Rightarrow A>B\)
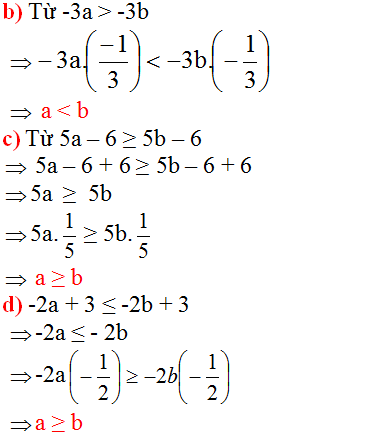

Ta có a - 3 > b - 3 ⇒ ( a - 3 ) + 3 > ( b - 3 ) + 3 ⇔ a > b
Chọn đáp án C.