HJ
2
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
CM
27 tháng 1 2019
Đáp án B.
Ta có 4 = 2 x + 2 y ≥ 2 2 x . 2 y = 2 2 x + y
⇔ 4 ≥ 2 x + y ⇔ x + y ≤ 2 .
Suy ra x y ≤ x + y 2 2 = 1
Khi đó
P = 2 x 3 + y 3 + 4 x 2 y 2 + 10 x y 2 x + y x + y 2 - 3 x y + 2 x y 2 + 10 x y
≤ 4 4 - 3 x y + 4 x 2 y 2 + 10 x y
= 16 + 2 x 2 y 2 + 2 x y x y - 1 ≤ 18
Vậy Pmax = 18 khi x = y = 1.
CM
12 tháng 11 2019
Đáp án C.
Ta có: GT
<=> 5x+2y + x + 2y – 3–x–2y = 5xy–1 – 31–xy + xy – 1.
X é t h à m s ố f t = 5 t + t - 3 - t
⇒ f t = 5 t ln 5 + 1 + 3 - t ln 3 > 0 ∀ t ∈ ℝ
Do đó hàm số đồng biến trên ℝ suy ra
f(x+2y) = f(xy – 1) <=> x+ 2y = xy – 1
⇔ x = 2 y + 1 y - 1 ⇒ T = 2 y + 1 y - 1 + y .
Do x > 0 => y > 1.
Ta có:
T = 2 + y + 3 y - 1 = 3 + y - 1 + 3 y - 1 ≥ 3 + 2 3 .
PN
1
15 tháng 6 2018
Ta có: \(x^2+2xy+7(x+y)+2y^2+10=0\)
<=> \((x^2+2xy+y^2)+7(x+y)+y^2+10=0\)
<=>(1)
Đặt t=x+y
=>(1)<=>\(y^2+t^2+7t+10=0
\)
Phương trình có nghiệm khi \(\Delta\)'\(\ge\)0
<=>\(t^2+7t+10=0
\) \(\le\)0
<=> -5\(\le\)t\(\le\)-2
=>Max S=1 khi t=-2<=>y=0;x=-2
Min S=-2 khi t=-5<=>y=0;x=-5
NV
Nguyễn Việt Lâm
Giáo viên
5 tháng 1 2021
\(4=2^x+2^y\ge2\sqrt{2^{x+y}}\Rightarrow2^{x+y}\le4\Rightarrow x+y\le2\)
\(\Rightarrow xy\le1\)
\(P=4x^2y^2+2x^3+2y^3+10xy\)
\(P=4x^2y^2+10xy+2\left(x+y\right)\left[\left(x+y\right)^2-3xy\right]\)
\(P\le4x^2y^2+10xy+4\left(4-3xy\right)=4x^2y^2-2xy+16\)
Đặt \(xy=t\Rightarrow0< t\le1\)
Xét hàm \(f\left(t\right)=4t^2-2t+16\) trên \((0;1]\)
\(\Rightarrow...\)
NV
Nguyễn Việt Lâm
Giáo viên
18 tháng 4 2020
\(4=2^x+2^y\ge2\sqrt{2^{x+y}}=2.2^{\frac{x+y}{2}}\)
\(\Rightarrow\frac{x+y}{2}\le1\Rightarrow x+y\le2\Rightarrow xy\le1\)
\(P=4x^2y^2+2x^3+2y^3+10xy\)
\(P=4x^2y^2+10xy+2\left(x+y\right)^3-6xy\left(x+y\right)\)
\(P=4x^2y^2-2xy+16=2\left(xy-1\right)\left(2xy+1\right)+18\)
Do \(xy\le1\Rightarrow2\left(xy-1\right)\left(2xy+1\right)\le0\Rightarrow P\le18\)
\(\Rightarrow P_{max}=18\) khi \(x=y=1\)
NV
Nguyễn Việt Lâm
Giáo viên
1 tháng 3 2021
Do \(1\le x\le2\Rightarrow\left(x-1\right)\left(x-2\right)\le0\)
\(\Leftrightarrow x^2+2\le3x\)
Hoàn toàn tương tự ta có \(y^2+2\le3y\)
Do đó: \(P\ge\dfrac{x+2y}{3x+3y+3}+\dfrac{2x+y}{3x+3y+3}+\dfrac{1}{4\left(x+y-1\right)}\)
\(P\ge\dfrac{x+y}{x+y+1}+\dfrac{1}{4\left(x+y-1\right)}\)
Đặt \(a=x+y-1\Rightarrow1\le a\le3\)
\(\Rightarrow P\ge f\left(a\right)=\dfrac{a+1}{a+2}+\dfrac{1}{4a}\)
\(f'\left(a\right)=\dfrac{3a^2-4a-4}{4a^2\left(a+2\right)^2}=\dfrac{\left(a-2\right)\left(3a+2\right)}{4a^2\left(a+2\right)^2}=0\Rightarrow a=2\)
\(f\left(1\right)=\dfrac{11}{12}\) ; \(f\left(2\right)=\dfrac{7}{8}\) ; \(f\left(3\right)=\dfrac{53}{60}\)
\(\Rightarrow f\left(a\right)\ge\dfrac{7}{8}\Rightarrow P_{min}=\dfrac{7}{8}\) khi \(\left(x;y\right)=\left(1;2\right);\left(2;1\right)\)
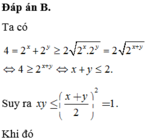
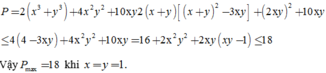
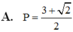
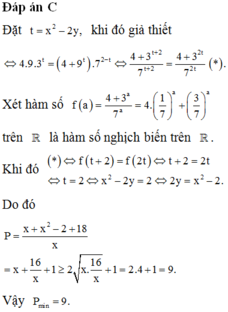
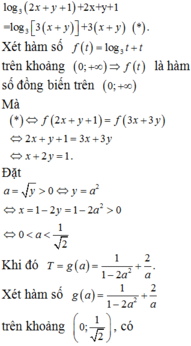
Lời giải:
Áp dụng BĐT Cauchy-Schwarz:
\((2x^2+3y^2)\left(\frac{1}{2}+\frac{4}{3}\right)\geq (x+2y)^2\)
\(\Leftrightarrow \frac{22}{3}\geq (x+2y)^2\Leftrightarrow x+2y\leq \sqrt{\frac{22}{3}}\)
Vậy \((x+2y)_{\max}=\sqrt{\frac{22}{3}}\)
Dấu bằng xảy ra khi \((x,y)=\left (\sqrt{\frac{6}{11}},4\sqrt{\frac{2}{33}}\right)\)
Cho 2 tập hợp A và B. Biết tập hợp B khác rỗng, số phần tử của tập B gấp đôi số phần tử của tập A∩B và A∪B có 10 phần tử. Hỏi tập A và B có bao nhiêu phần tử? Hãy xét các trường hợp xảy ra và dùng biểu đồ Ven minh họa?