Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: Phương trình hoành độ giao điểm là:
3x-4=4x-6
\(\Leftrightarrow3x-4x=-6+4\)
\(\Leftrightarrow-x=-2\)
hay x=2
Thay x=2 vào \(\left(d1\right)\), ta được:
\(y=3\cdot2-4=2\)
b: Thay y=0 vào \(\left(d1\right)\), ta được:
\(3x-4=0\)
hay \(x=\dfrac{4}{3}\)
Thay x=0 vào \(\left(d1\right)\), ta được:
\(y=3\cdot0-4=-4\)
Vậy: \(A\left(\dfrac{4}{3};0\right);B\left(0;-4\right)\)

a, HS Tự làm
b, Tìm được C(–2; –3) là tọa độ giao điểm của d 1 và d 2
c, Kẻ OH ⊥ AB (CH ⊥ Ox)
S A B C = 1 2 C H . A B = 9 4 (đvdt)

1) \(\left\{{}\begin{matrix}\left(d_1\right):y=2x\\\left(d_2\right):y=-\dfrac{1}{2}x+5\end{matrix}\right.\)
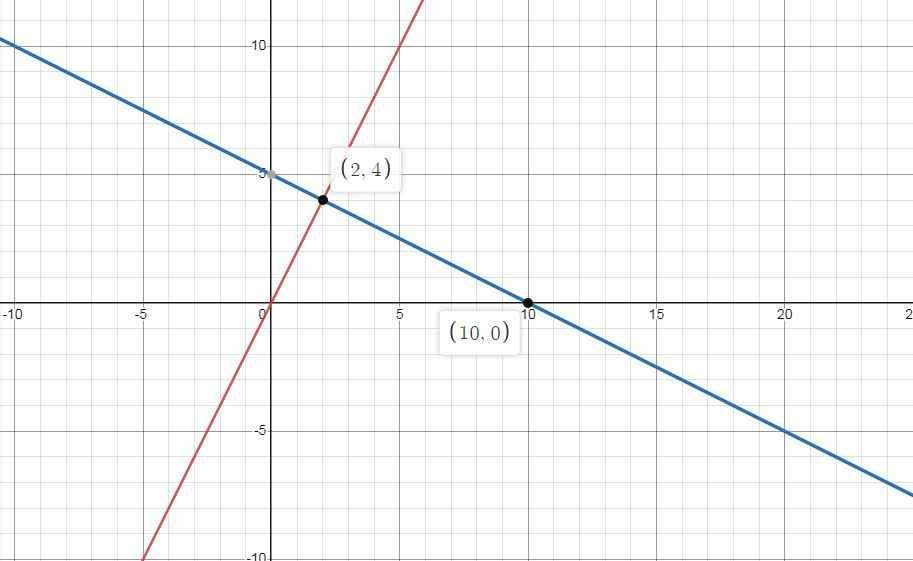
2) Theo đồ thi ta có :
\(\left(d_1\right)\cap\left(d_2\right)=A\left(2;4\right)\)
3) \(\left(d_2\right)\cap Ox=B\left(a;0\right)\)
\(\Leftrightarrow-\dfrac{1}{2}a+5=0\)
\(\Leftrightarrow\dfrac{1}{2}a=5\)
\(\Leftrightarrow a=10\)
\(\Rightarrow\left(d_2\right)\cap Ox=B\left(10;0\right)\)
4) \(OA=\sqrt[]{\left(2-0\right)^2+\left(4-0\right)^2}=\sqrt[]{20}=2\sqrt[]{5}\)
\(OB=\sqrt[]{\left(10-0\right)^2+\left(0-0\right)^2}=\sqrt[]{10^2}=10\)
\(AB=\sqrt[]{\left(10-2\right)^2+\left(0-4\right)^2}=\sqrt[]{80}=4\sqrt[]{5}\)
Ta thấy :
\(OA^2+AB^2=20+80=OB^2=100\)
\(\Rightarrow\Delta OAB\) vuông tại A
\(\Rightarrow\widehat{OAB}=90^o\)
\(sin\widehat{AOB}=\dfrac{AB}{OB}=\dfrac{4\sqrt[]{5}}{10}=\dfrac{2\sqrt[]{5}}{5}\)
\(\Rightarrow\widehat{AOB}\sim63,43^o\)
\(\Rightarrow\widehat{OBA}=90^o-63,43^o=26,57^o\)
5) Chu vi \(\Delta OAB\) :
\(AB+OA+OB=4\sqrt[]{5}+2\sqrt[]{5}+10=10\sqrt[]{5}+10=10\left(\sqrt[]{5}+1\right)\left(đvmd\right)\)
Diện tích \(\Delta OAB\) :
\(\dfrac{1}{2}AB.OA=\dfrac{1}{2}.4\sqrt[]{5}.2\sqrt[]{5}=20\left(đvdt\right)\)

a/ Bạn tự vẽ
b/ Ta lập pt hoành độ giao điểm :
(d1) giao với (d2) : \(-x-5=\frac{1}{4}x\Leftrightarrow x=-4\) thay vào (d1) được y = -1
Vậy A(-4;-1) . Tương tự ta tìm được điểm B(-1;-4)
c/ Ta có : \(AB=\sqrt{\left(x_B-x_A\right)^2+\left(y_B-y_A\right)^2}=\sqrt{\left(-1+4\right)^2+\left(-4+1\right)^2}=3\sqrt{2}\)
\(OA=\sqrt{x_A^2+y_A^2}=\sqrt{4^2+1^2}=\sqrt{17}\) ; \(OB=\sqrt{x_B^2+y_B^2}=\sqrt{1^2+4^2}=\sqrt{17}\)
=> OAB là tam giác cân
d/ Gọi OH là đường cao hạ từ O xuống AB (H thuộc AB)
Vì tam giác OAB cân tại O nên AH = HB = 1/2AB = \(\frac{3\sqrt{2}}{2}\)
\(OH=\sqrt{OA^2-BH^2}=\sqrt{17-\left(\frac{3\sqrt{2}}{2}\right)^2}=\frac{5\sqrt{2}}{2}\)
\(S_{ABC}=\frac{1}{2}AB.OH=\frac{1}{2}.3\sqrt{2}.\frac{5\sqrt{2}}{2}=\frac{15}{2}\)