
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.




a. \(A=\left(\dfrac{1}{\sqrt{x}-1}-\dfrac{1}{\sqrt{x}}\right):\left(\dfrac{\sqrt{x}+1}{\sqrt{x}-2}-\dfrac{\sqrt{x}+2}{\sqrt{x}-1}\right)\) (ĐKXĐ: \(x>0;x\ne1;x\ne4\))
\(=\left[\dfrac{\sqrt{x}}{\sqrt{x}\left(\sqrt{x}-1\right)}-\dfrac{\sqrt{x}-1}{\sqrt{x}\left(\sqrt{x}-1\right)}\right]:\left[\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)}-\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)}\right]\)
\(=\dfrac{\sqrt{x}-\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{x-1-\left(x-4\right)}{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{x-1-x+4}{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)}\)
\(=\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)}{3}\)
\(=\dfrac{\sqrt{x}-2}{3\sqrt{x}}\)
Vậy \(A=\dfrac{\sqrt{x}-2}{3\sqrt{x}}\).
\(---\)
b. Ta có: \(A=0\Leftrightarrow\dfrac{\sqrt{x}-2}{3\sqrt{x}}=0\)
\(\Leftrightarrow\sqrt{x}-2=0\)
\(\Leftrightarrow\sqrt{x}=2\)
\(\Leftrightarrow x=4\left(ktm\right)\)
Vậy không thể tìm được giá trị nào của \(x\) để \(A=0\).
\(---\)
c. Ta có: \(A< 0\Leftrightarrow\dfrac{\sqrt{x}-2}{3\sqrt{x}}< 0\)
\(\Leftrightarrow\sqrt{x}-2< 0\left(vì.3\sqrt{x}>0\right)\)
\(\Leftrightarrow\sqrt{x}< 2\)
\(\Leftrightarrow x< 4\)
Kết hợp với điều kiện xác định của \(x\), ta được:
\(0< x< 4;x\ne1\)
Vậy \(A< 0\) khi \(0< x< 4;x\ne1\).
a) \(A=\left(\dfrac{1}{\sqrt{x}-1}-\dfrac{1}{\sqrt{x}}\right):\left(\dfrac{\sqrt{x}+1}{\sqrt{x}-2}-\dfrac{\sqrt{x}+2}{\sqrt{x}-1}\right)\) (ĐK: \(x>0;x\ne1;x\ne4\))
\(A=\left[\dfrac{\sqrt{x}}{\sqrt{x}\left(\sqrt{x}-1\right)}-\dfrac{\sqrt{x}-1}{\sqrt{x}\left(\sqrt{x}-1\right)}\right]:\left[\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}-1\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-1\right)}-\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-1\right)}\right]\)
\(A=\dfrac{\sqrt{x}-\sqrt{x}+1}{\sqrt{x}\left(\sqrt{x}-1\right)}:\dfrac{x-1-x+4}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-1\right)}\)
\(A=\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}\cdot\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}-1\right)}{3}\)
\(A=\dfrac{\sqrt{x}-2}{3\sqrt{x}}\)
b) \(A=0\) khi
\(\dfrac{\sqrt{x}-2}{3\sqrt{x}}=0\)
\(\Rightarrow\sqrt{x}-2=0\)
\(\Rightarrow\sqrt{x}=2\)
\(\Rightarrow x=4\left(ktm\right)\)
c) \(A< 0\) khi
\(\dfrac{\sqrt{x}-2}{3\sqrt{x}}< 0\)
\(\Rightarrow\sqrt{x}-2< 0\)
\(\Rightarrow\sqrt{x}< 2\)
\(\Rightarrow x< 4\)
kết hợp với đk:
\(0< x< 4,x\ne1\)

a. Phương trình hoành độ giao điểm:
\(3x-5=-2x\)
\(\Leftrightarrow5x=5\)
\(\Rightarrow x=1\)
Thế vào \(y=3x-5\Rightarrow y=3.1-5=-2\)
Vậy \(A\left(1;-2\right)\)
b. Gọi phương trình d có dạng \(y=ax+b\)
Do d song song \(d_1\Rightarrow a=1\Rightarrow y=x+b\)
Do d qua A nên: \(y_A=x_A+b\Leftrightarrow-2=1+b\Rightarrow b=-3\)
Vậy pt d có dạng: \(y=x-3\)

Bài 1:
Phần a bạn tự làm nha! (Đ/S: 0,5)
b, B = \(\dfrac{\sqrt{x}+3}{\sqrt{x}-2}+\dfrac{\sqrt{x}+2}{3-\sqrt{x}}+\dfrac{\sqrt{x}+2}{x-5\sqrt{x}+6}\) với \(x\ge0;x\ne4;x\ne9\)
B = \(\dfrac{\sqrt{x}+3}{\sqrt{x}-2}-\dfrac{\sqrt{x}+2}{\sqrt{x}-3}+\dfrac{\sqrt{x}+2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\)
B = \(\dfrac{x-9}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}-\dfrac{x-4}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}+\dfrac{\sqrt{x}+2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\)
B = \(\dfrac{\sqrt{x}-3}{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}\)
B = \(\dfrac{1}{\sqrt{x}-2}=\dfrac{\sqrt{x}+2}{x-4}\)
Vậy ...
c, Ta có: A = \(1-\dfrac{\sqrt{x}}{\sqrt{x}+1}\)= \(\dfrac{1}{\sqrt{x}+1}\)
T = \(\dfrac{A}{B}\)= \(\dfrac{\sqrt{x}-2}{\sqrt{x}+1}\)= 1 - \(\dfrac{3}{\sqrt{x}+1}\)
Ta có: x \(\ge\) 0 \(\Leftrightarrow\) \(\sqrt{x}\ge0\) \(\Leftrightarrow\) \(\sqrt{x}+1\ge1\) \(\Leftrightarrow\) \(\dfrac{3}{\sqrt{x}+1}\le3\) \(\Leftrightarrow\) \(-\dfrac{3}{\sqrt{x}+1}\ge-3\) \(\Leftrightarrow\) T \(\ge\) -2
Vậy ...
Bài 2: ĐK: x \(\ge\) 0
Giả sử: \(P\) < \(\sqrt{P}\)
\(\Leftrightarrow\) \(\dfrac{\sqrt{x}+2}{\sqrt{x}+5}< \dfrac{\sqrt{\sqrt{x}+2}}{\sqrt{\sqrt{x}+5}}\)
\(\Leftrightarrow\) \(\dfrac{\sqrt{\left(\sqrt{x}+2\right)\left(\sqrt{x}+5\right)}-\left(\sqrt{x}+2\right)}{\sqrt{x}+5}>0\)
\(\Leftrightarrow\) \(\sqrt{\left(\sqrt{x}+2\right)\left(\sqrt{x}+5\right)}-\left(\sqrt{x}+2\right)>0\) (\(\sqrt{x}+5>0\) với mọi x \(\ge\) 0)
\(\Leftrightarrow\) \(\sqrt{\left(\sqrt{x}+2\right)}\sqrt{\sqrt{x}+5-\sqrt{x}-2}>0\)
\(\Leftrightarrow\) \(\sqrt{\left(\sqrt{x}+2\right)}\sqrt{3}>0\)
\(\Leftrightarrow\) \(\sqrt{\sqrt{x}+2}>0\)
Vì x \(\ge\) 0 \(\Leftrightarrow\) \(\sqrt{x}+2\ge2\) \(\Leftrightarrow\) \(\sqrt{\sqrt{x}+2}\ge\sqrt{2}>0\) (Đpcm)
Vậy \(P\) < \(\sqrt{P}\)
Chúc bn học tốt!

Bài 5:
a: ĐKXĐ: a>0; a<>1; a<>4
b: \(B=\dfrac{\sqrt{a}-\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}:\dfrac{a-1+a-4}{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}\)
\(=\dfrac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}{2a-5}\)
\(=\dfrac{\sqrt{a}-2}{\sqrt{a}\left(2a-5\right)}\)

DKXD : \(x\ge-1;y\ne-1\)
Dat : \(\left\{{}\begin{matrix}\sqrt{x+1}=a\left(a\ge0\right)\\y+1=b\left(b\ne0\right)\end{matrix}\right.\)
hpt<=>\(\left\{{}\begin{matrix}a+2-\dfrac{2}{y+1}=2\\2a-\dfrac{1}{y+1}=\dfrac{3}{2}\end{matrix}\right.\)
\(< =>\left\{{}\begin{matrix}a+2-\dfrac{2}{b}=2\\2a-\dfrac{1}{b}=\dfrac{3}{2}\end{matrix}\right.\)
\(< =>\left\{{}\begin{matrix}a-\dfrac{2}{b}=0\\4a-\dfrac{2}{b}=3\end{matrix}\right.< =>\left\{{}\begin{matrix}3a=3\\a=\dfrac{2}{b}\end{matrix}\right.< =>\left\{{}\begin{matrix}a=1\\b=2\end{matrix}\right.\)(tmdk)
\(=>\left\{{}\begin{matrix}x=0\\y=1\end{matrix}\right.\)(tmdk)

\(B=\left(\dfrac{\sqrt{x}}{\sqrt{x}-1}-\dfrac{1}{x-\sqrt{x}}\right).\dfrac{1}{\sqrt{x}+1}\)
\(\Rightarrow B=\left(\dfrac{x}{\sqrt{x}\left(\sqrt{x}-1\right)}-\dfrac{1}{\sqrt{x}\left(\sqrt{x}-1\right)}\right).\dfrac{1}{\sqrt{x}+1}\)
\(\Rightarrow B=\dfrac{x-1}{\sqrt{x}\left(\sqrt{x}-1\right)}.\dfrac{1}{\sqrt{x}+1}\)
\(\Rightarrow B=\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}{\sqrt{x}\left(\sqrt{x}-1\right)\left(\sqrt{x}+1\right)}\)
\(\Rightarrow B=\dfrac{1}{\sqrt{x}}\)
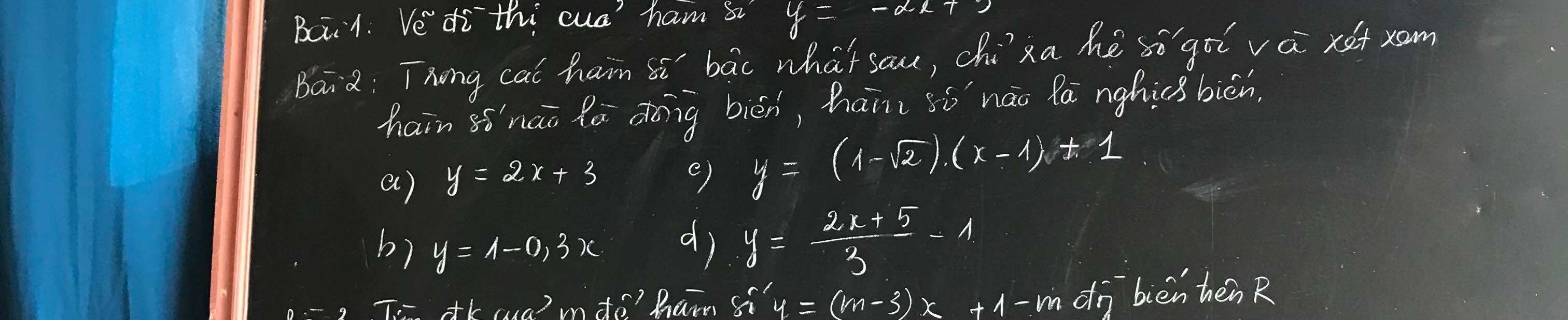


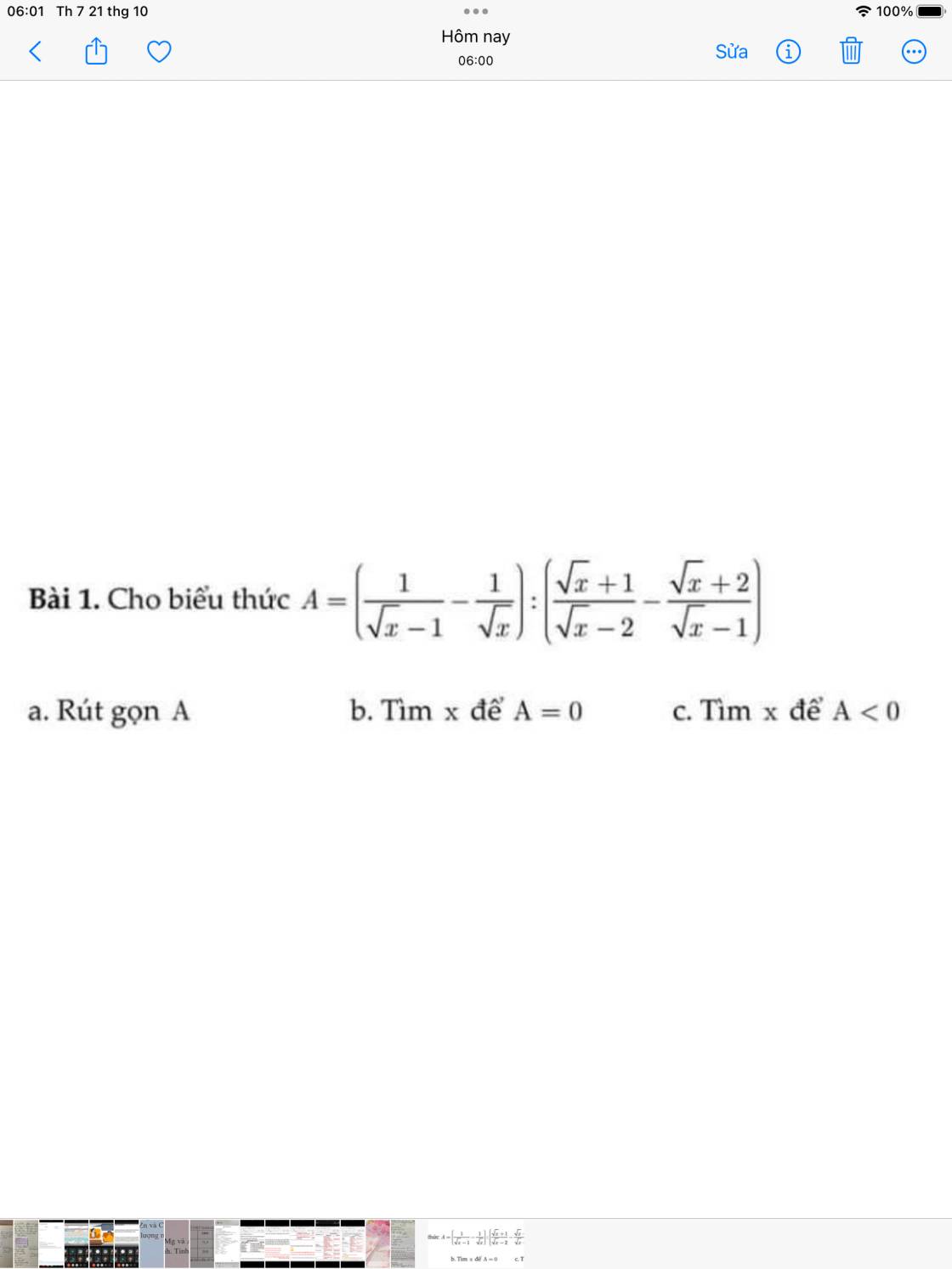


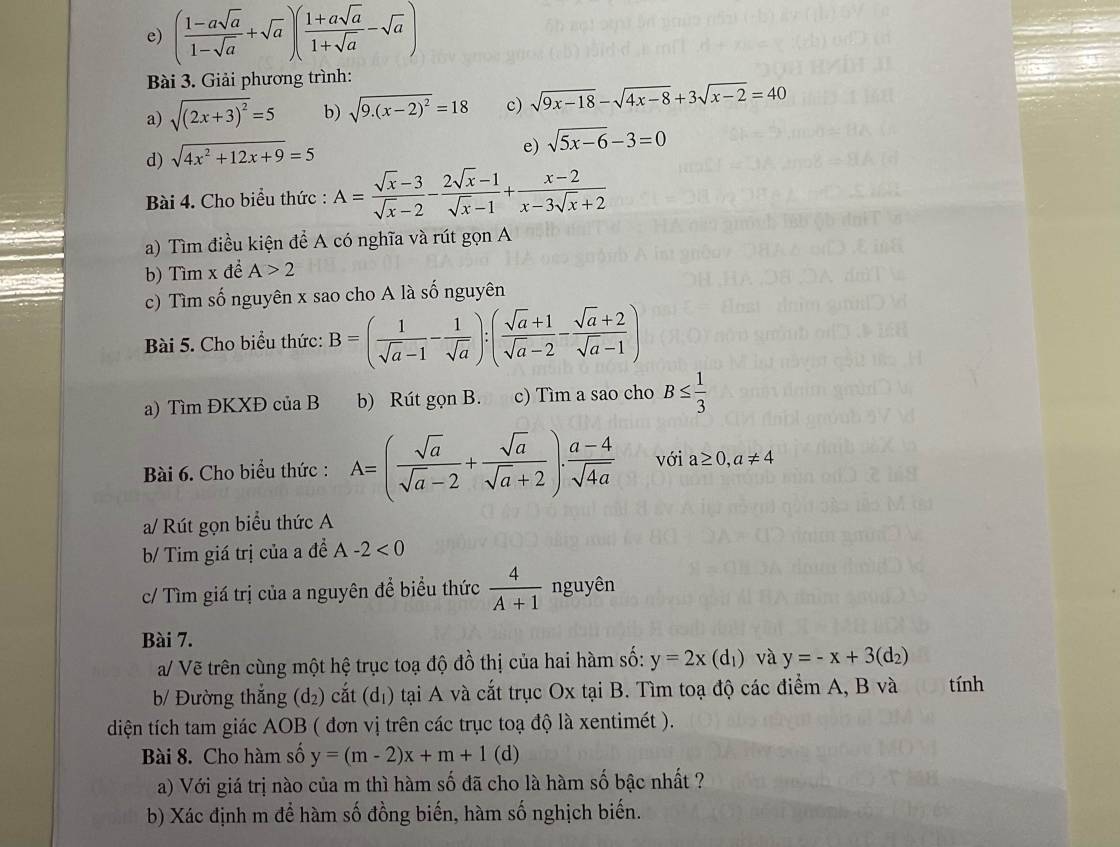

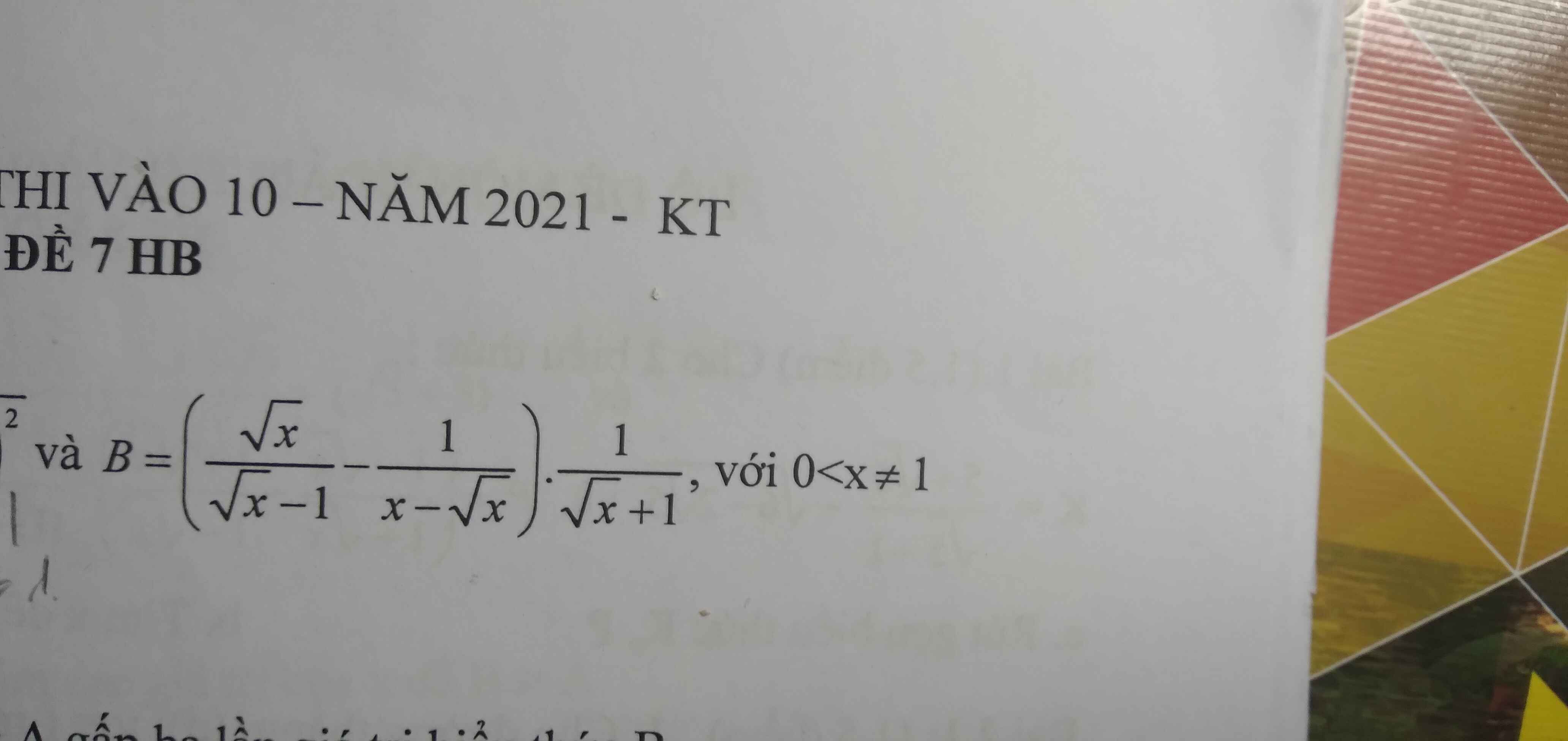
giúp gì vậy ạ??