
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a: ĐKXĐ: \(x\notin\left\{10;-10;\sqrt{10};-\sqrt{10}\right\}\)
b: \(A=\dfrac{5x^3+50x+2x^2+20+5x^3-50x-2x^2+20}{\left(x^2-10\right)\left(x^2+10\right)}\cdot\dfrac{x^2-100}{x^2+4}\)
\(=\dfrac{10x^3+40}{\left(x^2-10\right)\left(x^2+10\right)}\cdot\dfrac{x^2-100}{x^2+4}\)


a: \(4x^2-4x\)
\(=4x\cdot x-4x\cdot1\)
\(=4x\left(x-1\right)\)
b: \(x^2-2xy+y^2-4\)
\(=\left(x-y\right)^2-2^2\)
\(=\left(x-y-2\right)\left(x-y+2\right)\)

Đặt \(A=\left|x-2018\right|+\left|x-2019\right|+\left|x-2020\right|+\left|x-2021\right|\)
Ta có: \(\hept{\begin{cases}\left|x-2021\right|=\left|2021-x\right|\\\left|x-2020\right|=\left|2020-x\right|\end{cases}}\)
Ta lại có: \(\hept{\begin{cases}\left|x-2018\right|+\left|2021-x\right|\ge\left|x-2018+2021-x\right|=3\\\left|x-2019\right|+\left|2020-x\right|\ge\left|x-2019+2020-x\right|=1\end{cases}}\)
\(\Rightarrow\left|x-2018\right|+\left|x-2019\right|+\left|x-2020\right|+\left|x-2021\right|\ge1+3=4\)
\(\Rightarrow A_{min}=4\)
Dấu '=' xảy ra khi: \(\hept{\begin{cases}\left(x-2018\right).\left(2021-x\right)\ge0\\\left(x-2019\right).\left(2020-x\right)\ge0\end{cases}}\)
\(\Rightarrow\hept{\begin{cases}2018\le x\le2021\\2019\le x\le2020\end{cases}}\)\(\Rightarrow2018\le x\le2020\)
Vậy \(A_{min}=4\)\(\Leftrightarrow\)\(2018\le x\le2020\)
Nếu các bạn chưa hiểu chỗ suy ra ở chỗ dấu bằng xảy ra thì bạn hãy lập bảng xét dấu nhé ^_^
@#@@# Chúc bn hok tốt #@#@!


Bài 4:
\(\left\{{}\begin{matrix}a^4+b^4\ge2a^2b^2\\b^4+c^4\ge2b^2c^2\\c^4+a^4\ge2a^2c^2\end{matrix}\right.\\ \Leftrightarrow2\left(a^4+b^4+c^4\right)\ge2\left(a^2b^2+b^2c^2+c^2a^2\right)\\ \Leftrightarrow a^4+b^4+c^4\ge a^2b^2+b^2c^2+c^2a^2\left(1\right)\\ \left\{{}\begin{matrix}a^2b^2+b^2c^2\ge2ab^2c\\b^2c^2+c^2a^2\ge2abc^2\\c^2a^2+a^2b^2\ge2a^2bc\end{matrix}\right.\\ \Leftrightarrow2\left(a^2b^2+b^2c^2+c^2a^2\right)\ge2\left(a^2bc+ab^2c+abc^2\right)\\ \Leftrightarrow a^2b^2+b^2c^2+c^2a^2\ge a^2bc+ab^2c+abc^2=abc\left(a+b+c\right)\left(2\right)\\ \left(1\right)\left(2\right)\Leftrightarrow a^4+b^4+c^4\ge abc\left(a+b+c\right)\)
Dấu \("="\Leftrightarrow a=b=c\)

a: \(9x^3y^2+3x^2y^2\)
\(=3x^2y^2\cdot3x+3x^2y^2\cdot1\)
\(=3x^2y^2\left(3x+1\right)\)
b: \(x^2-2x+1-y^2\)
\(=\left(x^2-2x+1\right)-y^2\)
\(=\left(x-1\right)^2-y^2\)
\(=\left(x-1-y\right)\left(x-1+y\right)\)


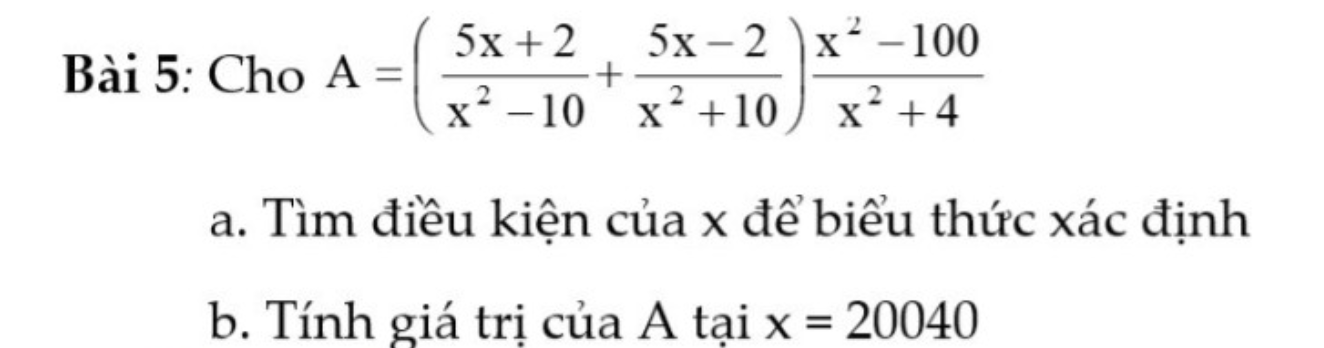 Các bạn giải giúp mình với ạ, phần a ghi chi tiết bước giải hộ mình nhé. Mình cám ơn ạ! (Mai mình nộp rồi nên nhanh giúp mình ạ T.T)
Các bạn giải giúp mình với ạ, phần a ghi chi tiết bước giải hộ mình nhé. Mình cám ơn ạ! (Mai mình nộp rồi nên nhanh giúp mình ạ T.T)
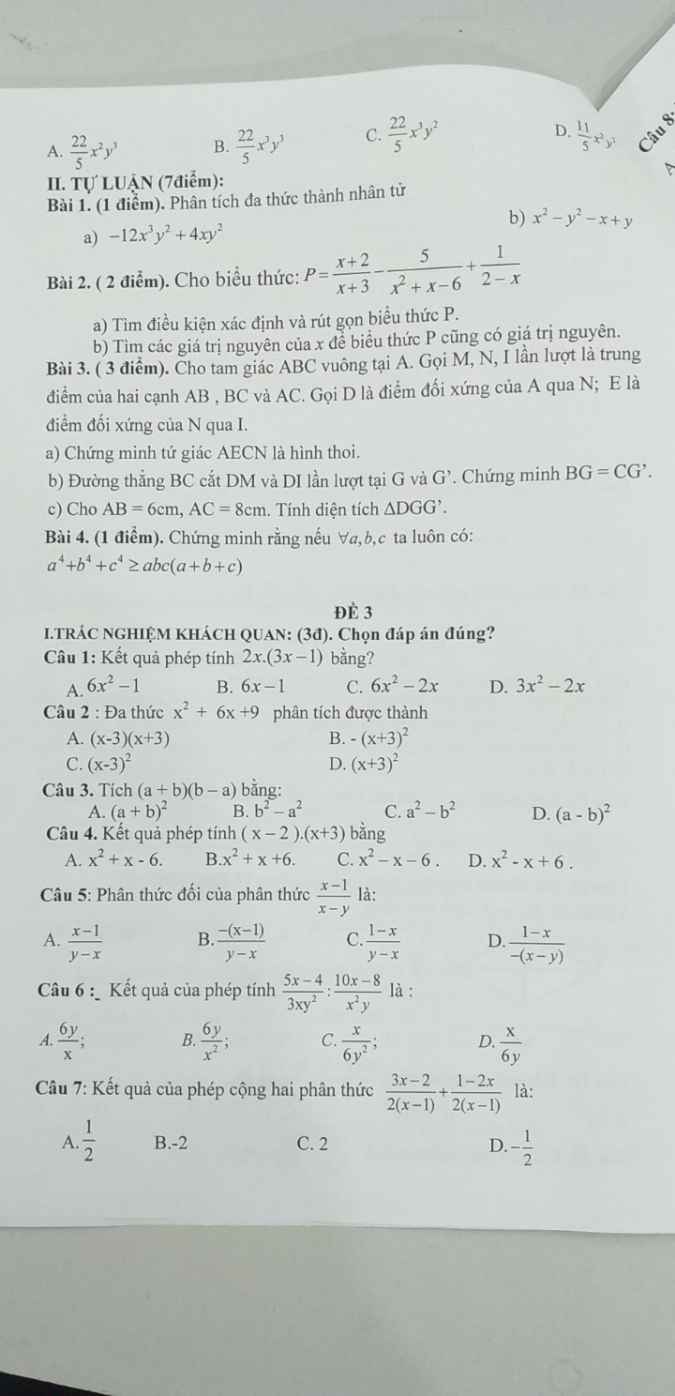
`2x - 3 = 5`
`<=> 2x = 5 + 3`
`<=> 2x = 8`
`=> x = 4`
Vậy `S = {4}`
_____________________
`3x - 4 = 11`
`<=> 3x = 11 + 4`
`<=> 3x = 15`
`=> x = 5`
Vậy `S = {5}`
______________
`(2x + 1)(x - 3) = 0`
`<=>` $\left[\begin{matrix} 2x + 1 = 0\\ x - 3 = 0\end{matrix}\right.$
`<=>` $\left[\begin{matrix} x = 1/2\\ x = 3\end{matrix}\right.$
Vậy `S = {1/2; -3}`
__________________
`(2x - 3)(x + 2) = 0`
`<=>` $\left[\begin{matrix} 2x - 3 = 0\\ x + 2 = 0\end{matrix}\right.$
`<=>` $\left[\begin{matrix} x = 3/2\\ x = -2\end{matrix}\right.$
Vậy `S = {-2; 3/2}`