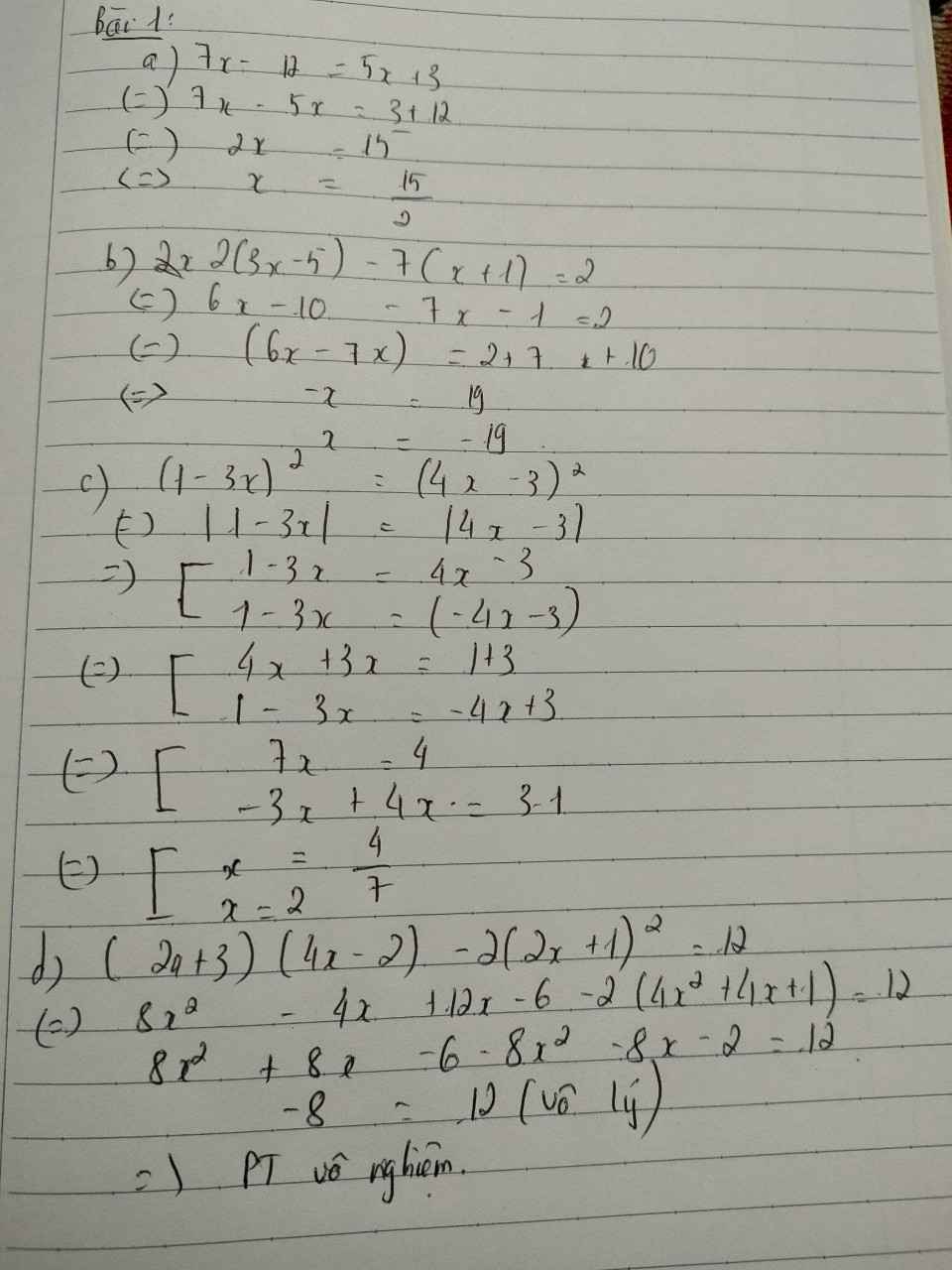Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: =(6x)^2-(3x-2)^2
=(6x-3x+2)(6x+3x-2)
=(9x-2)(3x+2)
d: \(=\left[\left(x+1\right)^2-\left(x-1\right)^2\right]\left[\left(x+1\right)^2+\left(x-1\right)^2\right]\)
\(=4x\cdot\left[x^2+2x+1+x^2-2x+1\right]\)
=8x(x^2+1)
e: =(4x)^2-2*4x*3y+(3y)^2
=(4x-3y)^2
f: \(=-\left(\dfrac{1}{4}x^4-2\cdot\dfrac{1}{2}x^2\cdot2y^3+4y^6\right)\)
\(=-\left(\dfrac{1}{2}x^2-2y^3\right)^2\)
g: =(4x)^3+1^3
=(4x+1)(16x^2-4x+1)
k: =x^3(27x^3-8)
=x^3(3x-2)(9x^2+6x+4)
l: =(x^3-y^3)(x^3+y^3)
=(x-y)(x+y)(x^2-xy+y^2)(x^2+xy+y^2)

b: \(x^3+\dfrac{1}{27}=\left(x+\dfrac{1}{3}\right)\left(x^2-\dfrac{1}{3}x+\dfrac{1}{9}\right)\)
c: \(x^3-3x^2+3x-1=\left(x-1\right)^3\)
e: \(a^2y^2-2axby+b^2x^2\)
\(=\left(ay\right)^2-2\cdot ay\cdot bx+\left(bx\right)^2\)
\(=\left(ay-bx\right)^2\)
f: \(100-\left(3x-y\right)^2\)
\(=\left(10-3x+y\right)\left(10+3x-y\right)\)
g: \(64x^2-\left(8a+b\right)^2\)
\(=\left(8x\right)^2-\left(8a+b\right)^2\)
\(=\left(8x-8a-b\right)\left(8x+8a+b\right)\)

Bài 2:
a: \(3x^2-3xy=3x\left(x-y\right)\)
b: \(x^2-4y^2=\left(x-2y\right)\left(x+2y\right)\)
c: \(3x-3y+xy-y^2=\left(x-y\right)\left(3+y\right)\)
d: \(x^2-y^2+2y-1=\left(x-y+1\right)\left(x+y-1\right)\)

a) \(x^4-y^4\)
\(=\left(x^2\right)^2-\left(y^2\right)^2\)
\(=\left(x^2-y^2\right)\left(x^2+y^2\right)\)
\(=\left(x+y\right)\left(x-y\right)\left(x^2+y^2\right)\)
b) \(x^2-3y^2\)
\(=x^2-\left(y\sqrt{3}\right)^2\)
\(=\left(x-y\sqrt{3}\right)\left(x+y\sqrt{3}\right)\)
c) \(\left(3x-2y\right)^2-\left(2x-3y\right)^2\)
\(=\left(3x-2y+2x-3y\right)\left(3x-2y-2x+3y\right)\)
\(=\left(5x-5y\right)\left(x+y\right)\)
\(=5\left(x-y\right)\left(x+y\right)\)
d) \(9\left(x-y\right)^2-4\left(x+y\right)^2\)
\(=\left[3\left(x-y\right)+2\left(x+y\right)\right]\left[3\left(x-y\right)-2\left(x+y\right)\right]\)
\(=\left(3x-3y+2x+2y\right)\left(3x-3y-2x-2y\right)\)
\(=\left(5x-y\right)\left(x-5y\right)\)
e) \(\left(4x^2-4x+1\right)-\left(x+1\right)^2\)
\(=\left(2x-1\right)^2-\left(x+1\right)\)
\(=\left(2x-1+x+1\right)\left(2x-1-x-1\right)\)
\(=3x\left(x-2\right)\)
f) \(x^3+27\)
\(=x^3+3^3\)
\(=\left(x+3\right)\left(x^2-3x+9\right)\)
g) \(27x^3-0,001\)
\(=\left(3x\right)^3-\left(0,1\right)^3\)
\(=\left(3x-0,1\right)\left(9x^2+0,3x+0,01\right)\)
h) \(125x^3-1\)
\(=\left(5x\right)^3-1^3\)
\(=\left(5x-1\right)\left(25x^2+5x+1\right)\)

\(2x\left(x^2-7x-3\right)=2x^3-14x-6x\)
\(4xy^2\left(-2x^3+y^2-7xy\right)=-8x^4y^2+4xy^5-28x^2y^3\)

1.
a.\(\Leftrightarrow7x-5x=3+12\)
\(\Leftrightarrow2x=15\Leftrightarrow x=\dfrac{15}{2}\)
b.\(\Leftrightarrow6x-10-7x-7=2\)
\(\Leftrightarrow x=-19\)
c.\(\Leftrightarrow1-3x=4x-3\)
\(\Leftrightarrow7x=2\Leftrightarrow x=\dfrac{2}{7}\)
d.\(\Leftrightarrow8x^2-4x+12x-6-8x^2-8x-2=12\)
\(\Leftrightarrow-2=12\left(voli\right)\)

A = 2x2 - 6xy - 3xy - 6y - 2x2 + 8xy + 6y
= - xy
= \(\frac{2}{3}\)\(x\)\(\frac{3}{4}\)
= \(\frac{1}{2}\)
mk đang bận mấy câu kia tương tự nha

a) =(x-y)*(x+y)-(5*(x+y))
=(x+y)*(x-y-5)
Mấy bài còn lại cũng tương tự nha bạn = cách đặt nhân tử chung
bai nao khong hieu thi pan nhan tin vào nick minh minh se giai đùm ban
a) (x2 - y2) - 5(x + y)
= (x - y)(x + y) - 5 (x + y)
= (x + y) (x - y -5)
b) 5x3 - 5x2y - 10x2 + 10 xy
= 5[(x3 - x2y) - (2x2 - 2 xy)]
=5[x2(x - y) - 2x(x - y)]
=5x(x-y)(x - 2)
c) 2x2 - 5x = x(2x - 5)
d) x3 - 3x2 +1 - 3x
= (x3 + 1) - (3x2 + 3x)
= (x + 1)(x2 - x + 1) - 3x(x + 1)
= (x + 1) [x2 - x + 1 - 3x]
= (x + 1)[x2 - 4x + 1]
= (x + 1)[x2 - 2.x.2 + 22 - 22 + 1]
= (x + 1)[(x - 2)2 - 3]
= \(\left(x+1\right)\left(x-2+\sqrt{3}\right)\left(x-2-\sqrt{3}\right)\)
e) 3x2 - 6xy + 3y2 - 12z2
= 3[ x2 - 2xy + y2 - 4z2]
= 3[ (x - y)2 - (2z)2]
= 3(x - y + 2z)(x - y - 2z)
f) 3x2 - 7x - 10
= 3x2 - 7x - 7 - 3
= (3x2 -3) - (7x + 7)
= 3(x2 - 1) - 7(x + 1)
= 3 (x + 1)(x - 1) - 7(x + 1)
= (x + 1)[3(x - 1) - 7]
= (x +1)(3x - 8)
g) x4 + 1 - 2x2 = (x2)2 - 2.x2 + 1 = (x2 - 1)2
= (x + 1)2(x - 1)2
h) 3x2 - 3y2 - 12x + 12y
= 3(x2 - y2) - 12(x - y)
= 3(x - y)(x + y) - 12(x -y)
= (x - y) [3(x + y) - 12]
= (x - y). 3. (x+y - 4)
j) x2 - 3x + 2 = x2 - x - 2x +2
= x(x - 1) - 2(x -1)
=(x - 1)(x - 2)