Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

LG a
(1−a√a1−√a+√a).(1−√a1−a)2=1(1−aa1−a+a).(1−a1−a)2=1 với a≥0a≥0 và a≠1a≠1
Phương pháp giải:
+ Biến đối vế trái thành vế phải ta sẽ có điều cần chứng minh.
+ √A2=|A|A2=|A|.
+ |A|=A|A|=A nếu A≥0A≥0,
|A|=−A|A|=−A nếu A<0A<0.
+ Sử dụng các hằng đẳng thức:
a2+2ab+b2=(a+b)2a2+2ab+b2=(a+b)2
a2−b2=(a+b).(a−b)a2−b2=(a+b).(a−b).
a3−b3=(a−b)(a2+ab+b2)a3−b3=(a−b)(a2+ab+b2).
Lời giải chi tiết:
Biến đổi vế trái để được vế phải.
Ta có:
VT=(1−a√a1−√a+√a).(1−√a1−a)2VT=(1−aa1−a+a).(1−a1−a)2
=(1−(√a)31−√a+√a).(1−√a(1−√a)(1+√a))2=(1−(a)31−a+a).(1−a(1−a)(1+a))2
=((1−√a)(1+√a+(√a)2)1−√a+√a).(11+√a)2=((1−a)(1+a+(a)2)1−a+a).(11+a)2
=[(1+√a+(√a)2)+√a].1(1+√a)2=[(1+a+(a)2)+a].1(1+a)2
=[(1+2√a+(√a)2)].1(1+√a)2=[(1+2a+(a)2)].1(1+a)2
=(1+√a)2.1(1+√a)2=1=VP=(1+a)2.1(1+a)2=1=VP.
LG b
a+bb2√a2b4a2+2ab+b2=|a|a+bb2a2b4a2+2ab+b2=|a| với a+b>0a+b>0 và b≠0b≠0
Phương pháp giải:
+ Biến đối vế trái thành vế phải ta sẽ có điều cần chứng minh.
+ √A2=|A|A2=|A|.
+ |A|=A|A|=A nếu A≥0A≥0,
|A|=−A|A|=−A nếu A<0A<0.
+ Sử dụng các hằng đẳng thức:
a2+2ab+b2=(a+b)2a2+2ab+b2=(a+b)2
a2−b2=(a+b).(a−b)a2−b2=(a+b).(a−b).
a3−b3=(a−b)(a2+ab+b2)a3−b3=(a−b)(a2+ab+b2).
Lời giải chi tiết:
Ta có:
VT=a+bb2√a2b4a2+2ab+b2VT=a+bb2a2b4a2+2ab+b2
=a+bb2√(ab2)2(a+b)2=a+bb2(ab2)2(a+b)2
=a+bb2√(ab2)2√(a+b)2=a+bb2(ab2)2(a+b)2
=a+bb2|ab2||a+b|=a+bb2|ab2||a+b|
=a+bb2.|a|b2a+b=|a|=VP=a+bb2.|a|b2a+b=|a|=VP
Vì a+b>0⇒|a+b|=a+ba+b>0⇒|a+b|=a+b.

a) \(M=\dfrac{1+\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-1\right)}.\dfrac{\left(\sqrt{a}-1\right)^2}{\sqrt{a}+1}=\dfrac{\sqrt{a}-1}{\sqrt{a}}\)
b) \(M=\dfrac{\sqrt{a}-1}{\sqrt{a}}=1-\dfrac{1}{\sqrt{a}}< 1\)
c) \(M=\dfrac{\sqrt{a}-1}{\sqrt{a}}=\dfrac{\sqrt{3-2\sqrt{2}}-1}{\sqrt{3-2\sqrt{2}}}=\dfrac{\sqrt{\left(\sqrt{2}-1\right)^2}-1}{\sqrt{\left(\sqrt{2}-1\right)^2}}=\dfrac{\sqrt{2}-1-1}{\sqrt{2}-1}=\dfrac{\sqrt{2}-2}{\sqrt{2}-1}\)
\(a,M=\dfrac{1+\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\left(\sqrt{a}-1\right)^2}{\sqrt{a}+1}=\dfrac{\sqrt{a}-1}{\sqrt{a}}\\ b,M=1-\dfrac{1}{\sqrt{a}}< 1\\ c,a=3-2\sqrt{2}\Leftrightarrow\sqrt{a}=\sqrt{\left(\sqrt{2}-1\right)^2}=\sqrt{2}-1\\ \Leftrightarrow M=\dfrac{\sqrt{2}-1-1}{\sqrt{2}-1}=\dfrac{\sqrt{2}-2}{\sqrt{2}-1}=\dfrac{-\sqrt{2}\left(\sqrt{2}-1\right)}{\sqrt{2}-1}=-\sqrt{2}\)


bạn tham khảo nha : https://loigiaihay.com/bai-76-trang-41-sgk-toan-9-tap-1-c44a26988.html

a) \(M=3\sqrt{3}-\sqrt{12}-\sqrt{\left(\sqrt{3}-1\right)^2}\)
\(M=3\sqrt{3}-2\sqrt{3}-\left|\sqrt{3}-1\right|\)
\(M=\sqrt{3}-\sqrt{3}+1\)
\(M=1\)
b) Ta có:
\(N=\left(\dfrac{1}{a-\sqrt{a}}+\dfrac{1}{\sqrt{a}-1}\right):\dfrac{\sqrt{a}+1}{a-2\sqrt{a}+1}\)
\(N=\left(\dfrac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}+\dfrac{\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-1\right)}\right):\dfrac{\sqrt{a}+1}{\left(\sqrt{a}-1\right)^2}\)
\(N=\left(\dfrac{1+\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-1\right)}\right)\cdot\dfrac{\left(\sqrt{a}-1\right)^2}{\sqrt{a}+1}\)
\(N=\dfrac{\left(\sqrt{a}+1\right)\cdot\left(\sqrt{a}-1\right)^2}{\sqrt{a}\left(\sqrt{a}-1\right)\cdot\left(\sqrt{a}+1\right)}\)
\(N=\dfrac{\sqrt{a}-1}{\sqrt{a}}\)
Theo đề ta có: \(M=2N\)
Khi: \(1=2\cdot\left(\dfrac{\sqrt{a}-1}{\sqrt{a}}\right)\)
\(\Leftrightarrow1=\dfrac{2\sqrt{a}-2}{\sqrt{a}}\)
\(\Leftrightarrow\sqrt{a}=2\sqrt{a}-2\)
\(\Leftrightarrow2\sqrt{a}-\sqrt{a}=2\)
\(\Leftrightarrow\sqrt{a}=2\)
\(\Leftrightarrow a=4\left(tm\right)\)

\(M=\dfrac{a\sqrt{a}-b\sqrt{b}-a\sqrt{a}+a\sqrt{b}+b\sqrt{a}+b\sqrt{b}}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}-\sqrt{b}\right)}\\ M=\dfrac{a\sqrt{b}+b\sqrt{a}}{\left(\sqrt{a}+\sqrt{b}\right)\left(\sqrt{a}-\sqrt{b}\right)}=\dfrac{\sqrt{ab}\left(\sqrt{a}+\sqrt{b}\right)}{\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{a}+\sqrt{b}\right)}\\ M=\dfrac{\sqrt{ab}}{\sqrt{a}-\sqrt{b}}\)
\(\left(1-a\right)\left(1-b\right)+2\sqrt{ab}=1\\ \Leftrightarrow1-a-b+ab+2\sqrt{ab}=1\\ \Leftrightarrow a+b-ab-2\sqrt{ab}=0\\ \Leftrightarrow\left(\sqrt{a}-\sqrt{b}\right)^2=ab\\ \Leftrightarrow\left[{}\begin{matrix}\sqrt{a}-\sqrt{b}=\sqrt{ab}\\\sqrt{a}-\sqrt{b}=-\sqrt{ab}\end{matrix}\right.\)
Với \(\sqrt{a}-\sqrt{b}=\sqrt{ab}\Leftrightarrow M=\dfrac{\sqrt{ab}}{\sqrt{ab}}=1\)
Với \(\sqrt{a}-\sqrt{b}=-\sqrt{ab}\Leftrightarrow M=\dfrac{\sqrt{ab}}{-\sqrt{ab}}=-1\)
\(M=\dfrac{a\sqrt{a}-b\sqrt{b}-a\left(\sqrt{a}-\sqrt{b}\right)+b\left(\sqrt{a}+\sqrt{b}\right)}{\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{a}+\sqrt{b}\right)}\)
\(=\dfrac{a\sqrt{b}+b\sqrt{a}}{\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{a}+\sqrt{b}\right)}=\dfrac{\sqrt{ab}\left(\sqrt{a}+\sqrt{b}\right)}{\left(\sqrt{a}-\sqrt{b}\right)\left(\sqrt{a}+\sqrt{b}\right)}=\dfrac{\sqrt{ab}}{\sqrt{a}-\sqrt{b}}\)
\(\left(1-a\right)\left(1-b\right)+2\sqrt{ab}=1\)
\(\Leftrightarrow a+b-ab-2\sqrt{ab}=0\)
\(\Leftrightarrow\left(\sqrt{a}-\sqrt{b}\right)^2=ab\Leftrightarrow\sqrt{a}-\sqrt{b}=\sqrt{ab}\)
\(M=\dfrac{\sqrt{ab}}{\sqrt{a}-\sqrt{b}}=\dfrac{\sqrt{ab}}{\sqrt{ab}}=1\)

a) Ta có : Vì \(x\ge0\)và \(y\ge0\)nên \(x+y\ge0\)\(\Leftrightarrow\left|x+y\right|=x+y\)
\(\frac{2}{x^2-y^2}\sqrt{\frac{3\left(x+y\right)^2}{2}}\)
\(=\frac{2}{x^2-y^2}\sqrt{\frac{3}{2}.\left(x+y\right)^2}\)
\(=\frac{2}{x^2-y^2}.\sqrt{\frac{3}{2}}.\left|x+y\right|\)
\(=\frac{2}{\left(x-y\right)\left(x+y\right)}.\sqrt{\frac{3}{2}}.\left(x+y\right)\)
\(=\frac{2}{x-y}.\sqrt{\frac{3}{2}}\)
\(=\frac{1}{x-y}.2.\sqrt{\frac{3}{2}}\)
\(=\frac{1}{x-y}.\sqrt{\frac{2^2.3}{2}}\)
\(=\frac{1}{x-y}.\sqrt{6}=\frac{\sqrt{6}}{x-y}\)
a, \(\frac{2}{x^2-y^2}\sqrt{\frac{3\left(x+y\right)^2}{2}}=\frac{2}{x^2-y^2}\frac{\sqrt{3}\left|x+y\right|}{\sqrt{2}}=\frac{2\sqrt{3}\left(x+y\right)}{\left(x-y\right)\left(x+y\right)\sqrt{2}}\)
do \(x\ge0;y\ge0\)
\(=\frac{2\sqrt{3}}{\sqrt{2}\left(x-y\right)}=\frac{2\sqrt{6}}{2\left(x-y\right)}=\frac{\sqrt{6}}{x-y}\)

3:
a: ĐKXĐ: \(\left\{{}\begin{matrix}x>=0\\x< >9\end{matrix}\right.\)
\(M=\left(\dfrac{1}{\sqrt{x}-3}-\dfrac{1}{\sqrt{x}+3}\right):\dfrac{3}{\sqrt{x}-3}\)
\(=\dfrac{\sqrt{x}+3-\sqrt{x}+3}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\cdot\dfrac{\sqrt{x}-3}{3}\)
\(=\dfrac{6}{3\left(\sqrt{x}+3\right)}=\dfrac{2}{\sqrt{x}+3}\)
b: M>1/3
=>M-1/3>0
=>\(\dfrac{2}{\sqrt{x}+3}-\dfrac{1}{3}>0\)
=>\(\dfrac{6-\sqrt{x}-3}{3\left(\sqrt{x}+3\right)}>0\)
=>\(3-\sqrt{x}>0\)
=>\(\sqrt{x}< 3\)
=>0<=x<9
c: \(\sqrt{x}+3>=3\) với mọi x thỏa mãn ĐKXĐ
=>\(M=\dfrac{2}{\sqrt{x}+3}< =\dfrac{2}{3}\) với mọi x thỏa mãn ĐKXĐ
Dấu = xảy ra khi x=0
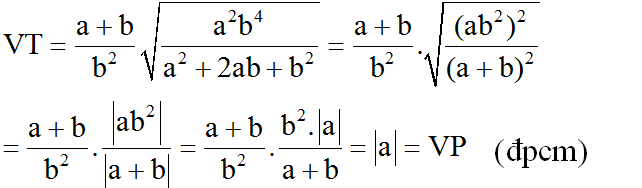
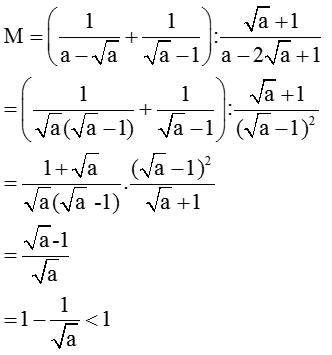
Rút gọn ta được:
M=√a−1/√a
Viết M ở dạng M=1−1/√a
suy ra M<1
Với \(x>0;x\ne1\)
\(M=\left(\frac{1}{a-\sqrt{a}}+\frac{1}{\sqrt{a}-1}\right):\frac{\sqrt{a}+1}{a-2\sqrt{a}+1}\)
\(=\left(\frac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}+\frac{\sqrt{a}}{\sqrt{a}\left(\sqrt{a}-1\right)}\right):\frac{\sqrt{a}+1}{\left(\sqrt{a}-1\right)^2}\)
\(=\frac{\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}.\frac{\left(\sqrt{a}-1\right)^2}{\sqrt{a}+1}=\frac{\sqrt{a}-1}{\sqrt{a}}\)
\(=1-\frac{1}{\sqrt{a}}< 1\)hay M < 1