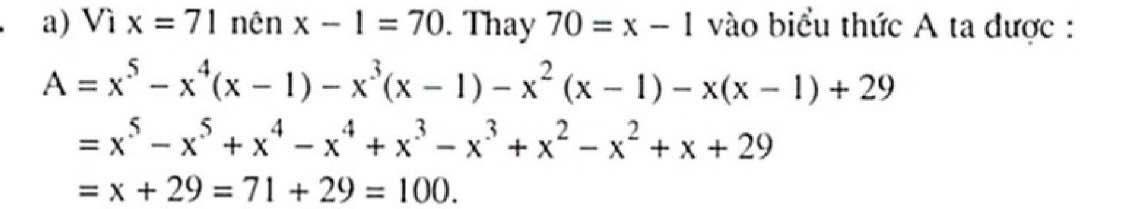K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
24 tháng 7 2021
Ta có: x=71
nên x-1=70
Ta có: \(A=x^5-70x^4-70x^3-70x^2-70x^2-70x+29\)
\(=x^5-x^4\left(x-1\right)-x^3\left(x-1\right)-x^2\left(x-1\right)-x\left(x-1\right)+29\)
\(=x^5-x^5+x^4-x^4+x^3-x^3+x^2-x^2+x+29\)
=x+29
=71+29
=100
27 tháng 8 2019
Cách 1: Ta có x=71=>x-71=0
A=x5-71x4+x4-71x3+x3-71x2+x2-71x+x-71+100
=x4(x-71)+x3(x-71)+x2(x-71)+x(x-71)+(x-71)+100
=100
Vậy A=100 tại giá trị x=71
Cách 2: vì x=71=>x-1=70
A=x5-(x-1)x4-(x-1)x3-(x-1)x2-(x-1)x+29
=x5-x5+x4-x4+x3-x3+x2-x2+x+29
=x+29=71+29=100
Vậy A=100 tại giá trị x=71
Cách 3: Thay trực tiếp x=71 vào biểu thức A, cách này không hay cho lắm
27 tháng 8 2019
Ta có x=71=>x-71=0
A=x5-71x4+x4-71x3+x3-71x2+x2-71x+x-71+100
=x4(x-71)+x3(x-71)+x2(x-71)+x(x-71)+(x-71)+100
=100
Vậy A=100 tại giá trị x=71
.,.............học tốt................
DL
2
LM
8 tháng 7 2018
\(A=x^5-70x^4-70x^3-70x^2-70x+29\) (ở đây mình có sửa đề nha, vì nếu để +70x2 thì sẽ không đúng với quy luật của bài toán và kết quả sẽ rất lớn)\(\Leftrightarrow A=x^5-71x^4+x^4-71x^3+x^3-71x^2+x^2-71x+x-71+100\)\(\Leftrightarrow A=x^4\left(x-71\right)+x^3\left(x-71\right)+x^2\left(x-71\right)+x\left(x-71\right)+\left(x-71\right)+100\)\(\Leftrightarrow A=\left(x-71\right)\left(x^4+x^3+x^2+x+1\right)+100\)
Với x = 71 thì:
\(A=\left(71-71\right)\left(71^4+71^3+71^2+71+1\right)+100\) \(\Leftrightarrow A=0\times\left(71^4+71^3+71^2+71+1\right)+100\)
\(\Leftrightarrow A=100\)
LM
8 tháng 7 2018
Nếu không sửa đề thì bài toán sẽ là:
\(A=x^5-70x^4-70x^3+70x^2-70x+29\)\(\Leftrightarrow A=x^5-71x^4+x^4-71x^3+x^3-71x^2+141x^2-10011x+9941x-705811+705840\)\(\Leftrightarrow A=x^4\left(x-71\right)+x^3\left(x-71\right)+x^2\left(x-71\right)+141x\left(x-71\right)+9941\left(x-71\right)+705840\)\(\Leftrightarrow A=\left(x-71\right)\left(x^4+x^3+x^2+141x+9941\right)+705840\)
Với x = 71 thì:
\(A=\left(71-71\right)\left(71^4+71^3+71^2+141\times71+9941\right)+705840\) \(\Leftrightarrow A=0\times\left(71^4+71^3+71^2+141\times71+9941\right)+705840\)
\(\Leftrightarrow A=705840\)
27 tháng 8 2017
\(A=x^5-70x^4-70x^3-70x^2-70x+34\)
\(=x^5-71x^4+x^4-71x^3+x^3-71x^2+x^2-71x+x-71+105\)
\(=x^4\left(x-71\right)+x^3\left(x-71\right)+x^2\left(x-71\right)+x\left(x-71\right)+\left(x-71\right)+105\)
\(=\left(x^4+x^3+x^2+x+1\right)\left(x-71\right)+105\)
Thay x = 71\(\Rightarrow A=105\)
Vậy...
18 tháng 9 2021
\(x=71\Leftrightarrow x-1=70\\ \Leftrightarrow A=x^5-\left(x-1\right)x^4-\left(x-1\right)x^3-\left(x-1\right)x^2-\left(x-1\right)x+34\\ A=x^5-x^5+x^4-x^4+x^3-x^3+x^2-x^2-x+x+34=34\)
18 tháng 9 2021
\(A=x^5-70x^4-70x^3-70x^2-70x+34\)
\(=x^4\left(x-71\right)+x^3\left(x-71\right)+x^2\left(x-71\right)+x^2\left(x-71\right)+x\left(x-71\right)+x+34\)
\(=x^4\left(71-71\right)+...+x\left(71-71\right)+71+34\)
\(=x^4.0+...+x.0+105=105\)
KB
15 tháng 9 2018
a ) Nếu \(x=71\) \(\Rightarrow70=x-1\)
Thay \(70=x-1\) vào A , ta được :
\(A=x^5-\left(x-1\right)x^4-\left(x-1\right)x^3-\left(x-1\right)x^2-\left(x-1\right)x\)
\(=x^5-x^5+x^4-x^4+x^3-x^3+x^2-x^2+x\)
\(=x\)
\(=71\)
Vậy \(A=71\) tại \(x=71\)
b ) Ta có : \(x=35\)
\(\Rightarrow\left\{{}\begin{matrix}36=x+1\\37=x+2\\69=2x-1\\34=x-1\end{matrix}\right.\) ( * )
Thay ( * ) vào B , ta được :
\(B=x^5-\left(x+1\right)x^4+\left(x+2\right)x^3-\left(2x-1\right)x^2-\left(x-1\right)x+15\)
\(=x^5-x^5-x^4+x^4+2x^3-2x^3+x^2-x^2+x+15\)
\(=x+15\)
\(=35+15=50\)
Vậy \(B=50\) tại \(x=35\)
ND
1
T
29 tháng 8 2018
A=x5-70x4-70x3-70x2-70x+34 taị x=71
x=71⇒ x-1=71-1=70
A=x5- (x-1)x4 - (x-1)x3-(x-1)x2-(x-1)x + 34
A= x5- x5 + x4 - x4 + x3 - x3 + x2 - x2 + x + 34
A= x + 34⇒71+34=105
CHÚC BẠN HỌC TỐT ^.^
HS
1
22 tháng 8 2017
Ta có: x= 71 => x-1=70
Thay x-1=70 vào BT A ta được:
A= x5 - ( x-1) x4 -....- ( x-1 ) x + 34
A= x5 - x5 +x4 -...- x2+ x +34
A= x + 34
Thay x=71 vào BT A ta được :
A= 71 + 34 = 105