
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a)
2x2+2x+1
=(x+1)2+x2
(x+1)2 luôn lớn hơn hoặc =0
dấu "=" xảy ra khi x=-1. mà với x=-1 thì x2=1 => biểu thức trên =1
x2 luôn lớn hơn hoặc =0
dấu "=" xảy ra khi x=0=> (x+1)2=1 => biểu thức trên =1
vậy biểu thức này có giá trị dương ( >0 ) với mọi giá trị của biến
b)9x2-6x+2
=(3x+1)2 +1
ta có: (3x+1)2 luôn lớ hơn hoặc =0
=> (3x+1)2+1 luôn lớn hơn hoặc =1
=> (3x+1)^2+1 luôn dương với mọi giá trị của biến
a) \(2x^2+2x+1=2\left(x^2+x+\frac{1}{2}\right)=2\left[\left(x+\frac{1}{2}\right)^2+\frac{1}{4}\right]=\frac{1}{2}+2\left(x+\frac{1}{2}\right)^2\)
Vì: \(2\left(x+\frac{1}{2}\right)^2\ge0\) với mọi x
=> \(\frac{1}{2}+2\left(x+\frac{1}{2}\right)^2>0\)
Vậy biểu thức trên luôn luôn dương với mọi giá trị của biến
b) \(9x^2-6x+2=9x^2-6x+1+1=\left(3x-1\right)^2+1\)
Vì: \(\left(3x-1\right)^2\ge0\) với mọi giá trị của x
=> \(\left(3x-1\right)^2+1>0\)
vậy biểu thức trên luôn luôn dương với mọi giá trị của x

a:
ĐKXĐ: \(\left\{{}\begin{matrix}a>0\\a\ne4\end{matrix}\right.\)
\(A=\left(\dfrac{\sqrt{a}}{\sqrt{a}-2}+\dfrac{\sqrt{a}}{\sqrt{a}+2}\right)\cdot\dfrac{a-4}{\sqrt{4a}}\)
\(=\dfrac{\sqrt{a}\left(\sqrt{a}+2\right)+\sqrt{a}\left(\sqrt{a}-2\right)}{\left(\sqrt{a}+2\right)\left(\sqrt{a}-2\right)}\cdot\dfrac{a-4}{2\sqrt{a}}\)
\(=\dfrac{a+2\sqrt{a}+a-2\sqrt{a}}{a-4}\cdot\dfrac{a-4}{2\sqrt{a}}=\dfrac{2a}{2\sqrt{a}}=\sqrt{a}\)
b: A-2<0
=>\(\sqrt{a}-2< 0\)
=>\(\sqrt{a}< 2\)
=>0<=a<4
kết hợp ĐKXĐ, ta được: 0<a<4
c: Để \(\dfrac{4}{A+1}=\dfrac{4}{\sqrt{a}+1}\) là số nguyên thì
\(\sqrt{a}+1\inƯ\left(4\right)\)
=>\(\sqrt{a}+1\in\left\{1;-1;2;-2;4;-4\right\}\)
=>\(\sqrt{a}\in\left\{0;-2;1;-3;3;-5\right\}\)
=>\(\sqrt{a}\in\left\{0;1;3\right\}\)
=>\(a\in\left\{0;1;9\right\}\)
Kết hợp ĐKXĐ, ta được: \(a\in\left\{1;9\right\}\)
a) \(A=\left(\dfrac{\sqrt{a}}{\sqrt{a}-2}+\dfrac{\sqrt{a}}{\sqrt{a}+2}\right)\cdot\dfrac{a-4}{\sqrt{4a}}\left(dkxd:a\ge0;a\ne4\right)\)
\(=\left[\dfrac{\sqrt{a}\left(\sqrt{a}+2\right)}{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}+\dfrac{\sqrt{a}\left(\sqrt{a}-2\right)}{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}\right]\cdot\dfrac{a-4}{2\sqrt{a}}\)
\(=\dfrac{a+2\sqrt{a}+a-2\sqrt{a}}{a-4}\cdot\dfrac{a-4}{2\sqrt{a}}\)
\(=\dfrac{2a}{2\sqrt{a}}\)
\(=\sqrt{a}\)
b) Để \(A-2< 0\) thì: \(\sqrt{a}-2< 0\)
\(\Rightarrow\sqrt{a}< 2\)
\(\Rightarrow a< 4\)
Kết hợp với điều kiện xác định của \(a\), ta được: \(0\le a< 4\)
c) Để \(\dfrac{4}{A+1}\) nguyên thì \(\dfrac{4}{\sqrt{a}+1}\) nguyên
\(\Rightarrow4⋮\sqrt{a}+1\)
\(\Rightarrow\sqrt{a}+1\inƯ\left(4\right)\)
Mà \(\sqrt{a}+1\ge1\forall a\ge0;a\ne4\)
\(\Rightarrow\sqrt{a}+1\in\left\{1;2;4\right\}\)
\(\Rightarrow\sqrt{a}\in\left\{0;1;3\right\}\)
\(\Rightarrow a\in\left\{0;1;9\right\}\)
Kết hợp với điều kiện xác định của \(a\), ta được: \(a\in\left\{0;1;9\right\}\)
\(\text{#}Toru\)

a) \(B=\dfrac{x-\sqrt{x}+1}{\sqrt{x}-1}=\dfrac{9-\sqrt{9}+1}{\sqrt{9}-1}=\dfrac{9-3+1}{3-1}=\dfrac{7}{2}\)
b) \(A=\dfrac{\left(\sqrt{x}+1\right)\left(\sqrt{x}+3\right)+2\left(\sqrt{x}-2\right)-9\sqrt{x}+3}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{x-3\sqrt{x}+2}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}=\dfrac{\left(\sqrt{x}-1\right)\left(\sqrt{x}-2\right)}{\left(\sqrt{x}-2\right)\left(\sqrt{x}+3\right)}=\dfrac{\sqrt{x}-1}{\sqrt{x}+3}\)
c) \(A=\dfrac{\sqrt{x}-1}{\sqrt{x}+3}>0\Leftrightarrow\sqrt{x}-1>0\left(do.\sqrt{x}+3>0\right)\)
\(\Leftrightarrow\sqrt{x}>1\Leftrightarrow x>1\)
\(B=\dfrac{x-\sqrt{x}+1}{\sqrt{x}-1}=\dfrac{\sqrt{x}\left(\sqrt{x}-1\right)+1}{\sqrt{x}-1}=\sqrt{x}+\dfrac{1}{\sqrt{x}-1}\)
Do \(\sqrt{x}>1\Leftrightarrow\sqrt{x}-1>0\)
Áp dụng BĐT Cauchy cho 2 số k âm:
\(B=\sqrt{x}-1+\dfrac{1}{\sqrt{x}-1}+1\ge2\sqrt{\left(\sqrt{x}-1\right).\dfrac{1}{\sqrt{x}-1}}+1=2+1=3\)
Dấu "=" xảy ra \(\Leftrightarrow\left(\sqrt{x}-1\right)^2=1\Leftrightarrow x=4\)

Câu 1:
a: \(A=15\sqrt{4a}+\sqrt{a}-\sqrt{25a}\)
\(=15\cdot2\sqrt{a}+\sqrt{a}-5\sqrt{a}\)
\(=30\sqrt{a}-4\sqrt{a}=26\sqrt{a}\)
b: Sửa đề: Khi a=100
Thay a=100 vào A, ta được:
\(A=26\cdot\sqrt{100}=26\cdot10=260\)

a: \(A=\dfrac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\left(\sqrt{a}-1\right)\left(\sqrt{a}-2\right)}{a-1-a+4}\)
\(=\dfrac{\sqrt{a}-2}{3\sqrt{a}}\)
\(ĐK:a>0;a\ne1;a\ne4\\ a,A=\dfrac{\sqrt{a}-\sqrt{a}+1}{\sqrt{a}\left(\sqrt{a}-1\right)}:\dfrac{a-1-a+4}{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}=\dfrac{1}{\sqrt{a}\left(\sqrt{a}-1\right)}\cdot\dfrac{\left(\sqrt{a}-2\right)\left(\sqrt{a}-1\right)}{3}=\dfrac{\sqrt{a}-2}{3\sqrt{a}}\\ b,A>0\Leftrightarrow\sqrt{a}-2>0\Leftrightarrow a>4\)

Ta có:
\(A=x-\left(\frac{1}{\sqrt{x}-\sqrt{x-1}}-\frac{1}{\sqrt{x}+\sqrt{x-1}}\right)\)
\(A=x-\frac{\sqrt{x}+\sqrt{x-1}-\sqrt{x}+\sqrt{x-1}}{\left(\sqrt{x}-\sqrt{x-1}\right)\left(\sqrt{x}+\sqrt{x-1}\right)}\)
\(A=x-\frac{2\sqrt{x-1}}{x-x+1}\)
\(A=x-2\sqrt{x-1}\)
\(A=\left(x-1\right)-2\sqrt{x-1}+1\)
\(A=\left(\sqrt{x-1}-1\right)^2\ge0\left(\forall x\ge1\right)\)
=> đpcm

a: ĐKXĐ: \(\left\{{}\begin{matrix}a>0\\a\ne4\end{matrix}\right.\)
\(A=\left(\dfrac{\sqrt{a}}{\sqrt{a}-2}+\dfrac{\sqrt{a}}{\sqrt{a}-2}\right)\cdot\dfrac{a-4}{\sqrt{4a}}\)
\(=\dfrac{2\sqrt{a}}{\sqrt{a}-2}\cdot\dfrac{\left(\sqrt{a}-2\right)\left(\sqrt{a}+2\right)}{2a}\)
\(=\sqrt{a}+2\)
b: A-2<0
=>\(\sqrt{a}+2-2< 0\)
=>\(\sqrt{a}< 0\)
=>\(a\in\varnothing\)
c: Bạn ghi đầy đủ đề đi bạn

a: ta có: \(M=\dfrac{a}{\sqrt{ab}+b}+\dfrac{b}{\sqrt{ab}-a}-\dfrac{a+b}{\sqrt{ab}}\)
\(=\dfrac{a\left(\sqrt{ab}-a\right)+b\left(\sqrt{ab}+b\right)}{\left(\sqrt{ab}+b\right)\left(\sqrt{ab}-a\right)}-\dfrac{a+b}{\sqrt{ab}}\)
\(=\dfrac{-\sqrt{ab}\left(a+b\right)+\left(a-b\right)\left(a+b\right)}{\sqrt{b}\left(\sqrt{a}+\sqrt{b}\right)\cdot\sqrt{a}\cdot\left(\sqrt{a}-\sqrt{b}\right)}-\dfrac{a+b}{\sqrt{ab}}\)
\(=\dfrac{-\sqrt{ab}\left(a+b\right)+\left(a-b\right)\left(a+b\right)}{\sqrt{ab}\left(a-b\right)}-\dfrac{a^2-b^2}{\sqrt{ab}\left(a-b\right)}\)
\(=\dfrac{-\sqrt{ab}}{\sqrt{ab}\left(a-b\right)}\)
\(=-\dfrac{1}{a-b}\)
b: Thay \(a=\sqrt{5}+1\) và \(b=\sqrt{5}-1\) vào M, ta được:
\(M=\dfrac{-1}{\sqrt{5}+1-\sqrt{5}+1}=\dfrac{-1}{2}\)
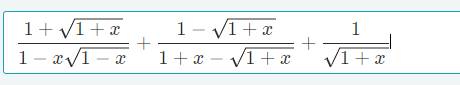
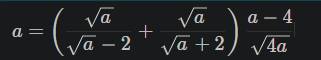 với a ≥ 0, a ≠ 4
với a ≥ 0, a ≠ 4