K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
CM
31 tháng 1 2019
a) Đa thức thương x 2 – 6x + 9.
b) Đa thức thương 2 x 2 – 5.
c) Đa thức thương x 2 + 4x + 3 và đa thức dư -12.
d) Đa thức x + 5 và đa thức dư x – 4.
8 tháng 1 2022
a: \(=6x^3-10x^2+6x\)
b: \(=-2x^4-10x^3+6x^2\)
c: \(=-x^5+2x^3-\dfrac{3}{2}x^2\)
d: \(=2x^3+10x^2-8x-x^2-5x+4=2x^3+9x^2-13x+4\)
PN
22 tháng 5 2021
\(\left(1-x\right)\left(5x+3\right)=\left(3x-7\right)\left(x-1\right)\)
\(< =>\left(1-x\right)\left(5x+3+3x-7\right)=0\)
\(< =>\left(1-x\right)\left(8x-4\right)=0\)
\(< =>\orbr{\begin{cases}1-x=0\\8x-4=0\end{cases}< =>\orbr{\begin{cases}x=1\\x=\frac{1}{2}\end{cases}}}\)
PN
22 tháng 5 2021
\(\left(x-2\right)\left(x+1\right)=x^2-4\)
\(< =>\left(x-2\right)\left(x+1\right)=\left(x-2\right)\left(x+2\right)\)
\(< =>\left(x-2\right)\left(x+1-x-2\right)=0\)
\(< =>-1\left(x-2\right)=0\)
\(< =>2-x=0< =>x=2\)
x2 ( 2x3 – 4x + 3)
24 tháng 12 2021
a: \(=15x^5-25x^4+15x^3\)
b: \(=2x^3+10x^2-8x-x^2-5x+4\)
\(=2x^3+9x^2-13x+4\)
H9
HT.Phong (9A5)
CTVHS
12 tháng 8 2023
a) \(\left(2x^3-x^2+5x\right):x\)
\(=\dfrac{2x^3-x^2+5x}{x}\)
\(=\dfrac{x\left(2x^2-x+5\right)}{x}\)
\(=2x^2-x+5\)
b) \(\left(3x^4-2x^3+x^2\right):\left(-2x\right)\)
\(=\dfrac{3x^4-2x^3+x^2}{-2x}\)
\(=\dfrac{2x\left(\dfrac{3}{2}x^3-x^2+\dfrac{1}{2}x\right)}{-2x}\)
\(=-\left(\dfrac{3}{2}x^3-x^2+\dfrac{1}{2}x\right)\)
\(=-\dfrac{3}{2}x^3+x^2-\dfrac{1}{2}x\)
c) \(\left(-2x^5+3x^2-4x^3\right):2x^2\)
\(=\dfrac{-2x^5+3x^2-4x^3}{2x^2}\)
\(=\dfrac{2x^2\left(-x^3+\dfrac{3}{2}-2x\right)}{2x^2}\)
\(=-x^3-2x+\dfrac{3}{2}\)
H9
HT.Phong (9A5)
CTVHS
12 tháng 8 2023
d) \(\left(x^3-2x^2y+3xy^2\right):\left(-\dfrac{1}{2}x\right)\)
\(=\dfrac{x^3-2x^2y+3xy^2}{-\dfrac{1}{2}x}\)
\(=\dfrac{\dfrac{1}{2}x\left(2x^2-4xy+6y^2\right)}{-\dfrac{1}{2}x}\)
\(=-\left(2x^2-4xy+6y^2\right)\)
\(=-2x^2+4xy-6y^2\)
e) \(\left[3\left(x-y\right)^5-2\left(x-y\right)^4+3\left(x-y\right)^2\right]:5\left(x-y\right)^2\)
\(=\dfrac{3\left(x-y\right)^5-2\left(x-y\right)^4+3\left(x-y\right)^2}{5\left(x-y\right)^2}\)
\(=\dfrac{5\left(x-y\right)^2\left[\dfrac{3}{5}\left(x-y\right)^3-\dfrac{2}{5}\left(x-y\right)^2+\dfrac{3}{5}\right]}{5\left(x-y\right)^2}\)
\(=\dfrac{3}{5}\left(x-y\right)^3-\dfrac{2}{5}\left(x-y\right)^2+\dfrac{3}{5}\)
f) \(\left(3x^5y^2+4x^3y^3-5x^2y^4\right):2x^2y^2\)
\(=\dfrac{3x^5y^2+4x^3y^3-5x^2y^4}{2x^2y^2}\)
\(=\dfrac{2x^2y^2\left(\dfrac{3}{2}x^3+2xy-\dfrac{5}{2}y^2\right)}{2x^2y^2}\)
\(=\dfrac{3}{2}x^3+2xy-\dfrac{5}{2}y^2\)
4 tháng 1 2022
a: \(=\dfrac{x^3\left(2x-1\right)+2\left(2x-1\right)}{2x-1}=x^3+2\)
b: \(=\dfrac{2x^3-4x^2+3x^2-6x+x-2}{x-2}=2x^2+3x+1\)
d: \(=\dfrac{x^4-2x^3+3x^2+2x^3-4x^2+6x-x^2+2x-3}{x^2-2x+3}=x^2+2x-1\)
9 tháng 10 2021
a)=\(3x^3-15x^2+21x\)
b)\(=-2x^4y-10x^2y+2xy\)
c)\(=-x^3+6x^2+5x-4x^2+24x+20=-x^3+2x^2+29x+20\)
d)\(=2x^4-3x^3+4x^2-2x^2+3x-4=2x^4-3x^32x^2+3x-4\)
e)\(=x^2-4y^2\)
f)\(=-2x^2y^3+y-3\)
g)\(=3xy^4-\dfrac{1}{2}y^2+2x^2y\)
h)\(=9x^2-6x+1-7x^2-14=2x^2-6x-13\)
i)\(=x^2-x-3\)
j)\(=\left(x+2y\right)\left(x^2-2y+4y^2\right):\left(x+2y\right)=x^2-2y+4y^2\)
27 tháng 10 2021
a: \(=\dfrac{\left(x^2+5\right)\left(x^2-5\right)+2x\left(x^2+5\right)}{x^2+5}=x^2+2x-5\)
b: \(=\dfrac{x^3-2x^2-x^2+2x+3x-6}{x-2}=x^2-x+3\)
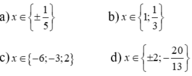
chia theo cột ta được 2x-1 và dư là 4x