
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(2^x.3^{x-1}.5^{x+2}=12\\ \Leftrightarrow2^x.\frac{3^x}{3}.\left(5^x.25\right)=12\\ \Leftrightarrow\left(2.3.5\right)^x=\frac{36}{25}\\ \Leftrightarrow30^x=\frac{36}{25}\\\Leftrightarrow x=log_{30}\left(\frac{36}{25}\right)\)
Bạn chép đề có đúng không? Nếu sửa lại đề bài 1 tý thì nghiệm sẽ đẹp hơn
\(2^x.3^{x-1}.5^{x-2}=12\\ \Leftrightarrow2^x.\frac{3^x}{3}.\frac{5^x}{25}=12\\ \Leftrightarrow\left(2.3.5\right)^x=900\\ \Leftrightarrow30^x=900\Leftrightarrow x=2\)



\(5\left(x^2+y^2+z^2\right)=9\left(xy+2yz+zx\right)\\ \Leftrightarrow5x^2+5\left(y^2+z^2\right)-9x\left(y+z\right)-18yz=0\\ \Leftrightarrow5x^2-9x\left(y+z\right)=18yz-5\left(y^2+z^2\right)\)
Ta có \(yz\le\dfrac{\left(y+z\right)^2}{4};y^2+z^2\ge\dfrac{\left(y+z\right)^2}{2}\)
\(\Leftrightarrow18yz-5\left(y^2+z^2\right)\le\dfrac{9\left(y+z\right)^2}{2}-\dfrac{5\left(y+z\right)^2}{2}=2\left(y+z\right)^2\\ \Leftrightarrow5x^2-9x\left(y+z\right)\le2\left(y+z\right)^2\\ \Leftrightarrow5x^2-9x\left(y+z\right)-2\left(y+z\right)^2\le0\\ \Leftrightarrow\left[x-2\left(y+z\right)\right]\left(5x+y+z\right)\le0\\ \Leftrightarrow x-2\left(y+z\right)\le0\left(x,y,z>0\right)\\ \Leftrightarrow x\le2\left(y+z\right)\)
Ta có \(P=\dfrac{x}{y^2+z^2}-\dfrac{1}{\left(x+y+z\right)^3}\le\dfrac{x}{\dfrac{\left(y+z\right)^2}{2}}-\dfrac{1}{\left(x+y+z\right)^3}\)
\(\Leftrightarrow P\le\dfrac{2x}{\left(y+z\right)^2}-\dfrac{1}{\left[2\left(y+z\right)+y+z\right]^3}\le\dfrac{4\left(y+z\right)}{\left(y+z\right)^2}-\dfrac{1}{27\left(y+z\right)^3}\\ \Leftrightarrow P\le\dfrac{4}{y+z}-\dfrac{1}{27\left(y+z\right)^3}\)
Đặt \(t=\dfrac{1}{y+z}>0\Leftrightarrow P\le4t-\dfrac{t^3}{27}\)
\(\Leftrightarrow16-P\ge16-4t+\dfrac{t^3}{27}=\dfrac{t^3-108t+432}{27}\\ \Leftrightarrow16-P\ge\dfrac{\left(t+12\right)\left(t-6\right)^2}{27}\ge0\left(t>0\right)\\ \Leftrightarrow P\le16\)
Dấu \("="\Leftrightarrow x=\dfrac{1}{3};y=z=\dfrac{1}{12}\)

\(I=\int\dfrac{2}{2+5sinxcosx}dx=\int\dfrac{2sec^2x}{2sec^2x+5tanx}dx\\ =\int\dfrac{2sec^2x}{2tan^2x+5tanx+2}dx\)
We substitute :
\(u=tanx,du=sec^2xdx\\ I=\int\dfrac{2}{2u^2+5u+2}du\\ =\int\dfrac{2}{2\left(u+\dfrac{5}{4}\right)^2-\dfrac{9}{8}}du\\ =\int\dfrac{1}{\left(u+\dfrac{5}{4}\right)^2-\dfrac{9}{16}}du\\ \)
Then,
\(t=u+\dfrac{5}{4}\\I=\int\dfrac{1}{t^2-\dfrac{9}{16}}dt\\ =\int\dfrac{\dfrac{2}{3}}{t-\dfrac{3}{4}}-\dfrac{\dfrac{2}{3}}{t+\dfrac{3}{4}}dt\)
Finally,
\(I=\dfrac{2}{3}ln\left(\left|\dfrac{t-\dfrac{3}{4}}{t+\dfrac{3}{4}}\right|\right)+C=\dfrac{2}{3}ln\left(\left|\dfrac{tanx+\dfrac{1}{2}}{tanx+2}\right|\right)+C\)

Đề bài bị sai
Gọi H là trung điểm AB thì \(SH\perp\left(ABCD\right)\Rightarrow\widehat{SCH}=60^0\)
\(\Rightarrow CH=\dfrac{SH}{tan60^0}=\dfrac{SH}{\sqrt{3}}\)
Mặt khác tam giác SAB đều \(\Rightarrow\widehat{SBH}=60^0\Rightarrow BH=\dfrac{SH}{tan60^0}=\dfrac{SH}{\sqrt{3}}\)
\(\Rightarrow CH=BH\) (vô lý do tam giác BCH vuông tại B theo giả thiết. Mà CH là cạnh huyền, BH là cạnh góc vuông, 2 cạnh này không thể bằng nhau)
 giúp mk với :(((((((((((
giúp mk với :(((((((((((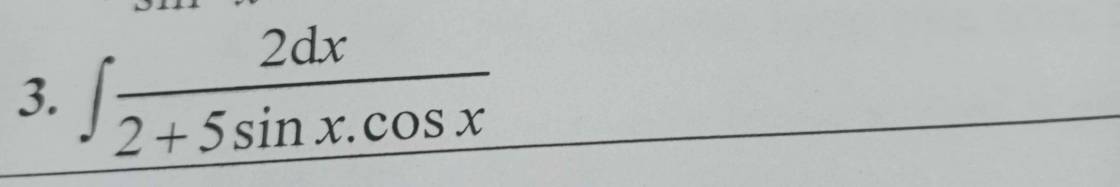

3 nhó cái cho mk
1+2=3
đúng luôn còn gì :)))