Trong mặt phẳng Oxy, cho tam giác MNP có M(1,-1),N(5,-3) và P là điểm thuộc trục Oy, trọng tâm G của tam giác MNP nằm trên trục Ox. Tọa độ điểm P là
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
3 tháng 1 2022
Do C thuộc Ox nên tọa độ có dạng: \(C\left(x;0\right)\)
Do trọng tâm G thuộc Oy \(\Rightarrow x_G=0\)
Mà \(x_A+x_B+x_C=3x_G\)
\(\Rightarrow1+\left(-3\right)+x=3.0\)
\(\Rightarrow x=2\)
\(\Rightarrow C\left(2;0\right)\)
NV
Nguyễn Việt Lâm
Giáo viên
26 tháng 12 2022
Do P thuộc Ox nên tọa độ có dạng \(P\left(p;0\right)\)
\(\Rightarrow\left\{{}\begin{matrix}\overrightarrow{MN}=\left(1;-3\right)\\\overrightarrow{MP}=\left(p-2;-1\right)\end{matrix}\right.\)
Do tam giác MNP vuông tại M \(\Rightarrow\overrightarrow{MN}.\overrightarrow{MP}=0\)
\(\Rightarrow1.\left(p-2\right)+3=0\) \(\Rightarrow p=-1\)
\(\Rightarrow P\left(-1;0\right)\)
\(\Rightarrow\overrightarrow{MP}=\left(-3;-1\right)\Rightarrow\left\{{}\begin{matrix}MN=\sqrt{1^2+\left(-3\right)^2}=\sqrt{10}\\MP=\sqrt{\left(-3\right)^2+\left(-1\right)^2}=\sqrt{10}\end{matrix}\right.\)
\(\Rightarrow S_{MNP}=\dfrac{1}{2}MN.MP=5\)
CM
24 tháng 4 2018
Tọa độ trọng tâm G của tam gác MNP là:
x G = x M + x N + x P 3 = 0 + ( − 3 ) + 9 3 = 2 y G = y M + y N + y P 3 = 4 + 2 + ( − 3 ) 3 = 1 ⇒ G ( 2 ; 1 )
Đáp án D
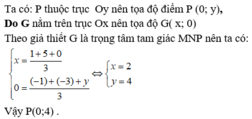
Gọi tọa độ \(P\left(0;a\right)\) và \(G\left(b;0\right)\)
Theo công thức trọng tâm:
\(\left\{{}\begin{matrix}x_G=\frac{x_M+x_N+x_P}{3}\\y_G=\frac{y_M+y_N+y_P}{3}\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}3b=1+5+0\\3.0=-1+\left(-3\right)+a\end{matrix}\right.\) \(\Rightarrow\left\{{}\begin{matrix}b=2\\a=4\end{matrix}\right.\) \(\Rightarrow P\left(0;4\right)\)
Hay