Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(x^2-2x+5=x^2-2x+1+4=\left(x-1\right)^2+4\ge4\)
\(\sqrt{\left(x-1\right)^2+4}\ge2\)
\(\sqrt{x^2-2x+5}\ge2\)

Ta có:
\(C=\sqrt{-x^2+6x}\)
Mà: \(\sqrt{-x^2+6x}\ge0\)
Dấu "=" xảy ra khi:
\(\sqrt{-x^2+6x}=0\)
\(\Leftrightarrow\sqrt{-x\left(x-6\right)}=0\)
\(\Leftrightarrow-x\left(x-6\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=6\end{matrix}\right.\)
Vậy: \(C_{min}=0\) khi \(\left[{}\begin{matrix}x=0\\x=6\end{matrix}\right.\)
\(D=\sqrt{6x-2x^2}\)
Mà: \(\sqrt{6x-2x^2}\ge0\)
Dấu "=" xảy ra khi:
\(\sqrt{6x-2x^2}=0\)
\(\Leftrightarrow\sqrt{2x\left(3-x\right)}=0\)
\(\Leftrightarrow2x\left(3-x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=3\end{matrix}\right.\)
Vậy: \(D_{min}=0\) khi \(\left[{}\begin{matrix}x=0\\x=3\end{matrix}\right.\)

a . ta có : \(1\le1+\sqrt{2-x}\Rightarrow GTNN=1\)
\(-2\le\sqrt{x-3}-2\Rightarrow GTNN=-2\)
b. \(0\le\sqrt{4-x^2}\le2\)
\(\sqrt{2x^2-x+3}=\sqrt{2\left(x^2-\frac{x}{2}+\frac{1}{16}\right)+\frac{23}{8}}=\sqrt{2\left(x-\frac{1}{4}\right)^2+\frac{23}{8}}\ge\frac{\sqrt{46}}{4}\)
vậy \(GTNN=\frac{\sqrt{46}}{4}\)
ta có : \(0\le-x^2+2x+5=-\left(x-1\right)^2+6\le6\)
\(\Rightarrow1-\sqrt{6}\le1-\sqrt{-x^2+2x+5}\le1\)Vậy \(\hept{\begin{cases}GTNN=1-\sqrt{6}\\GTLN=1\end{cases}}\)

1:
a: \(A=\dfrac{\sqrt{x}+1-2}{\sqrt{x}+1}=1-\dfrac{2}{\sqrt{x}+1}\)
căn x+1>=1
=>2/căn x+1<=2
=>-2/căn x+1>=-2
=>A>=-2+1=-1
Dấu = xảy ra khi x=0
b: 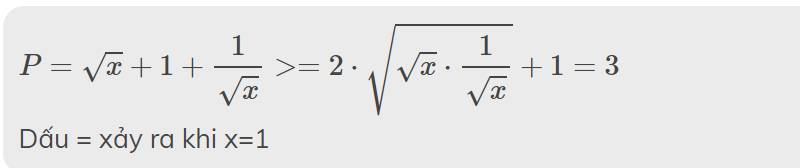

toan violympic lop 9 la GTNN
\(B=\sqrt{x^2-6x+2y^2+4y+20}+\sqrt{x^2+2x+5}\)
\(=\sqrt{\left(x-3\right)^2+2\left(y+1\right)^2+9}+\sqrt{\left(x+1\right)^2+4}\ge\sqrt{9}+\sqrt{4}=5\)
tick nha
\(B=\sqrt{\left(3-x\right)^2+2\left(y+1\right)^2+3^2}+\sqrt{\left(x+2\right)^2+2^2}\ge\sqrt{\left(3-x+x+2\right)^2+\left(3+2\right)^2}=5\sqrt{2}\)
Bmin = \(5\sqrt{2}\) khi x=0 ; y =-1
B min nhé

Tìm đc mỗi GTNN, cách tìm GTLN chưa chắc chắn lắm nên mk ko lm nha :D
1/ \(A=\sqrt{\left(x-1\right)^2}+\sqrt{\left(3-x\right)^2}=\left|x-1\right|+\left|3-x\right|\ge\left|x-1+3-x\right|=2\)
2/ \(B=\sqrt{x-1-2\sqrt{x-1}+1}+\sqrt{x-1+2\sqrt{x-1}+1}=\sqrt{\left(1-\sqrt{x-1}\right)^2}+\sqrt{\left(\sqrt{x-1}+1\right)^2}\)
\(=\left|1-\sqrt{x-1}\right|+\left|\sqrt{x-1}+1\right|\ge\left|1-\sqrt{x-1}+\sqrt{x-1}+1\right|=2\)
a) \(A=\sqrt[]{x^2-2x+5}\)
\(\Leftrightarrow A=\sqrt[]{x^2-2x+1+4}\)
\(\Leftrightarrow A=\sqrt[]{\left(x+1\right)^2+4}\)
mà \(\left(x+1\right)^2\ge0,\forall x\in R\)
\(A=\sqrt[]{\left(x+1\right)^2+4}\ge\sqrt[]{4}=2\)
Dấu "=" xảy ra khi và chỉ khi \(x+1=0\Leftrightarrow x=-1\)
Vậy \(GTNN\left(A\right)=2\left(khi.x=-1\right)\)
b) \(B=5-\sqrt[]{x^2-6x+14}\)
\(\Leftrightarrow B=5-\sqrt[]{x^2-6x+9+5}\)
\(\Leftrightarrow B=5-\sqrt[]{\left(x-3\right)^2+5}\left(1\right)\)
Ta có : \(\left(x-3\right)^2\ge0,\forall x\in R\)
\(\Leftrightarrow\left(x-3\right)^2+5\ge5,\forall x\in R\)
\(\Leftrightarrow\sqrt[]{\left(x-3\right)^2+5}\ge\sqrt[]{5},\forall x\in R\)
\(\Leftrightarrow-\sqrt[]{\left(x-3\right)^2+5}\le-\sqrt[]{5},\forall x\in R\)
\(\Leftrightarrow B=5-\sqrt[]{\left(x-3\right)^2+5}\le5-\sqrt[]{5},\forall x\in R\)
Dấu "=" xả ra khi và chỉ khi \(x-3=0\Leftrightarrow x=3\)
Vậy \(GTLN\left(B\right)=5-\sqrt[]{5}\left(khi.x=3\right)\)