Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

\(y'=4mx^3+2mx=2mx\left(2x^2+1\right)\)
Do \(2x\left(x^2+1\right)>0\) ;\(\forall x>0\)
\(\Rightarrow y'\ge0\) ;\(\forall x>0\) khi và chỉ khi \(m>0\)

\(y'=\dfrac{-m^2-1}{\left(x-m\right)^2}\)
\(y'< 0\) ;\(\forall x\in\left(0;1\right)\Leftrightarrow\left[{}\begin{matrix}m\ge1\\m\le0\end{matrix}\right.\)

3.
Hàm trùng phương \(f\left(x\right)=ax^4+bx^2+c\) với \(a\ne0\) đồng biến trên \(\left(0;+\infty\right)\) khi và chỉ khi:
\(\left\{{}\begin{matrix}a>0\\b\ge0\end{matrix}\right.\) \(\Leftrightarrow m\ge0\)
Hoặc giải bt: \(y'=4x^3+2mx\ge0\) ;\(\forall x>0\)
\(\Leftrightarrow2x\left(x^2+m\right)\ge0\)
\(\Leftrightarrow x^2+m\ge0\)
\(\Leftrightarrow x^2\ge-m\)
\(\Leftrightarrow-m\le min\left(x^2\right)=0\Rightarrow m\ge0\)
1.
Giả sử tiếp tuyến d có 1 vtpt là \(\left(a;b\right)\) với \(a^2+b^2>0\)
\(\Rightarrow cos30^0=\frac{\sqrt{3}}{2}=\frac{\left|a-2b\right|}{\sqrt{\left(a^2+b^2\right)\left(1^2+\left(-2\right)^2\right)}}=\frac{\left|a-2b\right|}{\sqrt{5\left(a^2+b^2\right)}}\)
\(\Leftrightarrow4\left(a-2b\right)^2=15\left(a^2+b^2\right)\)
\(\Leftrightarrow11a^2+16ab-b^2=0\)
Nghiệm xấu quá nhìn muốn nản, bạn tự làm tiếp :)
2.
\(y'=cosx-2sinx+2m-5\)
Hàm số đồng biến trên TXĐ khi và chỉ khi \(y'\ge0\) ; \(\forall x\)
\(\Leftrightarrow cosx-2sinx+2m-5\ge0\) ;\(\forall x\)
\(\Leftrightarrow2m-5\ge2sinx-cosx\)
\(\Leftrightarrow2m-5\ge f\left(x\right)_{max}\) với \(f\left(x\right)=2sinx-cosx\)
Ta có: \(f\left(x\right)=2sinx-cosx=\sqrt{5}\left(\frac{2}{\sqrt{5}}sinx-\frac{1}{\sqrt{5}}cosx\right)=\sqrt{5}sin\left(x-a\right)\)
Với \(a\in\left(0;\pi\right)\) sao cho \(cosa=\frac{2}{\sqrt{5}}\)
\(\Rightarrow f\left(x\right)\le\sqrt{5}\Rightarrow2m-5\ge\sqrt{5}\Rightarrow m\ge\frac{5+\sqrt{5}}{2}\)

a/ \(y'=3mx^2-2\left(m+1\right)x+3m\)
Xet m=0 ko thoa man
Xet m khac 0
\(y'\ge0\Leftrightarrow\left(m+1\right)^2-9m^2\le0\Leftrightarrow8m^2-2m-1\ge0\)
\(\Leftrightarrow m^2+8\le0\left(vl\right)\) => ko ton tai m thoa man
b/ \(y'=mx^2-2mx+2m-1\)
m=0 ko thoa man
Xet m khac 0
\(y'\ge0\Leftrightarrow\left\{{}\begin{matrix}m>0\\m^2-m\left(2m-1\right)\le0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m>0\\m^2-m\ge0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}m>0\\\left[{}\begin{matrix}m\ge1\\m\le0\end{matrix}\right.\end{matrix}\right.\Leftrightarrow m\ge1\)

3.
\(x-2y+1=0\Leftrightarrow y=\frac{1}{2}x+\frac{1}{2}\)
\(y'=\frac{2}{\left(x+1\right)^2}\Rightarrow\frac{2}{\left(x+1\right)^2}=\frac{1}{2}\)
\(\Rightarrow\left(x+1\right)^2=4\Rightarrow\left[{}\begin{matrix}x=1\Rightarrow y=1\\x=-3\Rightarrow y=3\end{matrix}\right.\)
Có 2 tiếp tuyến: \(\left[{}\begin{matrix}y=\frac{1}{2}\left(x-1\right)+1\\y=\frac{1}{2}\left(x+3\right)+3\end{matrix}\right.\) \(\Leftrightarrow\left[{}\begin{matrix}y=\frac{1}{2}x+\frac{1}{2}\left(l\right)\\y=\frac{1}{2}x+\frac{9}{2}\end{matrix}\right.\)
4.
\(\lim\limits\frac{\sqrt{2n^2+1}-3n}{n+2}=\lim\limits\frac{\sqrt{2+\frac{1}{n^2}}-3}{1+\frac{2}{n}}=\sqrt{2}-3\)
\(\Rightarrow\left\{{}\begin{matrix}a=2\\b=3\end{matrix}\right.\)
5.
\(\lim\limits_{x\rightarrow a}\frac{2\left(x^2-a^2\right)+a\left(a+1\right)-\left(a+1\right)x}{\left(x-a\right)\left(x+a\right)}=\lim\limits_{x\rightarrow a}\frac{\left(x-a\right)\left(2x+2a\right)-\left(a+1\right)\left(x-a\right)}{\left(x-a\right)\left(x+a\right)}\)
\(=\lim\limits_{x\rightarrow a}\frac{\left(x-a\right)\left(2x+a-1\right)}{\left(x-a\right)\left(x+a\right)}=\lim\limits_{x\rightarrow a}\frac{2x+a-1}{x+a}=\frac{3a-1}{2a}\)
1.
\(f'\left(x\right)=-3x^2+6mx-12=3\left(-x^2+2mx-4\right)=3g\left(x\right)\)
Để \(f'\left(x\right)\le0\) \(\forall x\in R\) \(\Leftrightarrow g\left(x\right)\le0;\forall x\in R\)
\(\Leftrightarrow\Delta'=m^2-4\le0\Rightarrow-2\le m\le2\)
\(\Rightarrow m=\left\{-1;0;1;2\right\}\)
2.
\(f'\left(x\right)=\frac{m^2-20}{\left(2x+m\right)^2}\)
Để \(f'\left(x\right)< 0;\forall x\in\left(0;2\right)\)
\(\Leftrightarrow\left\{{}\begin{matrix}m^2-20< 0\\\left[{}\begin{matrix}m>0\\m< -4\end{matrix}\right.\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}-\sqrt{20}< m< \sqrt{20}\\\left[{}\begin{matrix}m>0\\m< -4\end{matrix}\right.\end{matrix}\right.\)
\(\Rightarrow m=\left\{1;2;3;4\right\}\)

Do \(3+sinx+cosx=3+\sqrt{2}sin\left(x+\frac{\pi}{4}\right)\ge3-\sqrt{2}>0;\forall x\)
Nên BPT đã cho tương đương:
\(mcosx+m-1< 3+sinx+cosx\) ;\(\forall x\)
\(\Leftrightarrow\left(m-1\right)cosx-sinx< 4-m\)
\(\Leftrightarrow\frac{m-1}{\sqrt{\left(m-1\right)^2+1}}cosx-\frac{1}{\sqrt{\left(m-1\right)^2+1}}sinx< \frac{4-m}{\sqrt{\left(m-1\right)^2+1}}\) ; \(\forall x\)
\(\Leftrightarrow\frac{4-m}{\sqrt{\left(m-1\right)^2+1}}>max\left(VT\right)=1\)
\(\Leftrightarrow4-m>\sqrt{\left(m-1\right)^2+1}\)
\(\Leftrightarrow\left\{{}\begin{matrix}m< 4\\m^2-8m+16>m^2-2m+2\end{matrix}\right.\)
\(\Leftrightarrow m< \frac{7}{3}\)
\(\Rightarrow-10\le m\le2\)
Có \(13\) giá trị nguyên của m thỏa mãn
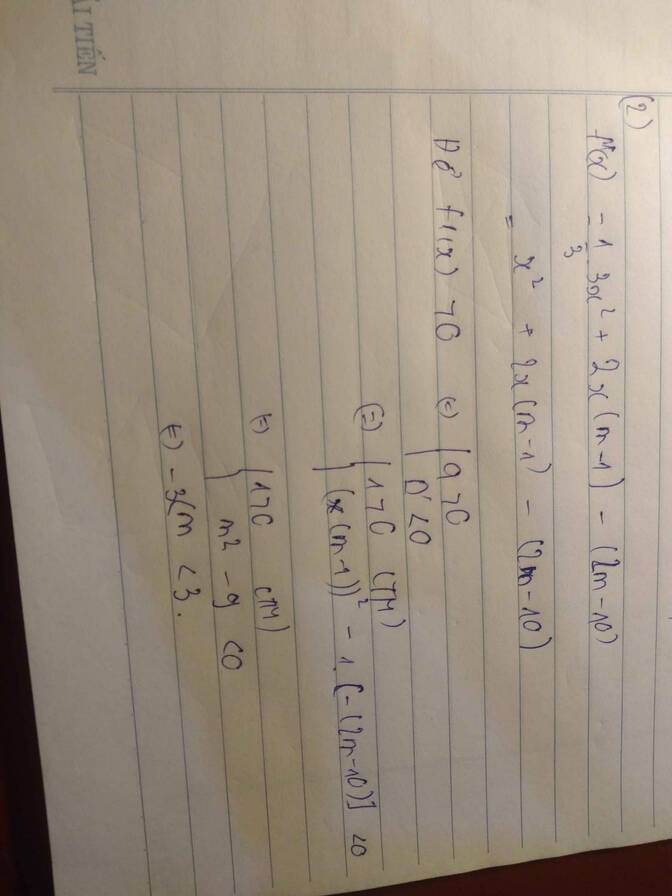
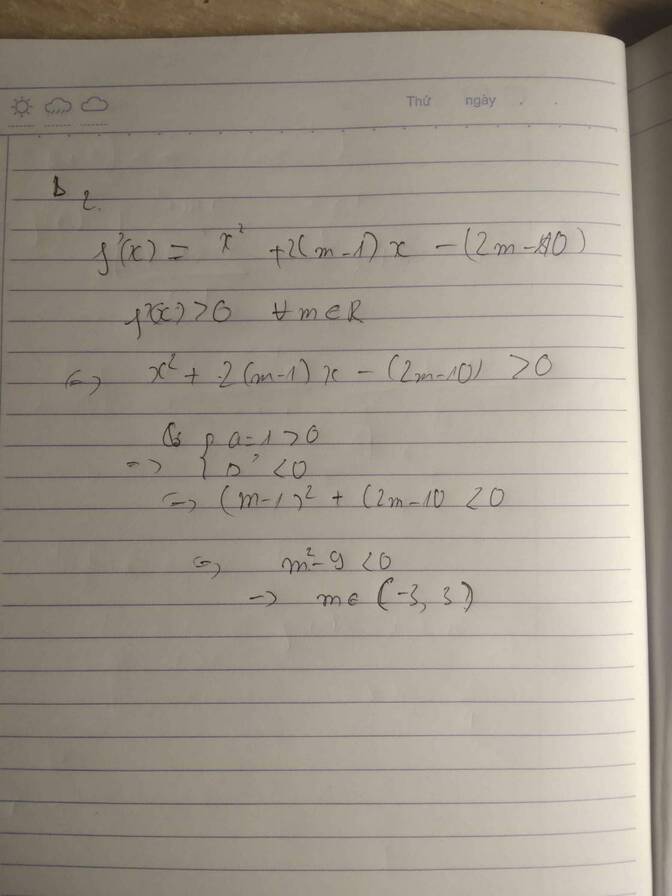
\(y'=x^2-2x+m\)
\(y'\ge0\) ; \(\forall x\in\left(1;3\right)\Leftrightarrow x^2-2x+m\ge0\) ;\(\forall x\in\left(1;3\right)\)
\(\Leftrightarrow m\ge\max\limits_{\left(1;3\right)}\left(-x^2+2x\right)\)
Xét hàm \(f\left(x\right)=-x^2+2x\) trên \(\left(1;3\right)\)
\(-\dfrac{b}{2a}=1\) ; \(f\left(1\right)=1\) ; \(f\left(3\right)=-3\)
\(\Rightarrow m\ge1\)