K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Các câu hỏi dưới đây có thể giống với câu hỏi trên
22 tháng 1 2022
a, 3 ( 1-x)(2x-1) = 5(x+8)(x-1)
<=> 3(2x-1-2x^2+x)=5(x^2+7x-8)
<=> 3(-2x^2+3x-1)=5(x^2+7x-8)
<=> -6x^2 + 9x - 3 = 5x^2 + 35x - 40
<=> -11x^2 - 26x + 37 = 0
<=> x = 1 ; x = -37/11
b, 4x^2 - 5x + 1 = 0 <=> 4x^2 - 4x - x + 1 =0
<=> 4x(x-1) - (x-1)=0 <=> (4x-1)(x-1)=0 <=> x = 1/4 ; x = 1
c, đk x khác 3 ; -3
<=> 4(x-3) + 5(x+3) = x - 5
<=> 9x + 3 = x - 5 <=> 8x = -8 <=> x = -1 (tm)
Y
5 tháng 3 2022
a)Đổi 4 giờ 30 phút = 4,5 giờ;
Gọi vận tốc ô tô là x (km/h)
=> vận tốc ca nô là x-25 (km/h) (x>25)
Đường bộ dài hơn đường thủy 10km nên ta có:
4,5(x–25)+10=2.x
⇒4,5x–4,5.25+10=2x
⇒2,5x=102,5
⇒x=41(km/h)
Vậy vận tốc ô tô là 41 km/h và ca nô là 16 km/h
b) Độ dài đường bộ là: 41.2=82 (km)
Độ dài đường thủy là: 82-10=72(km)
27 tháng 2 2022
Gọi độ dài quãng đường AB là x
Theo đề, ta có phương trình: x/30-x/40=1/2
hay x=60
2 tháng 3 2022
a: Khi a=1 thì pt sẽ là \(\dfrac{x+1}{1-x}+\dfrac{x-1}{1+x}=\dfrac{1\cdot\left(3+1\right)}{1^2-x^2}\)
\(\Leftrightarrow\left(x+1\right)^2-\left(x-1\right)^2=4\)
=>4x=4
hay x=1(loại)
b: Vì x=1 thì \(a\in\varnothing\)
nên không có giá trị nào của a để pt nhận x=1 là nghiệm
NT
3
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
8 tháng 12 2023
câu a, \(\dfrac{x}{x+1}\); \(\dfrac{x^2}{1-x}\); \(\dfrac{1}{x^2-1}\) (đk \(x\)≠ -1; 1)
\(x^2\) - 1 = ( \(x\) - 1).(\(x\) + 1)
\(\dfrac{x}{x+1}\) = \(\dfrac{x.\left(x-1\right)}{\left(x+1\right).\left(x-1\right)}\);
\(\dfrac{x^2}{1-x}\) = \(\dfrac{-x^2}{x-1}\)= \(\dfrac{-x^2.\left(x+1\right)}{\left(x-1\right)\left(x+1\right)}\)
\(\dfrac{1}{x^2-1}\) = \(\dfrac{1}{\left(x-1\right)\left(x+1\right)}\)
b, \(\dfrac{10}{x+2}\); \(\dfrac{5}{2x-4}\); \(\dfrac{1}{6-3x}\) (đk \(x\) ≠ -2; 2)
2\(x-4\) = 2.(\(x\) - 2); 6 - 3\(x\) = - 3.(\(x\) - 2)
\(\dfrac{10}{x+2}\) = \(\dfrac{10.2.3\left(x-2\right)}{2.3\left(x+2\right)\left(x-2\right)}\) = \(\dfrac{60\left(x-2\right)}{6\left(x-2\right)\left(x+2\right)}\)
\(\dfrac{5}{2x-4}\) = \(\dfrac{5.3\left(x+2\right)}{2.3\left(x-2\right).\left(x+2\right)}\) = \(\dfrac{15.\left(x+2\right)}{6.\left(x-2\right)\left(x+2\right)}\)
\(\dfrac{1}{6-3x}\) = \(\dfrac{-1}{3.\left(x-2\right)}\) = \(\dfrac{-1.\left(x+2\right)}{3.2.\left(x-2\right)\left(x+2\right)}\) = \(\dfrac{-2.\left(x+2\right)}{6.\left(x-2\right).\left(x+2\right)}\)
NT
Nguyễn Thị Thương Hoài
Giáo viên
VIP
8 tháng 12 2023
c, \(\dfrac{x}{2x-4}\); \(\dfrac{1}{2x+4}\) và \(\dfrac{3}{4-x^2}\) đk \(x\) ≠ 2; -2
\(\dfrac{x}{2x-4}\) = \(\dfrac{x}{2.\left(x-2\right)}\) = \(\dfrac{x.\left(x+2\right)}{2.\left(x-2\right).\left(x+2\right)}\)
\(\dfrac{1}{2x+4}\) = \(\dfrac{1}{2.\left(x+2\right)}\) = \(\dfrac{\left(x-2\right)}{2.\left(x+2\right).\left(x-2\right)}\)
\(\dfrac{3}{4-x^2}\) = \(\dfrac{-3}{\left(x-2\right)\left(x+2\right)}\) = \(\dfrac{-6}{2.\left(x-2\right)\left(x+2\right)}\)
17 tháng 6 2023
9:
a: XétΔABC vuông tại A và ΔHBA vuông tại H có
góc B chung
=>ΔABC đồng dạng với ΔHBA
=>BA/BH=BC/BA
=>BA^2=BH*BC
b: BC=25cm; AB=căn 9*25=15cm; AC=căn 16*25=20cm
S ABC=1/2*15*20=150cm2
C ABC=25+15+20=60cm
9 tháng 3 2022
\(a.\left|x-2\right|+3=x.\\ \Leftrightarrow\left|x-2\right|=x-3.\\ \Leftrightarrow\left\{{}\begin{matrix}x-3>0.\\\left(\left|x-2\right|\right)^2=\left(x-3\right)^2.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x>3.\\x^2-4x+4=x^2-6x+9.\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x>3.\\2x=5.\end{matrix}\right.\) \(\Leftrightarrow\left\{{}\begin{matrix}x>3.\\x=\dfrac{5}{2}.\end{matrix}\right.\) \(\Leftrightarrow x\in\phi.\)
\(b.\left(3x-4\right)\left(2x-5\right)=\left(3x-4\right)\left(x+2\right).\\ \Leftrightarrow\left(3x-4\right)\left(2x-5-x-2\right)=0.\\ \Leftrightarrow\left(3x-4\right)\left(x-7\right)=0.\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{4}{3}.\\x=7.\end{matrix}\right.\)
\(\dfrac{x}{x-2}+\dfrac{x-1}{x}=2.\left(x\ne2;0\right).\\ \Leftrightarrow\dfrac{x^2+\left(x-1\right)\left(x-2\right)-2x\left(x-2\right)}{x\left(x-2\right)}=0.\\ \Rightarrow x^2+x^2-2x-x+2-2x^2+4x=0.\\ \Leftrightarrow x=-2\left(TM\right).\)
\(d.\dfrac{x-2}{2}-\dfrac{x+5}{3}=1-\dfrac{x-2}{4}.\\ \Leftrightarrow\dfrac{6x-12-4x-20-12+3x-6}{12}=0.\\ \Rightarrow5x=50.\\ \Leftrightarrow x=10.\)
TK
1
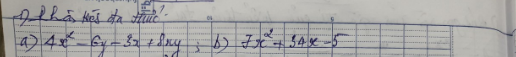
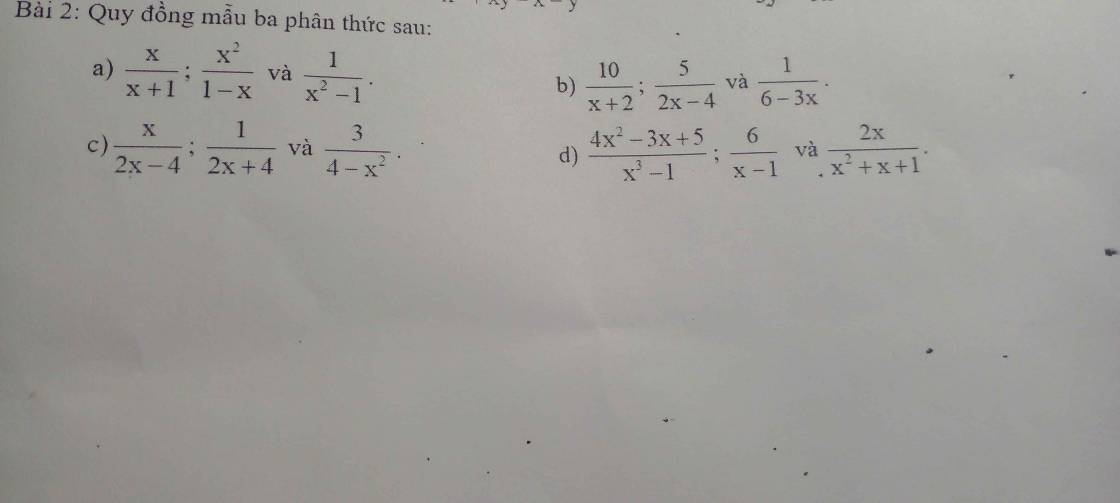
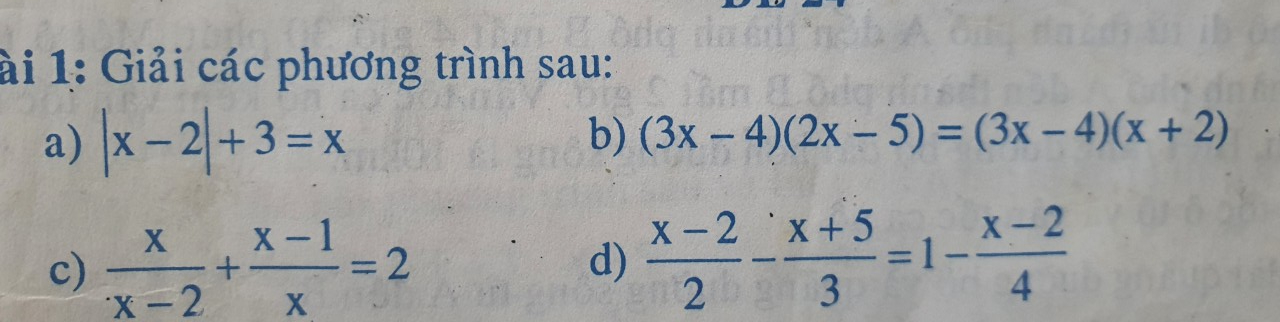
\(a,=4x\left(x+2y\right)-3\left(x+2y\right)=\left(4x-3\right)\left(x+2y\right)\\ b,=7x^2+35x-x-5=\left(x+5\right)\left(7x-1\right)\)