rút gọn 9^2-9^0-3/9^2
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(M=\dfrac{x+3+2\left(\sqrt{x}-3\right)-\left(\sqrt{x}+3\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(M=\dfrac{x+3+2\sqrt{x}-6-\sqrt{x}-3}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(M=\dfrac{x+\sqrt{x}-6}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(M=\dfrac{\left(\sqrt{x}+3\right)\left(\sqrt{x}-2\right)}{\left(\sqrt{x}+3\right)\left(\sqrt{x}-3\right)}\)
\(M=\dfrac{\sqrt{x}-2}{\sqrt{x}-3}\)

Sửa đề: \(\dfrac{\sqrt{x}}{\sqrt{x}+3}+\dfrac{2\sqrt{x}}{\sqrt{x}-3}-\dfrac{3x+9}{x-9}\)
\(=\dfrac{\sqrt{x}\left(\sqrt{x}-3\right)+2\sqrt{x}\left(\sqrt{x}+3\right)-3x-9}{x-9}\)
\(=\dfrac{x+3\sqrt{x}+2x+6\sqrt{x}-3x-9}{x-9}\)
\(=\dfrac{9\sqrt{x}-9}{x-9}\)

\(B=\dfrac{a+3\sqrt{a}-3\sqrt{a}+9-a+2}{a-9}=\dfrac{11}{a-9}\)

a) \(\dfrac{3-\sqrt{x}}{x-9}=\dfrac{-\left(\sqrt{x}-3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}=-\dfrac{1}{\sqrt{x+3}}\)(\(x\ge0,x\ne9\))
b) \(\dfrac{x-5\sqrt{x}+6}{\sqrt{x}-3}=\dfrac{\left(\sqrt{x}-3\right)\left(\sqrt{x}-2\right)}{\sqrt{x}-3}=\sqrt{x}-2\left(x\ge0,x\ne9\right)\)
a) \(\dfrac{3-\sqrt{x}}{x-9}=\dfrac{3-\sqrt{x}}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}=-\dfrac{1}{\sqrt{x}+3}\)
b) \(\dfrac{x-5\sqrt{x}+6}{\sqrt{x}-3}=\dfrac{\left(\sqrt{x}-2\right)\left(\sqrt{x}-3\right)}{\sqrt{x}-3}=\sqrt{x}-2\)
c) \(6-2x-\sqrt{9-6x+x^2}=6-2x-\sqrt{\left(3-x\right)^2}=6-2x-\left|3-x\right|\)
mà \(x< 3\Rightarrow3-x>0\Rightarrow6-2x-\left|3-x\right|=6-2x-3+x=3-x\)

Với x ≥ 0; x ≠ 9 ta có:
\(A=\dfrac{\sqrt{x}}{\sqrt{x}+3}+\dfrac{2\sqrt{x}}{\sqrt{x}-3}-\dfrac{3x+9}{x-9}\)
\(=\dfrac{\sqrt{x}\left(\sqrt{x-3}\right)+2\sqrt{x}\left(\sqrt{x}+3\right)-3x-9}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{x-3\sqrt{x}+2x+6\sqrt{x}-3x-9}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{3\sqrt{x}-9}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{3\left(\sqrt{x}-3\right)}{\left(\sqrt{x}-3\right)\left(\sqrt{x}+3\right)}\)
\(=\dfrac{3}{\sqrt{x}+3}\)
Vậy \(A=\dfrac{3}{\sqrt{x}+3}\).

\(\dfrac{2\sqrt{a}}{\sqrt{a}+3}+\dfrac{\sqrt{a}+1}{\sqrt{a}-3}+\dfrac{3+7\sqrt{a}}{9-a}\)
\(=\dfrac{2\sqrt{a}\left(\sqrt{a}-3\right)}{\left(\sqrt{a}-3\right)\left(\sqrt{a}+3\right)}+\dfrac{\left(\sqrt{a}+1\right)\left(\sqrt{a}+3\right)}{\left(\sqrt{a}-3\right)\left(\sqrt{a}+3\right)}-\dfrac{3+7\sqrt{a}}{\left(\sqrt{a}-3\right)\left(\sqrt{a}+3\right)}\)
\(=\dfrac{2a-6\sqrt{a}+a+4\sqrt{a}+3-3-7\sqrt{a}}{\left(\sqrt{a}-3\right)\left(\sqrt{a}+3\right)}\)
\(=\dfrac{3a-9\sqrt{a}}{\left(\sqrt{a}-3\right)\left(\sqrt{a}+3\right)}=\dfrac{3\sqrt{a}\left(\sqrt{a}-3\right)}{\left(\sqrt{a}-3\right)\left(\sqrt{a}+3\right)}\)
\(=\dfrac{3\sqrt{a}}{\sqrt{a}+3}\)


\(A=2^0+2^1+2^2\)\(+2^3+...+\)\(2^{50}\)
\(2A=2+2^2+2^3+...+2^{51}\)
\(2A-A=A=2^{51}-2^0\)
\(B=5+5^2+5^3+...+5^{99}+5^{100}\)
\(5B=5^2+5^3+5^4+...+5^{100}+5^{101}\)
\(5B-B=4B=5^{101}-5\)
\(B=\frac{5^{101}-5}{4}\)
\(C=3-3^2+3^3-3^4+...+\)\(3^{2007}-3^{2008}+3^{2009}-3^{2010}\)
\(3C=3^2-3^3+3^4-3^5+...-3^{2008}+3^{2009}-3^{2010}+3^{2011}\)
\(3C+C=4C=3^{2011}+3\)
\(C=\frac{3^{2011}+3}{4}\)
\(S_{100}=5+5\times9+5\times9^2+5\times9^3+...+5\times9^{99}\)
\(S_{100}=5\times\left(1+9+9^2+9^3+...+9^{99}\right)\)
\(9S_{100}=5\times\left(9+9^2+9^3+...+9^{99}+9^{100}\right)\)
\(9S_{100}-S_{100}=8S_{100}=5\times\left(9^{100}-1\right)\)
\(S_{100}=\frac{5\times\left(9^{100}-1\right)}{8}\)

1) Rút gọn biểu thức M: M = (2√x)/(√x - 3) - (x + 9√x)/(x - 9) = (2√x(x - 9) - (x + 9√x)(√x - 3))/(√x - 3)(x - 9) = (2x√x - 18√x - x√x + 9x + 9x - 27√x - 9√x + 27 )/(√x - 3)(x - 9) = (2x√x - 36√x + 27x)/(√x - 3)(x - 9) = (x(2√x - 36) + 27x) /(√x - 3)(x - 9) = (x(2√x - 36 + 27))/(√x - 3)(x - 9) = (x(2√x - 9))/( √x - 3)(x - 9) Do đó biểu thức M Rút gọn là: M = (x(2√x - 9))/(√x - 3)(x - 9) 2) Tìm các giá trị của x ă mãn M/N.(căn x + 3) = 3x - 5: Ta có phương trình: M/N.(căn x + 3) = 3x - 5 Đặt căn x + 3 = t, t >= 0, ta có x = t^2 - 3 Thay x = t^2 - 3 vào biểu thức M/N, ta có: M/N = [(t^2 - 3)(2√(t^2 - 3) - 9)]/[(t^2 - 3 + 5)t] = [(2(t^2 - 3) √(t^2 - 3) - 9(t^2 - 3))]/(t^3 + 2t - 3t - 6) = [2(t^2 - 3)√(t^2 - 3) - 9(t^2 - 3)]/(t(t - 1)(t + 2)) Đặt u = t^2 - 3, ta có: M/N = [2u√u - 9u]/((u + 3)(u + 2)) = [u(2√u - 9)]/((u + 3)(u + 2)) Đặt v = √u, ta có: M/N = [(v^ 2 + 3)(2v - 9)]/[((v^2 + 3)^2 - 3)(v^2 + 2)] = [(2v^3 - 18v + 6v - 54)]/[ ( (v^4 + 6v^2 + 9) - 3)(v^2 + 2)] = (2v^3 - 12v - 54)/(v^4 + 6v^2 + 6v^2 - 9v^2 + 18) = (2v^3 - 12v - 54)/(v^4 + 12v^2 + 18) Ta cần tìm các giá trị của v đối xứng phương trình M/N = 3x - 5: (2v^3 - 12v - 54)/(v^4 + 12v^2 + 18) = 3(t^2 - 3) - 5 (2v ^3 - 12v - 54)/(v^4 + 12v^2 + 18) = 3t^ 2 - 14 (2v^3 - 12v - 54) = (v^4 + 12v^2 + 18)(3t^2 - 14) Tuy nhiên, từ t = √(t^2 - 3), ta có v = √u = √(t^2 - 3) => (2(v^2)^3 - 12(v^2) - 54) = ((v^2)^4 + 12(v^2)^2 + 18) (3(v^2 - 3) - 14) => 2v^
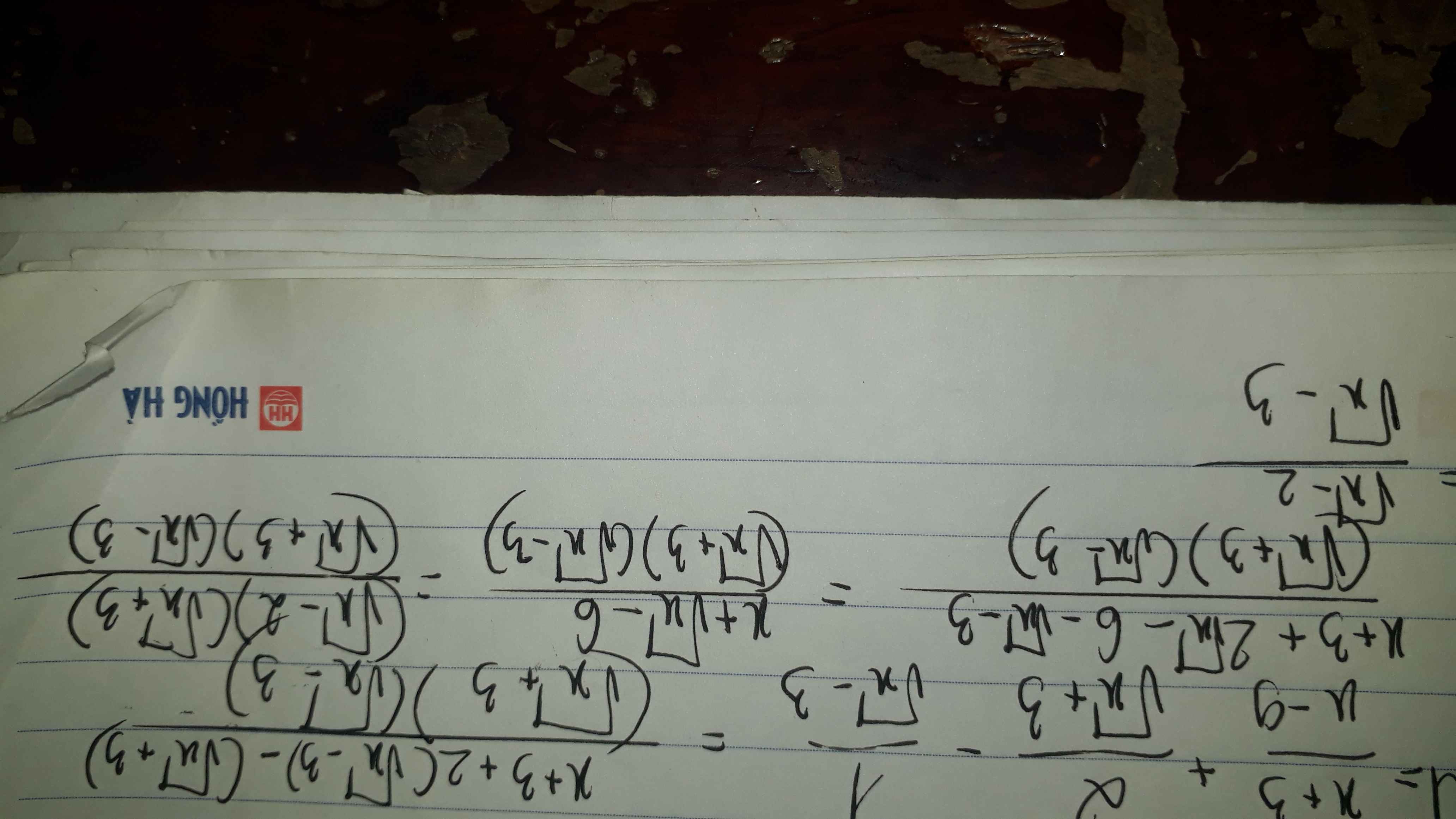
\(\frac{9^2-9^0-3}{9^2}=\frac{9^2-1-3}{9^2}=\frac{81-1-3}{81}=\frac{77}{81}\)