Tìm giá trị nhỏ nhất A= 16x2 -8x + 5
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
16 tháng 7 2023
\(C=16x^2-8x+2024\)
\(\Rightarrow C=16x^2-8x+1+2023\)
\(\Rightarrow C=\left(4x-1\right)^2+2023\ge2023\left(\left(4x-1\right)^2\ge0\right)\)
\(\Rightarrow Min\left(C\right)=2023\)
\(D=-25x^2+50x-2023\)
\(\Rightarrow D=-\left(25x^2-50x+25\right)-1998\)
\(\Rightarrow D=-\left(5x-5\right)^2-1998\le1998\left(-\left(5x-5\right)^2\le0\right)\)
\(\Rightarrow Max\left(D\right)=1998\)
\(B=-x^2+20x+100=-\left(x^2-20x+100\right)+200=-\left(x-10\right)^2+200\le200\left(-\left(x-10\right)^2\le0\right)\)
\(\Rightarrow Max\left(B\right)=200\)
\(E=\left(2x-1\right)^2-\left(3x+2\right)\left(x-5\right)\)
\(\Rightarrow E=4x^2-4x+1-\left(3x^2-13x-10\right)\)
\(\Rightarrow E=4x^2-4x+1-3x^2+13x+10\)
\(\Rightarrow E=x^2+9x+11=x^2+9x+\dfrac{81}{4}-\dfrac{81}{4}+11\)
\(\Rightarrow E=\left(x+\dfrac{9}{2}\right)^2-\dfrac{37}{4}\ge-\dfrac{37}{4}\left(\left(x+\dfrac{9}{2}\right)^2\ge0\right)\)
\(\Rightarrow Min\left(E\right)=-\dfrac{37}{4}\)
\(F=\left(3x-5\right)^2-\left(3x+2\right)\left(4x-1\right)\)
\(\Rightarrow F=9x^2-30x+25-\left(12x^2+3x-2\right)\)
\(\Rightarrow F=-3x^2-33x+27=-3\left(x^2-10x+9\right)\)
\(\Rightarrow F=-3\left(x^2-10x+25\right)+48=-3\left(x-5\right)^2+48\le48\left(-3\left(x-5\right)^2\le0\right)\)
\(\Rightarrow Max\left(F\right)=48\)
CM
24 tháng 11 2019
Ta có
B = 4 – 16 x 2 – 8 x = 5 – ( 16 x 2 + 8 x + 1 ) = 5 – [ ( 4 x ) 2 + 2 . 4 x . 1 + 1 2 ] = 5 – ( 4 x + 1 ) 2
Nhận thấy 4 x + 1 2 ≥ 0; Ɐx
=> 5 – 4 x + 1 2 ≤ 5
Dấu “=” xảy ra khi 4 x + 1 2 = 0 ó x = - 1 4
Đáp án cần chọn là: A
C
1
13 tháng 6 2021
`A=16x^2+8x+5`
`=16x^2+8x+1+4`
`=(4x+1)^2+4>=4`
Dấu "=" xảy ra khi `4x+1=0<=>x=-1/4`
`B=x^2-x`
`=x^2-x+1/4-1/4`
`=(x-1/2)^2-1/4>=-1/4`
Dấu "=" xảy ra khi `x=1/2`
`C=a^2-2a+b^2+6b+2021`
`=a^2-2a+1+b^2+6b+9+2011`
`=(a-1)^2+(b+3)^2+2011>=2011`
Dấu "=" xảy ra khi \(\begin{cases}a=1\\b=-3\\\end{cases}\)
KN
23 tháng 8 2020
\(A=5-8x+x^2=-8x+x^2+6-11\)
\(=\left(x-4\right)^2-11\)
Vì \(\left(x-4\right)^2\ge0\forall x\)\(\Rightarrow\left(x-4\right)^2-11\ge-11\)
Dấu "=" xảy ra \(\Leftrightarrow\left(x-4\right)^2=0\Leftrightarrow x-4=0\Leftrightarrow x=4\)
Vậy Amin = - 11 <=> x = 4
KN
23 tháng 8 2020
\(B=\left(2-x\right)\left(x+4\right)=-x^2-2x+8\)
\(=-\left(x^2+2x+1\right)+9=-\left(x+1\right)^2+9\)
Vì \(\left(x+1\right)^2\ge0\forall x\)\(\Rightarrow-\left(x+1\right)^2+9\le9\)
Dấu "=" xảy ra \(\Leftrightarrow-\left(x+1\right)^2=0\Leftrightarrow x+1=0\Leftrightarrow x=-1\)
Vậy Bmax = 9 <=> x = - 1
HV
3
MN
1
AH
Akai Haruma
Giáo viên
30 tháng 10 2023
Lời giải:
$A=(x^2+4y^2+4xy)+x^2+5-8x-12y$
$=(x+2y)^2-6(x+2y)+x^2+5-2x$
$=(x+2y)^2-6(x+2y)+9+(x^2-2x+1)-5$
$=(x+2y-3)^2+(x-1)^2-5\geq 0+0-5=-5$
Vậy $A_{\min}=-5$. Giá trị này đạt được khi $x+2y-3=x-1=0$
$\Leftrightarrow x=1; y=1$
TT
1
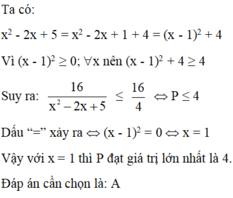
A = 16x2 - 8x + 5
A = [ ( 4x )2 - 2 . 4x . 1 + 1 ] + 4
A = ( 4x - 1 )2 + 4
Vì ( 4x - 1 )2 \(\ge\) 0 \(\forall\)x
=> ( 4x - 1 )2 + 4 \(\ge\)4 \(\forall\)x
=> A \(\ge\)4 \(\forall\)x
=> A = 4 <=> ( 4x - 1 )2 = 0
<=> 4x - 1 = 0
<=> 4x = 1
<=> x = \(\frac{1}{4}\)
Vậy A min = 4 <=> x = \(\frac{1}{4}\)