Tìm x biết :
16x : 4x = 16
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
AH
Akai Haruma
Giáo viên
30 tháng 11 2023
Lời giải:
$x^2=4.4.4.4=16.16=(-16)(-16)=16^2=(-16)^2$
$\Rightarrow x=16$ hoặc $x=-16$.
NN
0
23 tháng 6 2023
a: =>2x^2-2x+2x-2-2x^2-x-4x-2=0
=>-5x-4=0
=>x=-4/5
b: =>6x^2-9x+2x-3-6x^2-12x=16
=>-19x=19
=>x=-1
c: =>48x^2-12x-20x+5+3x-48x^2-7+112x=81
=>83x=83
=>x=1
HM
1
11 tháng 8 2018
\(x^4+4x^3-16x-16=0\)
\(\Leftrightarrow\left(x^4-16\right)+\left(4x^3-16x\right)=0\)
\(\Leftrightarrow\left(x^2-4\right)\left(x^2+4\right)+4x\left(x^2-4\right)=0\)
\(\Leftrightarrow\left(x^2-4\right)\left(x^2+4+4x\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x-2\right)\left(x+2\right)^2=0\)
\(\Leftrightarrow\left(x-2\right)\left(x+2\right)^3=0\)
\(\Leftrightarrow\hept{\begin{cases}x-2=0\\\left(x+2\right)^3=0\end{cases}\Leftrightarrow\hept{\begin{cases}x=2\\x=-2\end{cases}}}\)
=.= hok tốt!!
V
0
CM
11 tháng 6 2019
a) Rút gọn:
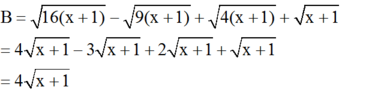
b) Để B = 16 thì:
⇔ x + 1 = 16 ⇔ x = 15 (thỏa mãn x ≥ -1)
TN
18 tháng 11 2018
Điều kiện: \(x\ne2\)
Phân tích tử thức: \(x^4-16=\left(x^2\right)^2-4^2=\left(x^2-4\right)\left(x^2+4\right)=\left(x-2\right)\left(x+2\right)\left(x^2+4\right)\)
Phân tích mẫu thức: \(x^4-4x^3+8x^2-16x+16=\left(x^4-4x^3+4x^2\right)+\left(4x^2-16x+16\right)\)
\(=x^2\left(x^2-4x+4\right)+4\left(x^2-4x+4\right)=\left(x-2\right)^2\left(x^2+4\right)\)
Ta có: \(P=\frac{\left(x-2\right)\left(x+2\right)\left(x^2+4\right)}{\left(x-2\right)^2\left(x^2+4\right)}=\frac{x+2}{x-2}=\frac{\left(x-2\right)+4}{x-2}=1+\frac{4}{x-2}\)
Để P là số nguyên thì \(x-2\inƯ\left(4\right)\)
\(\Rightarrow x-2\in\left\{-4;-2;-1;1;2;4\right\}\)
\(\Rightarrow x\in\left\{-2;0;1;3;4;6\right\}\)
19 tháng 12 2018
Điều kiện: x\ne2x̸=2
Phân tích tử thức: x^4-16=\left(x^2\right)^2-4^2=\left(x^2-4\right)\left(x^2+4\right)=\left(x-2\right)\left(x+2\right)\left(x^2+4\right)x4−16=(x2)2−42=(x2−4)(x2+4)=(x−2)(x+2)(x2+4)
Phân tích mẫu thức: x^4-4x^3+8x^2-16x+16=\left(x^4-4x^3+4x^2\right)+\left(4x^2-16x+16\right)x4−4x3+8x2−16x+16=(x4−4x3+4x2)+(4x2−16x+16)
=x^2\left(x^2-4x+4\right)+4\left(x^2-4x+4\right)=\left(x-2\right)^2\left(x^2+4\right)=x2(x2−4x+4)+4(x2−4x+4)=(x−2)2(x2+4)
Ta có: P=\frac{\left(x-2\right)\left(x+2\right)\left(x^2+4\right)}{\left(x-2\right)^2\left(x^2+4\right)}=\frac{x+2}{x-2}=\frac{\left(x-2\right)+4}{x-2}=1+\frac{4}{x-2}P=(x−2)2(x2+4)(x−2)(x+2)(x2+4)=x−2x+2=x−2(x−2)+4=1+x−24
Để P là số nguyên thì x-2\inƯ\left(4\right)x−2∈Ư(4)
\Rightarrow x-2\in\left\{-4;-2;-1;1;2;4\right\}⇒x−2∈{−4;−2;−1;1;2;4}
\Rightarrow x\in\left\{-2;0;1;3;4;6\right\}⇒x∈{−2;0;1;3;4;6}
NN
1
AH
Akai Haruma
Giáo viên
17 tháng 9 2021
Lời giải:
a. ĐKXĐ: $x\in\mathbb{R}$
PT $\Leftrightarrow \sqrt{(x-2)^2}=5$
$\Leftrightarrow |x-2|=5$
$\Leftrightarrow x-2=5$ hoặc $x-2=-5$
$\Leftrightarrow x=7$ hoặc $x=-3$ (đều tm)
b. ĐKXĐ: $x\geq -1$
PT $\Leftrightarrow \sqrt{16}.\sqrt{x+1}-3\sqrt{x+1}+\sqrt{4}.\sqrt{x+1}=16-\sqrt{x+1}$
$\Leftrightarrow 4\sqrt{x+1}-3\sqrt{x+1}+2\sqrt{x+1}=16-\sqrt{x+1}$
$\Leftrightarrow 4\sqrt{x+1}=16$
$\Leftrightarrow \sqrt{x+1}=4$
$\Leftrightarrow x+1=16$
$\Leftrightarrow x=15$ (tm)
x=1;2
sai roi