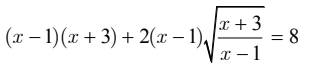( bài này giải theo phương pháp đặt ẩn phụ đúng không mọi người ? giải giúp mình với
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
S
1
Những câu hỏi liên quan
15 tháng 6 2023
Ôi, vì lười nên kết quả học tập của Lan mới kém như vậy.
Tôi thấy thương cho cô bé ấy - chỉ vì cái nghèo mà đánh mất cơ hội học tập
19 tháng 8 2021
Bài 1:
ĐKXĐ: \(x\ge\dfrac{1}{2}\)
Ta có: \(\sqrt{5x^2}=2x-1\)
\(\Leftrightarrow5x^2=\left(2x-1\right)^2\)
\(\Leftrightarrow5x^2-4x^2+4x-1=0\)
\(\Leftrightarrow x^2+4x-1=0\)
\(\text{Δ}=4^2-4\cdot1\cdot\left(-1\right)=20\)
Vì Δ>0 nên phương trình có hai nghiệm phân biệt là:
\(\left\{{}\begin{matrix}x_1=\dfrac{-4-2\sqrt{5}}{2}=-2-\sqrt{5}\left(loại\right)\\x_2=\dfrac{-4+2\sqrt{5}}{2}=-2+\sqrt{5}\left(loại\right)\end{matrix}\right.\)
AH
Akai Haruma
Giáo viên
19 tháng 8 2021
Bài 1: Bình phương hai vế lên có giải ra được kết quả. Nhưng phải kèm thêm điều kiện $2x-1\geq 0$ do $\sqrt{5x^2}\geq 0$
PT \(\Leftrightarrow \left\{\begin{matrix} 2x-1\geq 0\\ 5x^2=(2x-1)^2\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{2}\\ x^2+4x-1=0\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{2}\\ (x+2)^2-5=0\end{matrix}\right.\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{2}\\ (x+2-\sqrt{5})(x+2+\sqrt{5})=0\end{matrix}\right.\)
\(\Leftrightarrow \left\{\begin{matrix} x\geq \frac{1}{2}\\ x=-2\pm \sqrt{5}\end{matrix}\right.\) (vô lý)
Vậy pt vô nghiệm.
28 tháng 3 2020
a) \(4\sqrt{x}+\frac{2}{\sqrt{x}}< 2x+\frac{1}{2x}+2\)
hay \(2\sqrt{x}+\frac{1}{\sqrt{x}}< x+\frac{1}{4x}+1\)
\(\Leftrightarrow0< x+\frac{1}{4x}+1-2\sqrt{x}-\frac{1}{\sqrt{x}}\)
\(\Leftrightarrow0< \left(\sqrt{x}\right)^2-2\sqrt{x}-2\sqrt{x}\cdot1+1+\frac{1}{\left(2\sqrt{x}\right)^2}-2\cdot\frac{1}{2\sqrt{x}}\)
\(\Leftrightarrow1< \left(\sqrt{x}-1\right)^2+\left(\frac{1}{2\sqrt{x}}-1\right)^2\)
\(\Rightarrow\hept{\begin{cases}x>0\\\sqrt{x}>1\\2\sqrt{x}>1\end{cases}\Rightarrow\hept{\begin{cases}x>1\\x>\frac{1}{4}\end{cases}\Rightarrow}x>1}\)
b) \(\frac{1}{1-x^2}>\frac{3}{\sqrt{1-x^2}}-1\left(1\right)\left(ĐK:-1< x< 1\right)\)
Ta có (1) <=> \(\frac{1}{1-x^2}-1-\frac{3x}{\sqrt{1-x^2}}+2>0\)\(\Leftrightarrow\frac{x^2}{1-x^2}-\frac{3x}{\sqrt{1-x^2}}+2>0\)
Đặt \(t=\frac{x}{\sqrt{1-x^2}}\)ta được
\(t^2-3t+2>0\Leftrightarrow\orbr{\begin{cases}\frac{x}{\sqrt{1-x^2}}< 1\\\frac{x}{\sqrt{1-x^2}}>2\end{cases}\Leftrightarrow\orbr{\begin{cases}\sqrt{1-x^2}>x\left(a\right)\\2\sqrt{1-x^2}< x\left(b\right)\end{cases}}}\)
(a) <=> \(\hept{\begin{cases}x< 0\\1-x^2>0\end{cases}\Leftrightarrow\hept{\begin{cases}x\ge0\\1-x^2>x^2\end{cases}}}\)
\(\Leftrightarrow-1< x< 0\)hoặc \(\hept{\begin{cases}x\ge0\\x^2< \frac{1}{2}\end{cases}}\)
\(\Leftrightarrow-1< x< 0\)hoặc \(0\le x\le\frac{\sqrt{2}}{2}\Leftrightarrow-1< x< \frac{\sqrt{2}}{2}\)
(b) \(\Leftrightarrow\hept{\begin{cases}1-x^2>0\\x>0\\4\left(1-x^2\right)< x^2\end{cases}\Leftrightarrow\hept{\begin{cases}0< x< 1\\x^2>\frac{4}{5}\end{cases}\Leftrightarrow}\frac{2}{\sqrt{5}}< x< 1}\)
BD
31 tháng 10 2016
Bạn ơi , mình cho bạn ví dụ và hướng dẫn cách làm nha
f(x)=3x3 – 7x2 + 17x–5f(x)
Hướng dẫn:
±1,±5±1,±5 không là nghiệm của f(x)f(x), như vậy f(x)f(x) không có nghiệm nguyên. Nên f(x)f(x) nếu có nghiệm thì là nghiệm hữu tỉ
Ta nhận thấy x=x= 1313 là nghiệm của f(x)f(x) do đó f(x)f(x) có một nhân tử là 3x–13x–1. Nên
f(x)= 3x3 – 7x2 + 17x – 5 = 3x3− x2− 6x2 + 2x + 15x − 5f(x)
= 3x3 – 7x2 + 17x – 5 = 3x3 − x2 − 6x2 + 2x + 15x − 5
= (3x3−x2 ) − ( 6x2 −2x ) + (15x−5) = (3x3 − x2) − (6x2 − 2x) + (15x−5)
= x2 ( 3x−1 )− 2x(3x−1) + 5(3x−1) = (3x − 1)(x2 − 2x + 5 )
Vì x2 − 2x + 5 = (x2 − 2x + 1) + 4 = (x−1)2 + 4>0x2 − 2x + 5= (x2 − 2x + 1) + 4= (x−1)2 + 4>0 với mọi xx nên không phân tích được thành nhân tử nữa
31 tháng 10 2016
ình muốn giúp lắm nhưng mình......chưa học.mình mới học lớp 7
H9
HT.Phong (9A5)
CTVHS
25 tháng 1
\(\left\{{}\begin{matrix}\dfrac{1}{x}=\dfrac{3}{2}+\dfrac{1}{y}\\\dfrac{1}{x}+\dfrac{1}{y}=\dfrac{1}{24}\end{matrix}\right.\) (Đk: x,y ≠ 0)
Đặt: \(\dfrac{1}{x}=u;\dfrac{1}{y}=v\)
Hệ trở thành:
\(\left\{{}\begin{matrix}u=\dfrac{3}{2}+v\\u+v=\dfrac{1}{24}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u=\dfrac{3}{2}+v\\\dfrac{3}{2}+v+v=\dfrac{1}{24}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u=\dfrac{3}{2}+v\\2v=-\dfrac{35}{24}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}u=\dfrac{37}{48}\\v=-\dfrac{35}{48}\end{matrix}\right.\)
\(\Rightarrow\left\{{}\begin{matrix}\dfrac{1}{x}=\dfrac{37}{48}\\\dfrac{1}{y}=\dfrac{-35}{48}\end{matrix}\right.\)
\(\Leftrightarrow\left\{{}\begin{matrix}x=\dfrac{48}{37}\\y=-\dfrac{48}{35}\end{matrix}\right.\)
Vậy: \(\left(x;y\right)=\left(\dfrac{48}{37};-\dfrac{48}{35}\right)\)
7 tháng 2 2019
Bài 1 :
Mình nghĩ phải sửa đề ntn :
\(4\left(2x+7\right)^2-9\left(x+3\right)^2=0\)
\(\Leftrightarrow\left[2\left(2x+7\right)\right]^2-\left[3\left(x+3\right)\right]^2=0\)
\(\Leftrightarrow\left[2\left(2x+7\right)-3\left(x+3\right)\right]\left[2\left(2x+7\right)+3\left(x+3\right)\right]=0\)
\(\Leftrightarrow\left(4x+14-3x-9\right)\left(4x+14+3x+9\right)=0\)
\(\Leftrightarrow\left(x+5\right)\left(7x+23\right)=0\)
\(\Leftrightarrow\orbr{\begin{cases}x+5=0\\7x+23=0\end{cases}\Leftrightarrow\orbr{\begin{cases}x=-5\\x=\frac{-23}{7}\end{cases}}}\)
Vậy....
b) \(A=\left(x^2+x+1\right)\left(x^2+x+2\right)-12\)
Đặt \(q=x^2+x+1\)ta có :
\(A=q\left(q+1\right)-12\)
\(A=q^2+q-12\)
\(A=q^2+4q-3q-12\)
\(A=q\left(q+4\right)-3\left(q+4\right)\)
\(A=\left(q+4\right)\left(q-3\right)\)
Thay \(q=x^2+x+1\)ta có :
\(A=\left(x^2+x+1+4\right)\left(x^2+x+1-3\right)\)
\(A=\left(x^2+x+5\right)\left(x^2+x-2\right)\)
\(A=\left(x^2+x+5\right)\left(x^2+2x-x-2\right)\)
\(A=\left(x^2+x+5\right)\left[x\left(x+2\right)-\left(x+2\right)\right]\)
\(A=\left(x^2+x+5\right)\left(x+2\right)\left(x-1\right)\)
21 tháng 9 2021
Đặt \(\dfrac{1}{y-1}=a\), hpt tở thành
\(\left\{{}\begin{matrix}\dfrac{5}{x+1}+a=10\\\dfrac{1}{x-2}+3a=18\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}\dfrac{15}{x+1}+3a=30\left(1\right)\\\dfrac{1}{x-1}+3a=18\left(2\right)\end{matrix}\right.\)
Lấy \(\left(1\right)-\left(2\right)\), ta được:
\(\dfrac{15}{x+1}-\dfrac{1}{x-1}=12\\ \Leftrightarrow\dfrac{15x-15-x-1}{\left(x-1\right)\left(x+1\right)}=12\\ \Leftrightarrow12x^2-12=14x-16\\ \Leftrightarrow12x^2-14x+4=0\\ \Leftrightarrow\left(3x-2\right)\left(2x-1\right)=0\\ \Leftrightarrow\left[{}\begin{matrix}x=\dfrac{1}{2}\\x=\dfrac{2}{3}\end{matrix}\right.\)
Với \(x=\dfrac{1}{2}\Leftrightarrow\dfrac{10}{3}+\dfrac{1}{y-1}=10\Leftrightarrow\dfrac{10y-7}{3\left(y-1\right)}=10\)
\(\Leftrightarrow30y-30=10y-7\Leftrightarrow y=\dfrac{23}{20}\)
Với \(x=\dfrac{2}{3}\Leftrightarrow3+\dfrac{1}{y-1}=10\Leftrightarrow\dfrac{1}{y-1}=7\Leftrightarrow7y-7=1\Leftrightarrow y=\dfrac{8}{7}\)
Vậy \(\left(x;y\right)=\left\{\left(\dfrac{1}{2};\dfrac{23}{20}\right);\left(\dfrac{2}{3};\dfrac{8}{7}\right)\right\}\)
BH
23 tháng 8 2019
\(\frac{1}{x-3}=a,\frac{1}{y-4}=b\)
\(hpt\Leftrightarrow\hept{\begin{cases}a+b=\frac{5}{3}\\4a-3b=\frac{3}{2}\end{cases}\Rightarrow\hept{\begin{cases}a=\frac{13}{14}\\b=\frac{31}{42}\end{cases}\Rightarrow}}\hept{\begin{cases}x=\frac{53}{13}\\y=\frac{166}{31}\end{cases}}\)
đkxđ: \(\dfrac{x+3}{x-1}\ge0\)
Ptr ⇔\(\left(x-1\right)\left(x+3\right)+\dfrac{2\left(x-1\right)\sqrt{\left(x+3\right)\left(x-1\right)}}{x-1}=8\\ \Leftrightarrow\left(x-1\right)\left(x+3\right)+2\sqrt{\left(x-1\right)\left(x+3\right)}-8=0\)
Đặt \(\sqrt{\left(x-1\right)\left(x+3\right)}=a\) (a≥0)
Ptr ⇔ \(a^2+2a-8=0\)
⇔a=2 (tm) hoặc a=-4 (loại)
⇒\(\sqrt{\left(x-1\right)\left(x+3\right)}=2\)
⇔\(x^2+2x-3=4\)
\(\Leftrightarrow x^2+2x-7=0\)
⇔ \(x=-1+2\sqrt{2}\) (tm)
hoặc \(x=-1-2\sqrt{2}\) (tm)
Vậy...