Cho Q = 2x2 - 6x + x2 + 6x - 12. Tìm x để Q nhận giá trị là 0.
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
CM
23 tháng 8 2017
Đáp án D
Ta có lim x → 2 − f x = lim x → 2 − 2 x 2 − 7 x + 6 x − 2 = lim x → 2 − 2 x 2 − 7 x + 6 x − 2 = lim x → 2 − − 2 x − 3 = − 1
Và lim x → 2 − f x = lim x → 2 − a + 1 − x 2 + x = a − 1 4 ; f 2 = a − 1 4 .
Theo bài ra, ta có lim x → 2 + f x = lim x → 2 − f x = f 2 ⇒ a = − 3 4
Do đó, bất phương trình − x 2 + a x + 7 4 > 0 ⇔ − x 2 − 3 4 x + 7 4 > 0 ⇔ − 7 4 < x < 1.
TC
2
9 tháng 8 2016
a) \(P=x^2-2x+5\)
\(=x^2-2x+1+4\)
\(=\left(x-1\right)^2+4\ge4\)
\(MinP=4\Leftrightarrow x-1=0\Rightarrow x=1\)
b) \(Q=2x^2-6x\)
\(=2\left(x^2-3x\right)\)
\(=2\left(x^2-2.x.\frac{3}{2}+\frac{9}{4}-\frac{9}{4}\right)\)
\(=2\left(\left(x-\frac{3}{2}\right)^2-\frac{9}{4}\right)\)
\(=-\frac{9}{2}-2\left(x-\frac{3}{2}\right)^2\le\frac{-9}{2}\)
\(MinQ=\frac{-9}{2}\Leftrightarrow x-\frac{3}{2}=0\Rightarrow x=\frac{3}{2}\)
9 tháng 8 2016
M=x^2+y^2-x+6y+10
M=(x^2-x+1/4)+(y^2+6y+9)+3/4
M=(x-1/2)^2+(y+3)^2+3/4
\(minM=\frac{3}{4}\Leftrightarrow\hept{\begin{cases}x=\frac{1}{2}\\y=-3\end{cases}}\)
NV
Nguyễn Việt Lâm
Giáo viên
11 tháng 7 2021
\(2x^2+6x-5=2\left(x+\dfrac{3}{2}\right)^2-\dfrac{19}{2}\ge-\dfrac{19}{2}\)
Dấu "=" xảy ra khi \(x=-\dfrac{3}{2}\)
\(x^2-x+1=\left(x^2-x+\dfrac{1}{4}\right)+\dfrac{3}{4}=\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}\ge\dfrac{3}{4}\)
Dấu "=" xảy ra khi \(x=\dfrac{1}{2}\)
27 tháng 7 2021
Bài 1:
a) Ta có: \(P=1+\dfrac{3}{x^2+5x+6}:\left(\dfrac{8x^2}{4x^3-8x^2}-\dfrac{3x}{3x^2-12}-\dfrac{1}{x+2}\right)\)
\(=1+\dfrac{3}{\left(x+2\right)\left(x+3\right)}:\left(\dfrac{8x^2}{4x^2\left(x-2\right)}-\dfrac{3x}{3\left(x-2\right)\left(x+2\right)}-\dfrac{1}{x+2}\right)\)
\(=1+\dfrac{3}{\left(x+2\right)\left(x+3\right)}:\left(\dfrac{4}{x-2}-\dfrac{x}{\left(x-2\right)\left(x+2\right)}-\dfrac{1}{x+2}\right)\)
\(=1+\dfrac{3}{\left(x+2\right)\left(x+3\right)}:\dfrac{4\left(x+2\right)-x-\left(x-2\right)}{\left(x-2\right)\left(x+2\right)}\)
\(=1+\dfrac{3}{\left(x+2\right)\left(x+3\right)}\cdot\dfrac{\left(x-2\right)\left(x+2\right)}{4x+8-x-x+2}\)
\(=1+3\cdot\dfrac{\left(x-2\right)}{\left(x+3\right)\left(2x+10\right)}\)
\(=1+\dfrac{3\left(x-2\right)}{\left(x+3\right)\left(2x+10\right)}\)
\(=\dfrac{\left(x+3\right)\left(2x+10\right)+3\left(x-2\right)}{\left(x+3\right)\left(2x+10\right)}\)
\(=\dfrac{2x^2+10x+6x+30+3x-6}{\left(x+3\right)\left(2x+10\right)}\)
\(=\dfrac{2x^2+19x-6}{\left(x+3\right)\left(2x+10\right)}\)
ND
Nguyễn Đức Trí
VIP
8 tháng 7 2023
a) P(x)=4x2-6x+a; Q(x)=x-3
Lấy P(x):Q(x)=4x-6 dư a+30
Vậy để P(x)⋮Q(x) ⇒ a+30=0 ⇒ a=-30
b) P(x)=2x2+x+a; Q(x)=x+3
Lấy P(x):Q(x)=2x-7 dư a+21
Vậy để P(x)⋮Q(x) ⇒ a+21=0 ⇒ a=-21
c) P(x)=x3+ax2-4; Q(x)=x2+4x+4
Lấy P(x):Q(x)=x+a-4 dư -4(a-5)x+12
Vậy để P(x)⋮Q(x) ⇒ -4(a-5)x+12=0 ⇒ (a-5)x=3
⇒ a-5 ϵ {-1;1;-3;3} (a ϵ Z)
⇒ a ϵ {4;6;2;8}
d) P(x)=2x2+ax+1; Q(x)=x-3
Lấy P(x):Q(x)=2x+a+6 dư 3a+19
Vậy để P(x)⋮Q(x) ⇒ 3a+19=0 ⇒ a=-19/3
e) P(x)=ax5+5x4-9; Q(x)=x-1
Lấy P(x):Q(x)=ax4+(a-5)x3+(a-5)x2+(a-5)x+1 dư a-4
Vậy để P(x)⋮Q(x) ⇒ a-4=0 ⇒ a=4
f) P(x)=6x3-x2-23x+a; Q(x)=2x+3
Lấy P(x):Q(x)=3x2-5x-4 dư a+12
Vậy để P(x)⋮Q(x) ⇒ a+12=0 ⇒ a=-12
g) P(x)=x3-6x2+ax-6 Q(x)=x-2
Lấy P(x):Q(x)=x2-2x+a-4 dư 2(a-4)-6
Vậy để P(x)⋮Q(x) ⇒ 2(a-4)-6=0 ⇒ a=7
Bài h có a,b bạn xem lại đề
CH
4
TL
28 tháng 6 2016
\(B=\frac{3\left(x+2\right)-4}{x+2}\)\(=3-\frac{4}{x+2}\)
Để B nhận giá trị nguyên thì \(x-2\inƯ_{\left(4\right)}=\left\{\pm1;\pm2;\pm4\right\}\)
Với \(x+2=-4\Rightarrow x=-6\)
\(x+2=-2\Rightarrow x=-4\)
\(x+2=-1\Rightarrow x=-3\)
\(x+2=1\Rightarrow x=-1\)
\(x+2=2\Rightarrow x=0\)
\(x+2=4\Rightarrow x=2\)
28 tháng 6 2016
ta có : \(\frac{6x+2}{x+2}=\frac{6}{x+2}+1\)
Để B nguyên thì \(6⋮x+2\) \(\Rightarrow\left(x+2\right)\inƯ\left(6\right)=\left\{-1;-2;-3;-6;1;2;3;6\right\}\)
ta có :
| x+2 | x |
| -1 | -3 |
| -2 | -4 |
| -3 | -5 |
| -6 | -8 |
| 1 | -1 |
| 2 | 1 |
| 3 | 2 |
| 6 | 5 |
NH
4
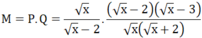
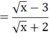
+) \(Q=2x^2-6x+x^2+6x-12\)
\(=\left(2x^2+x^2\right)+\left(-6x+6x\right)-12\)
\(=3x^2-12\)
\(Cho\) \(Q=0\) \(\Rightarrow3x^2-12=0\)
\(\Rightarrow3x^2=12\)
\(\Rightarrow x^2=4\)
\(\Rightarrow x=2\)\(hay\)\(x=-2\)
VẬY ........... ( NẾU SAI THÌ THÔI NHÉ >-< )
\(Q=2x^2-6x+x^2+6x-12\)
\(Q=\left(2x^2+x^2\right)+\left(-6x+6x\right)-12\)
\(Q=3x^2-12\)
\(\Leftrightarrow3x^2=0+12\)
\(\Leftrightarrow x^2=12:3\)
\(\Leftrightarrow x^2=4=2^2\Rightarrow x=2\)
Vậy với \(x=2\)thì \(Q=0\)
Các bạn nữ (xinh) k và kb làm người yêu mình nha !!!!!!!!!!!!!!!