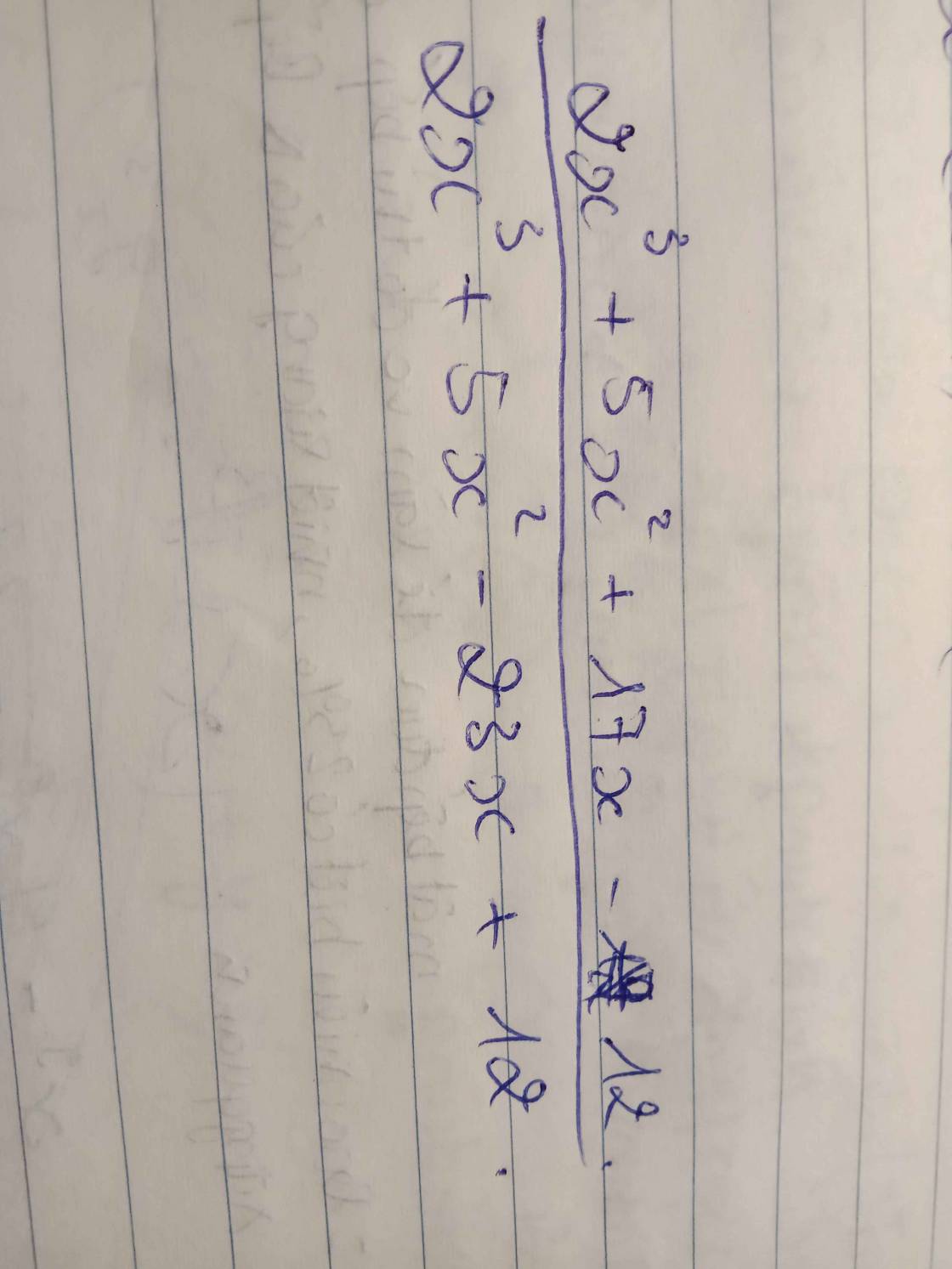Rút gọn biểu thức
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
18 tháng 1 2019
Bài làm
| a | . a
= a . a
= a2
# Chúc bạn học tốt #
AH
Akai Haruma
Giáo viên
9 tháng 12 2023
Lời giải:
$(2x+3)^2+(2x+5)^2=4x^2+12x+9+4x^2+20x+25$
$=8x^2+32x+34$
ML
0
4 tháng 7 2015
đk: x>=0; x khác 3
a) \(P=\frac{\sqrt{x}-3}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-3\right)}-\frac{5}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-3\right)}+\frac{\left(\sqrt{x}-2\right)\left(\sqrt{x}+2\right)}{\sqrt{x}-3}=\frac{\sqrt{x}-3-5+x-4}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-3\right)}=\frac{x+\sqrt{x}-12}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-3\right)}\)
\(P=\frac{\left(\sqrt{x}+4\right)\left(\sqrt{x}-3\right)}{\left(\sqrt{x}+2\right)\left(\sqrt{x}-3\right)}=\frac{\sqrt{x}+4}{\sqrt{x}+2}\)
b) \(P=\frac{\sqrt{x}+2+2}{\sqrt{x}+2}=1+\frac{2}{\sqrt{x}+2}\)
ta có: \(x\ge0\Rightarrow\sqrt{x}\ge0\Leftrightarrow\sqrt{x}+2\ge2\Leftrightarrow\frac{2}{\sqrt{x}+2}\le1\Leftrightarrow1+\frac{2}{\sqrt{x}+2}\le2\Rightarrow MaxP=2\Rightarrow x=0\)