MN ơi giúp em với ạ em cảm ơn
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NQ
0
9 tháng 9 2021
\(\left(3x-4\right)^3=5^2+4.5^2\)
\(\Leftrightarrow\left(3x-4\right)^3=5^2\left(1+4\right)\)
\(\Leftrightarrow\left(3x-4\right)^3=5^3\)
\(\Leftrightarrow3x-4=5\Leftrightarrow3x=9\Leftrightarrow x=3\)
9 tháng 9 2021
Ta có: \(\left(3x-4\right)^3=5^2+4\cdot5^2\)
\(\Leftrightarrow3x-4=5\)
hay x=3
AH
Akai Haruma
Giáo viên
4 tháng 9 2021
Lời giải:
$A=7+(7^2+7^3+7^4+7^5)+(7^6+7^6+7^8+7^9)+....+(7^{2018}+7^{2019}+7^{2020}+7^{2021})$
$=7+7^2(1+7+7^2+7^3)+7^6(1+7+7^2+7^3)+....+7^{2018}(1+7+7^2+7^3)$
$=7+(1+7+7^2+7^3)(7^2+7^6+....+7^{2018}$
$=7+400(7^2+7^6+....+7^{2018})$
Dễ thấy $400(7^2+7^6+....+7^{2018})$ tận cùng là $0$
Do đó $A$ tận cùng là $7$
12 tháng 9 2021
\(3\left(x+2\right)^3-1^{2019}=5\cdot4^2\)
\(\Leftrightarrow3\left(x+2\right)^3=5\cdot16+1=81\)
\(\Leftrightarrow x+2=3\)
hay x=1
MH
12 tháng 9 2021
\(4^{15}.9^{15}< 2^n.3^n< 18^{16}.2^{16}\)
⇒\(\left(4.9\right)^{15}< \left(2.3\right)^n< \left(18.2\right)^{16}\)
⇒\(\left(6^2\right)^{15}< 6^n< \left(6^2\right)^{16}\)
⇒\(6^{30}< 6^n< 6^{32}\)
⇒\(6^n=6^{31}\)
⇒n=31
12 tháng 9 2021
\(4^{15}\cdot9^{15}< 2^n\cdot3^n< 18^{16}\cdot2^{16}\\ \Leftrightarrow\left(4\cdot9\right)^{15}< \left(2\cdot3\right)^n< \left(18\cdot2\right)^{16}\\ \Leftrightarrow36^{15}< 6^n< 36^{16}\\ \Leftrightarrow6^{30}< 6^n< 6^{32}\\ \Leftrightarrow n=31\)
TM
0
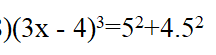
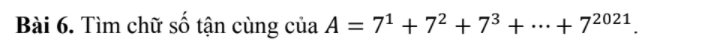
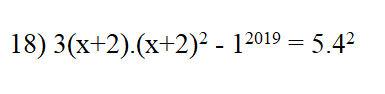
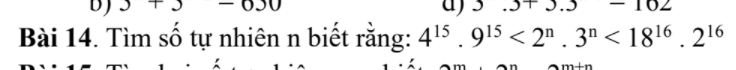
tách ra
ko nổi