chứng minh giá trị biểu thức không phụ thuộc vào các biến:
a, với a>0 và I a I > I b I , A = \(\frac{\sqrt{a^3}+a}{a^2+\sqrt{a^5}}.\left(\frac{b^2}{a-\sqrt{a^2-b^2}}+\frac{b^2}{a+\sqrt{a^2-b^2}}\right)\)
b, với a, b > 0 , B = \(\frac{a+b\sqrt{a}}{b-a}.\sqrt{\frac{ab+a^2-2\sqrt{a^3b}}{b^2+2b\sqrt{a}+a}}\)

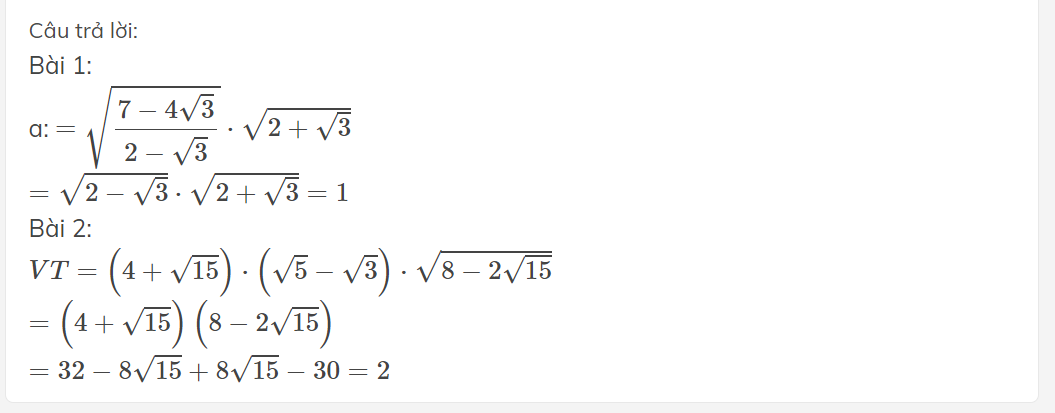
a/ A=2
b/ B = a/ (√a + √b)