Giải phương trình \(\dfrac{x^2-3x+5}{\left(x-3\right)\left(x+2\right)}=\dfrac{1}{x-3}\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


1a.
ĐKXĐ: \(x\ne\left\{1;3\right\}\)
\(\Leftrightarrow\dfrac{6}{x-1}=\dfrac{4}{x-3}+\dfrac{4}{x-3}\)
\(\Leftrightarrow\dfrac{3}{x-1}=\dfrac{4}{x-3}\Leftrightarrow3\left(x-3\right)=4\left(x-1\right)\)
\(\Leftrightarrow3x-9=4x-4\Rightarrow x=-5\)
b.
ĐKXĐ: \(x\ne\left\{-1;2\right\}\)
\(\Leftrightarrow\dfrac{5}{x+1}=\dfrac{3}{2-x}+\dfrac{1}{2-x}\)
\(\Leftrightarrow\dfrac{5}{x+1}=\dfrac{4}{2-x}\Leftrightarrow5\left(2-x\right)=4\left(x+1\right)\)
\(\Leftrightarrow10-2x=4x+4\Leftrightarrow6x=6\Rightarrow x=1\)
1c.
ĐKXĐ: \(x\ne\left\{2;5\right\}\)
\(\Leftrightarrow\dfrac{3x\left(x-5\right)}{\left(x-2\right)\left(x-5\right)}-\dfrac{x\left(x-2\right)}{\left(x-2\right)\left(x-5\right)}=\dfrac{-3x}{\left(x-2\right)\left(x-5\right)}\)
\(\Leftrightarrow3x\left(x-5\right)-x\left(x-2\right)=-3x\)
\(\Leftrightarrow2x^2-10x=0\Leftrightarrow2x\left(x-5\right)=0\Rightarrow\left[{}\begin{matrix}x=0\\x=5\left(loại\right)\end{matrix}\right.\)
2a.
\(\Leftrightarrow-4x^2-5x+6=x^2+4x+4\)
\(\Leftrightarrow5x^2+9x-2=0\Rightarrow\left[{}\begin{matrix}x=-2\\x=\dfrac{1}{5}\end{matrix}\right.\)
2b.
\(2x^2-6x+1=0\Rightarrow x=\dfrac{3\pm\sqrt{7}}{2}\)

\(i.\dfrac{\left(2x+1\right)^2}{5}-\dfrac{\left(x-1\right)^2}{3}=\dfrac{7x^2-14x-5}{15}\)
\(\Leftrightarrow\dfrac{4x^2+4x+1}{5}-\dfrac{x^2-2x+1}{3}=\dfrac{7x^2-14x-5}{15}\)
\(\Leftrightarrow\dfrac{12x^2+12x+3}{15}-\dfrac{5x^2-10x+5}{15}=\dfrac{7x^2-14x-5}{15}\)
\(\Leftrightarrow12x^2+12x+3-5x^2+10x-5=7x^2-14x-5\)
\(\Leftrightarrow36x=-3\)
\(\Leftrightarrow x=-\dfrac{1}{12}\)

a. \(x-\dfrac{x+2}{3}< 3x+\dfrac{x}{2}+5\)
\(\Leftrightarrow\dfrac{6x}{6}-\dfrac{2\left(x+2\right)}{6}< \dfrac{18x}{6}+\dfrac{3x}{6}+\dfrac{30}{6}\)
\(\Rightarrow6x-2x-4-18x-3x-30< 0\)
\(\Leftrightarrow-17x< 34\)
\(\Leftrightarrow x>-2\)
b. \(\dfrac{x}{2}+\dfrac{1-x}{3}>0\)
\(\Leftrightarrow3x+2-2x>0\)
\(\Leftrightarrow x>-2\)
c. \(\left(x-9\right)^2-x\left(x+9\right)< 0\)
\(\Leftrightarrow x^2-18x+81-x^2-9x< 0\)
\(\Leftrightarrow-27x< -81\)
\(\Leftrightarrow x>3\)

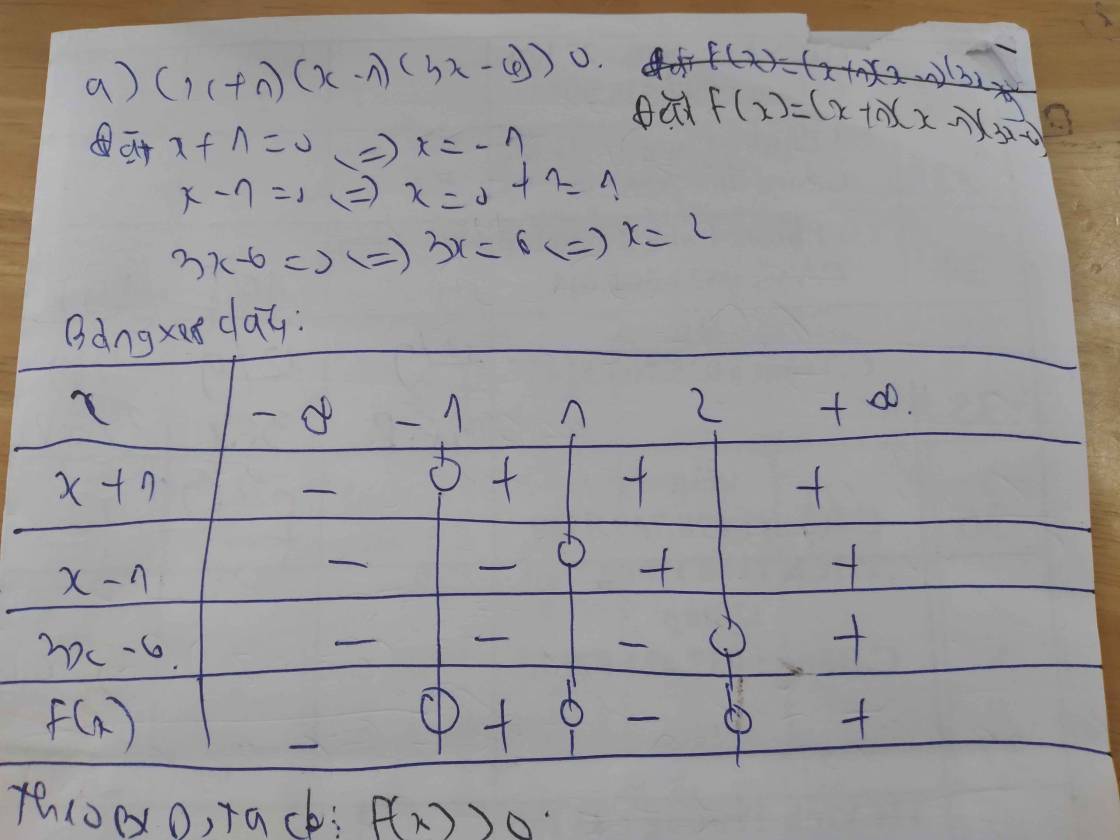
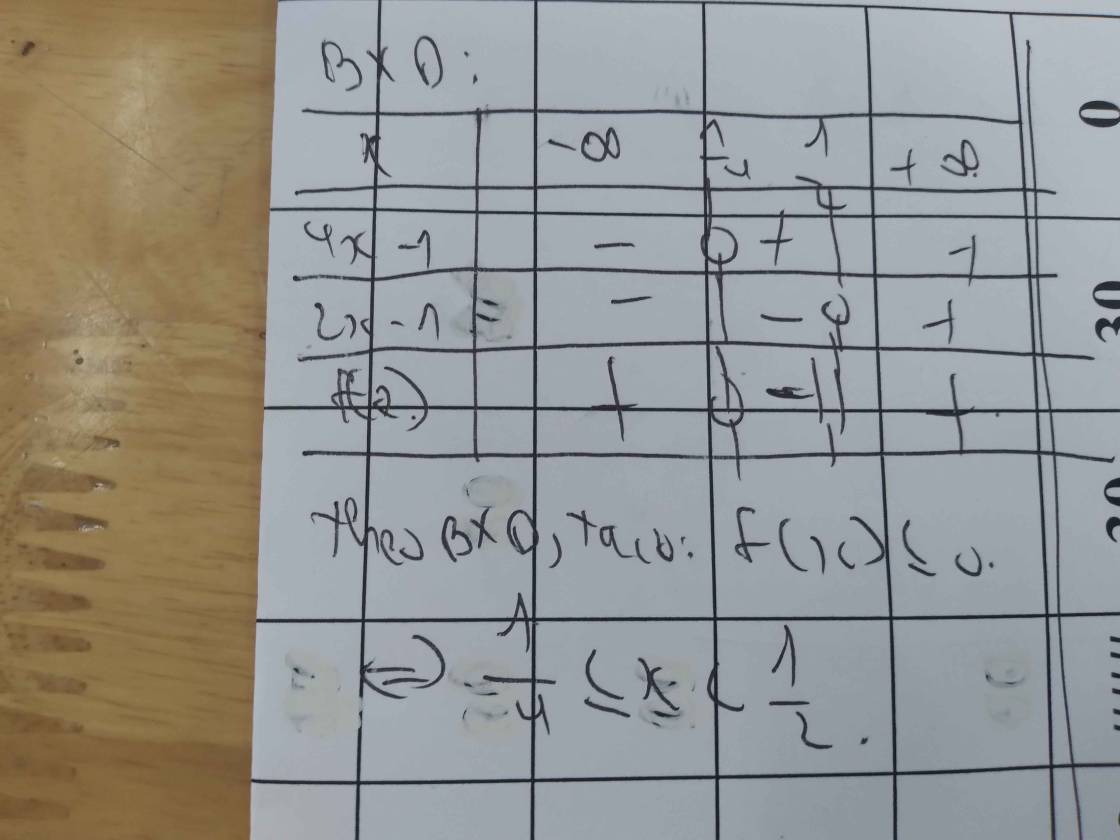
a) \(\left(x+1\right)\left(x-1\right)\left(3x-6\right)>0\)
Lập bảng xét dấu ta được kết quả :
\(Bpt\Leftrightarrow\left[{}\begin{matrix}-1< x< 1\\x>2\end{matrix}\right.\)
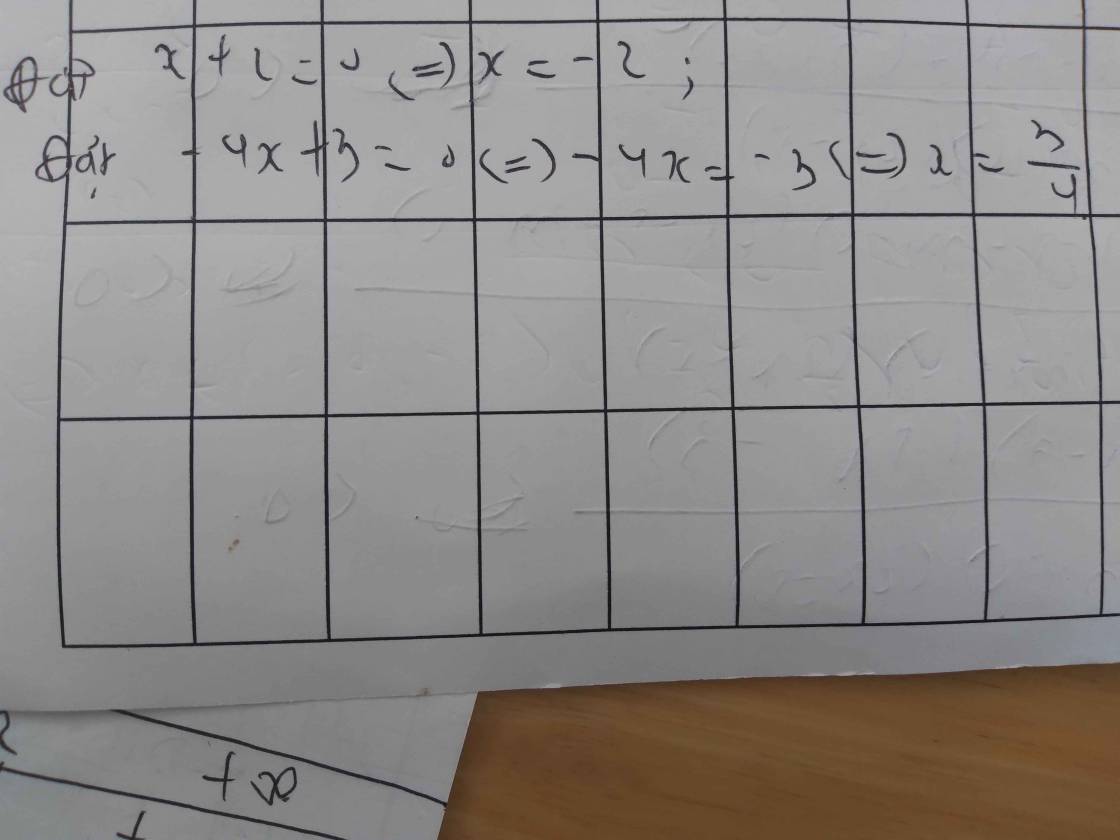
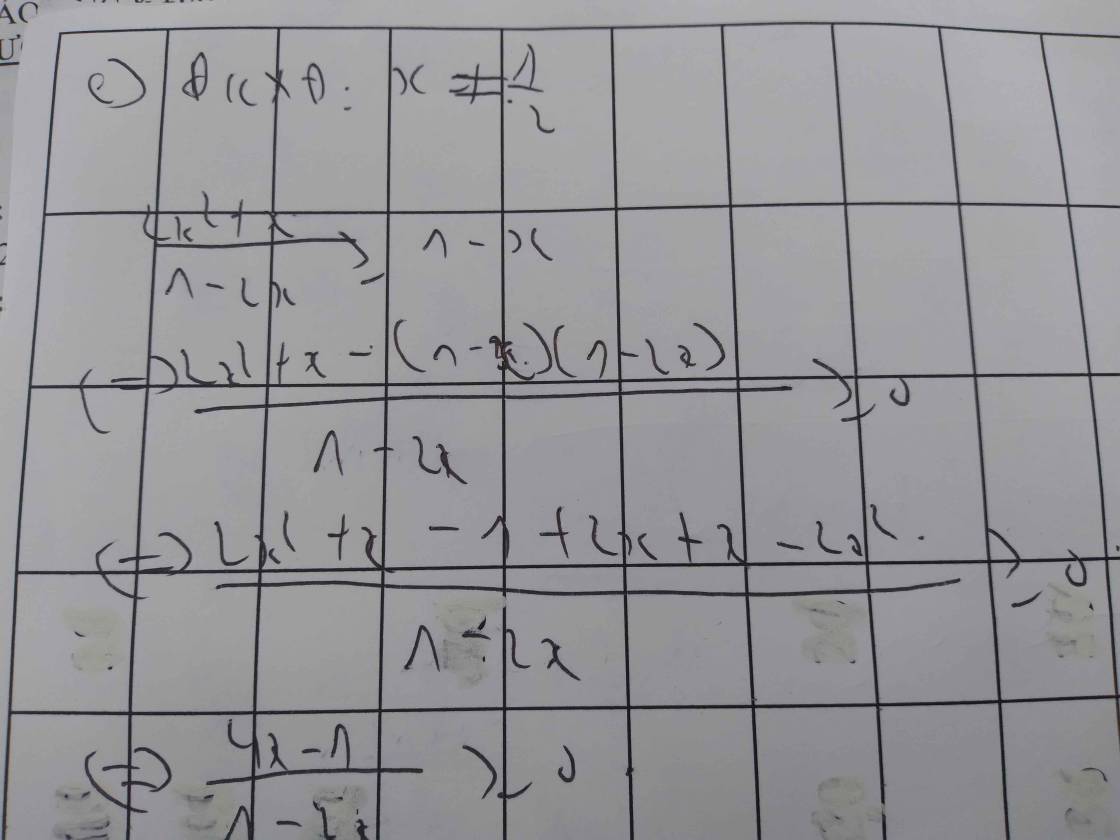
b) \(\dfrac{x+3}{x-2}\le0\)
Lập bảng xét dấu ta được kết quả :
\(Bpt\Leftrightarrow-3\le x< 2\)
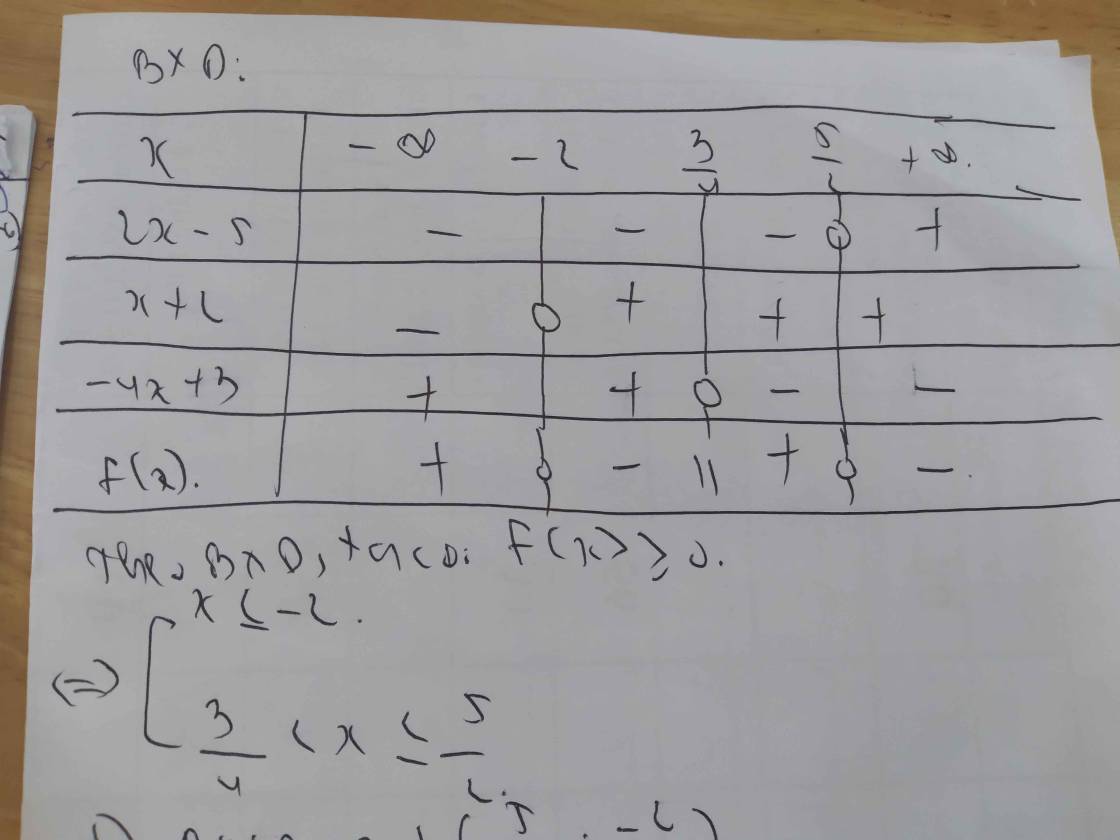
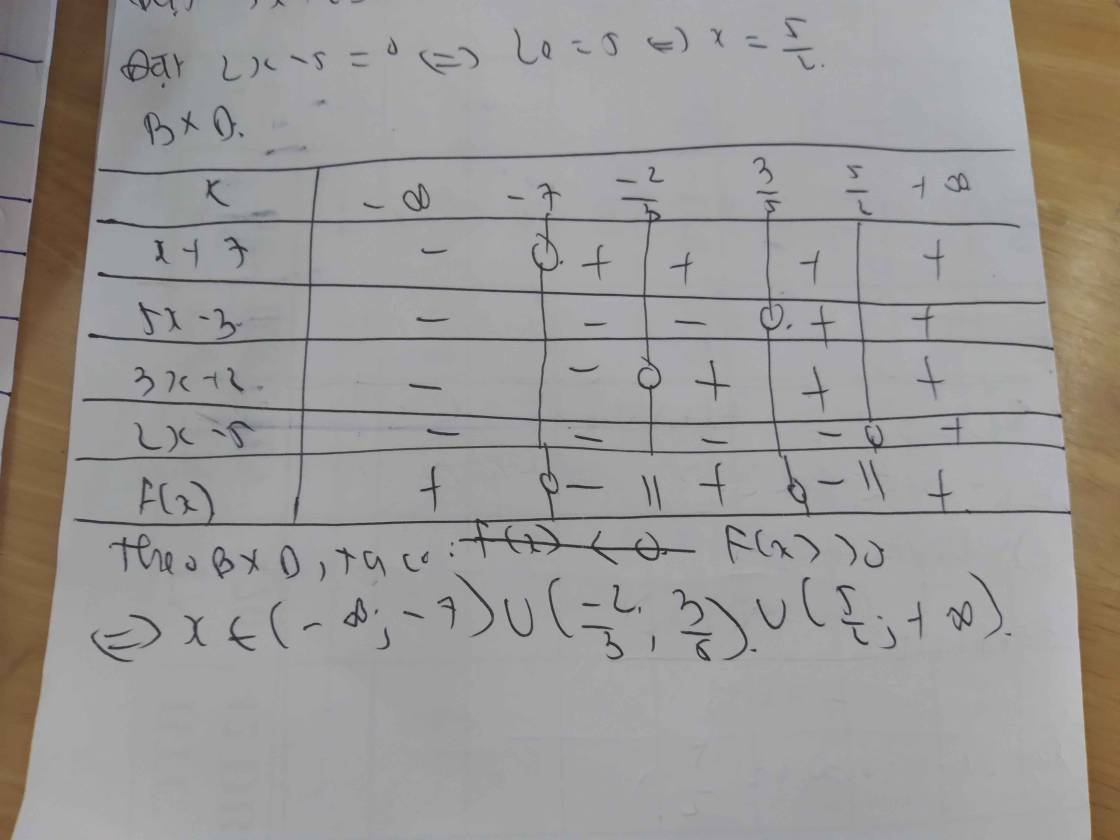
d) \(\dfrac{2x-5}{3x+2}< \dfrac{3x+2}{2x-5}\)
\(\Leftrightarrow\dfrac{2x-5}{3x+2}-\dfrac{3x+2}{2x-5}< 0\)
\(\Leftrightarrow\dfrac{\left(2x-5\right)^2-\left(3x+2\right)^2}{\left(3x+2\right)\left(2x-5\right)}< 0\)
\(\Leftrightarrow\dfrac{\left(2x-5+3x+2\right)\left(2x-5-3x-2\right)}{\left(3x+2\right)\left(2x-5\right)}< 0\)
\(\Leftrightarrow\dfrac{-\left(5x-3\right)\left(x+7\right)}{\left(3x+2\right)\left(2x-5\right)}< 0\)
Lập bảng xét dấu ta được kết quả :
\(Bpt\Leftrightarrow\left[{}\begin{matrix}-7< x< -\dfrac{2}{3}\\\dfrac{5}{3}< x< \dfrac{5}{2}\end{matrix}\right.\)

Với \(x=0\) không phải nghiệm
Với \(x\ne0\) chia 2 vế cho \(x^2\), pt tương đương:
\(2x^2+3x-1+\dfrac{3}{x}+\dfrac{2}{x^2}=0\)
\(\Leftrightarrow2\left(x+\dfrac{1}{x}\right)^2+3\left(x+\dfrac{1}{x}\right)-5=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x+\dfrac{1}{x}=1\\x+\dfrac{1}{x}=-\dfrac{5}{2}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x^2-x+1=0\\2x^2+5x+2=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\left(x-\dfrac{1}{2}\right)^2+\dfrac{3}{4}=0\left(vô-nghiệm\right)\\\left(x+2\right)\left(2x+1\right)=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=-\dfrac{1}{2}\end{matrix}\right.\)
Câu a chắc là đề sai, vì nghiệm vô cùng xấu, tử số của phân thức cuối cùng là \(x+17\) mới hợp lý
b.
Đặt \(x+3=t\)
\(\Rightarrow\left(t+1\right)^4+\left(t-1\right)^4=14\)
\(\Leftrightarrow t^4+6t^2-6=0\) (đến đây đoán rằng bạn tiếp tục ghi sai đề, nhưng thôi cứ giải tiếp)
\(\Rightarrow\left[{}\begin{matrix}t^2=-3+\sqrt{15}\\t^2=-3-\sqrt{15}\left(loại\right)\end{matrix}\right.\)
\(\Rightarrow t=\pm\sqrt{-3+\sqrt{15}}\Rightarrow x=-3\pm\sqrt{-3+\sqrt{15}}\)
Câu c chắc cũng sai đề, vì lên lớp 8 rồi không ai cho đề kiểu này cả, người ta sẽ rút gọn luôn số 1 bên trái và 60 bên phải.

a: Ta có: \(4x-2\left(1-x\right)=5\left(x-4\right)\)
\(\Leftrightarrow4x-2+2x=5x-20\)
\(\Leftrightarrow x=-18\)
b: Ta có: \(\dfrac{x}{6}+\dfrac{1-3x}{9}=\dfrac{-x+1}{12}\)
\(\Leftrightarrow6x+4\left(1-3x\right)=3\left(-x+1\right)\)
\(\Leftrightarrow6x+4-12x=-3x+3\)
\(\Leftrightarrow-3x=-1\)
hay \(x=\dfrac{1}{3}\)
c: Ta có: \(\left(x+2\right)^2-3\left(x+2\right)=0\)
\(\Leftrightarrow\left(x+2\right)\left(x-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=-2\\x=1\end{matrix}\right.\)

a) Ta có: \(\left(2x-3\right)^2=\left(2x-3\right)\left(x+1\right)\)
\(\Leftrightarrow\left(2x-3\right)^2-\left(2x-3\right)\left(x+1\right)=0\)
\(\Leftrightarrow\left(2x-3\right)\left(2x-3-x-1\right)=0\)
\(\Leftrightarrow\left(2x-3\right)\left(x-4\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}2x-3=0\\x-4=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}2x=3\\x=4\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{3}{2}\\x=4\end{matrix}\right.\)
Vậy: \(S=\left\{\dfrac{3}{2};4\right\}\)
b) Ta có: \(x\left(2x-9\right)=3x\left(x-5\right)\)
\(\Leftrightarrow x\left(2x-9\right)-3x\left(x-5\right)=0\)
\(\Leftrightarrow x\left(2x-9\right)-x\left(3x-15\right)=0\)
\(\Leftrightarrow x\left(2x-9-3x+15\right)=0\)
\(\Leftrightarrow x\left(6-x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\6-x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=0\\x=6\end{matrix}\right.\)
Vậy: S={0;6}
c) Ta có: \(3x-15=2x\left(x-5\right)\)
\(\Leftrightarrow3\left(x-5\right)-2x\left(x-5\right)=0\)
\(\Leftrightarrow\left(x-5\right)\left(3-2x\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x-5=0\\3-2x=0\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\2x=3\end{matrix}\right.\Leftrightarrow\left[{}\begin{matrix}x=5\\x=\dfrac{3}{2}\end{matrix}\right.\)
Vậy: \(S=\left\{5;\dfrac{3}{2}\right\}\)
d) Ta có: \(\dfrac{5-x}{2}=\dfrac{3x-4}{6}\)
\(\Leftrightarrow6\left(5-x\right)=2\left(3x-4\right)\)
\(\Leftrightarrow30-6x=6x-8\)
\(\Leftrightarrow30-6x-6x+8=0\)
\(\Leftrightarrow-12x+38=0\)
\(\Leftrightarrow-12x=-38\)
\(\Leftrightarrow x=\dfrac{19}{6}\)
Vậy: \(S=\left\{\dfrac{19}{6}\right\}\)
e) Ta có: \(\dfrac{3x+2}{2}-\dfrac{3x+1}{6}=2x+\dfrac{5}{3}\)
\(\Leftrightarrow\dfrac{3\left(3x+2\right)}{6}-\dfrac{3x+1}{6}=\dfrac{12x}{6}+\dfrac{10}{6}\)
\(\Leftrightarrow6x+4-3x-1=12x+10\)
\(\Leftrightarrow3x+3-12x-10=0\)
\(\Leftrightarrow-9x-7=0\)
\(\Leftrightarrow-9x=7\)
\(\Leftrightarrow x=-\dfrac{7}{9}\)
Vậy: \(S=\left\{-\dfrac{7}{9}\right\}\)

a: =>3x-9+5+10x=90
=>13x-4=90
=>13x=94
hay x=94/13
b: \(\Leftrightarrow2x-4-x-1=3x-11\)
=>3x-11=x-5
=>2x=6
hay x=3(nhận)

`a,` \(\dfrac{5x+2}{6}-\dfrac{8x-1}{3}=\dfrac{4x+2}{5}-5\)
`<=> (5(5x+2))/30 - (10(8x-1))/30 = (6(4x+2))/30 - (5.30)/30`
`<=> 5(5x+2) - 10(8x-1) =6(4x+2) - 5.30`
`<=> 25x + 10 - 80x + 10 = 24x+12 - 150`
`<=> -55x +20 = 24x-138`
`<=> -55x -24x=-138-20`
`<=>-79x=-158`
`<=> x=2`
Vậy pt có nghiệm `x=2`
`b,` \(\dfrac{x+2}{x-2}-\dfrac{1}{x}=\dfrac{2}{x\left(x-2\right)}\)
ĐKXĐ : \(\left\{{}\begin{matrix}x-2\ne0\\x\ne0\end{matrix}\right.\Leftrightarrow\left\{{}\begin{matrix}x\ne2\\x\ne0\end{matrix}\right.\)
Ta có : `(x+2)/(x-2) -1/x = 2/(x(x-2))`
`<=> (x(x+2))/(x(x-2)) - (x-2)/(x(x-2)) = 2/(x(x-2))`
`=> x^2 +2x - x +2 = 2`
`<=> x^2 + x =0`
`<=>x(x+1)=0`
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x+1=0\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\left(l\right)\\x=-1\end{matrix}\right.\)
Vậy pt có nghiệm `x=-1`
`c,2x^3 + 6x^2 =x^2 +3x`
`<=> 2x^3 + 6x^2 -x^2 -3x=0`
`<=> 2x^3 + 5x^2 -3x=0`
`->` Đề có sai ko ạ ?
`d,` \(\left|x-4\right|+3x=5\) `(1)`
Thường hợp `1` : `x-4 >= 0<=> x >=0` thì phương trình `(1)` thở thành :
`x-4 = 5-3x`
`<=> x+3x=5+4`
`<=> 4x=9`
`<=> x= 9/4 (t//m)`
Trường hợp `2` : `x-4< 0<=> x<0` thì phương trình `(1)` trở thành :
`-(x-4) =5-3x`
`<=> -x +4=5-3x`
`<=> -x+3x=5-4`
`<=> 2x =1`
`<=>x=1/2 ( kt//m)`
Vậy phương trình có nghiệm `x=9/4`



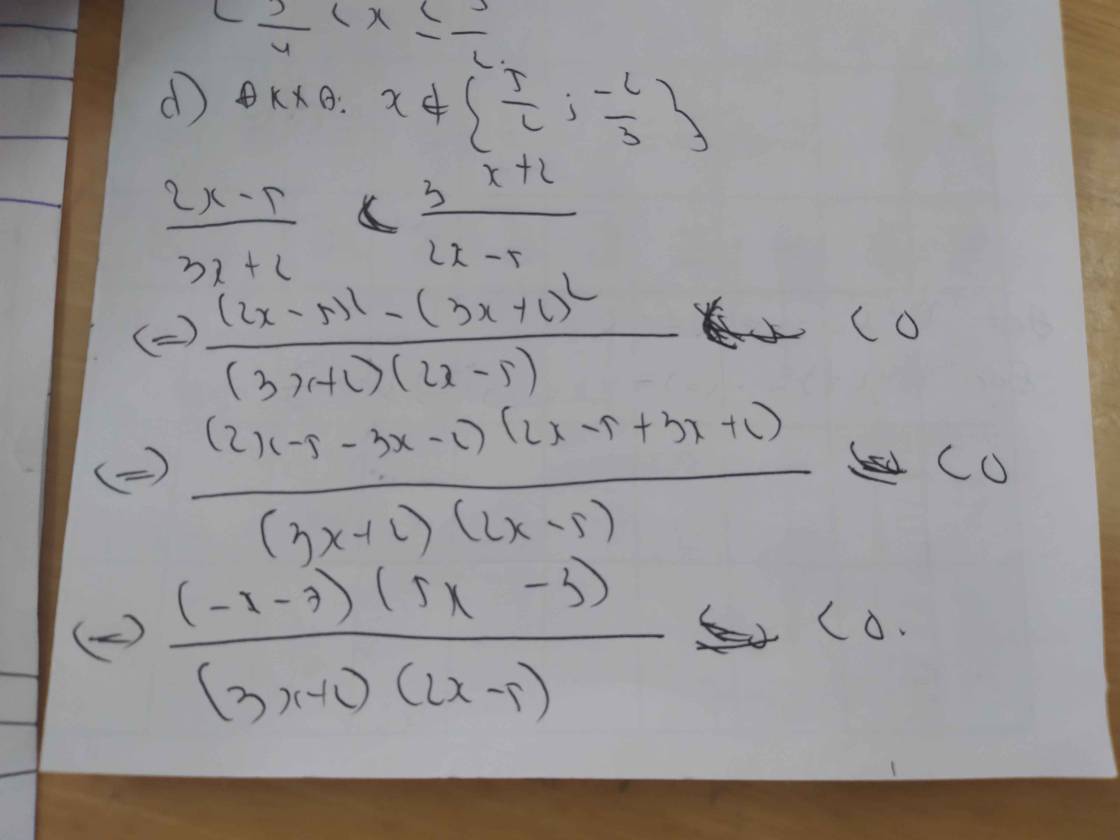


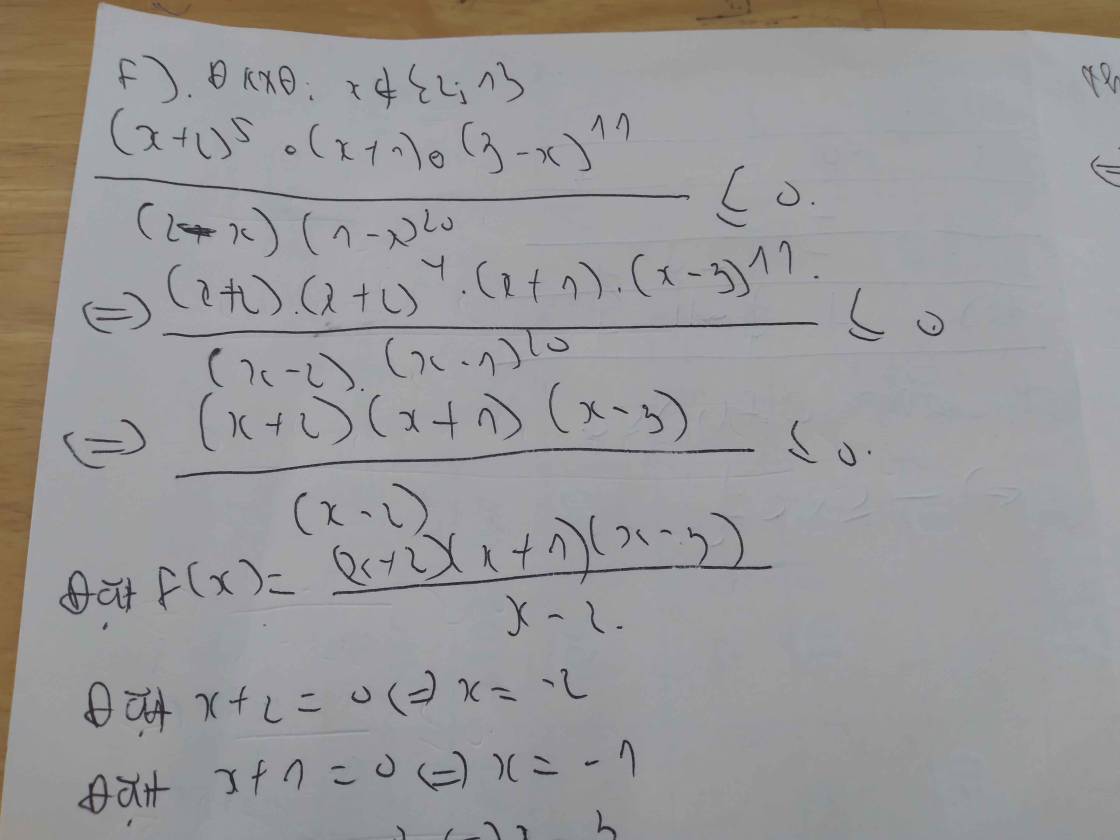
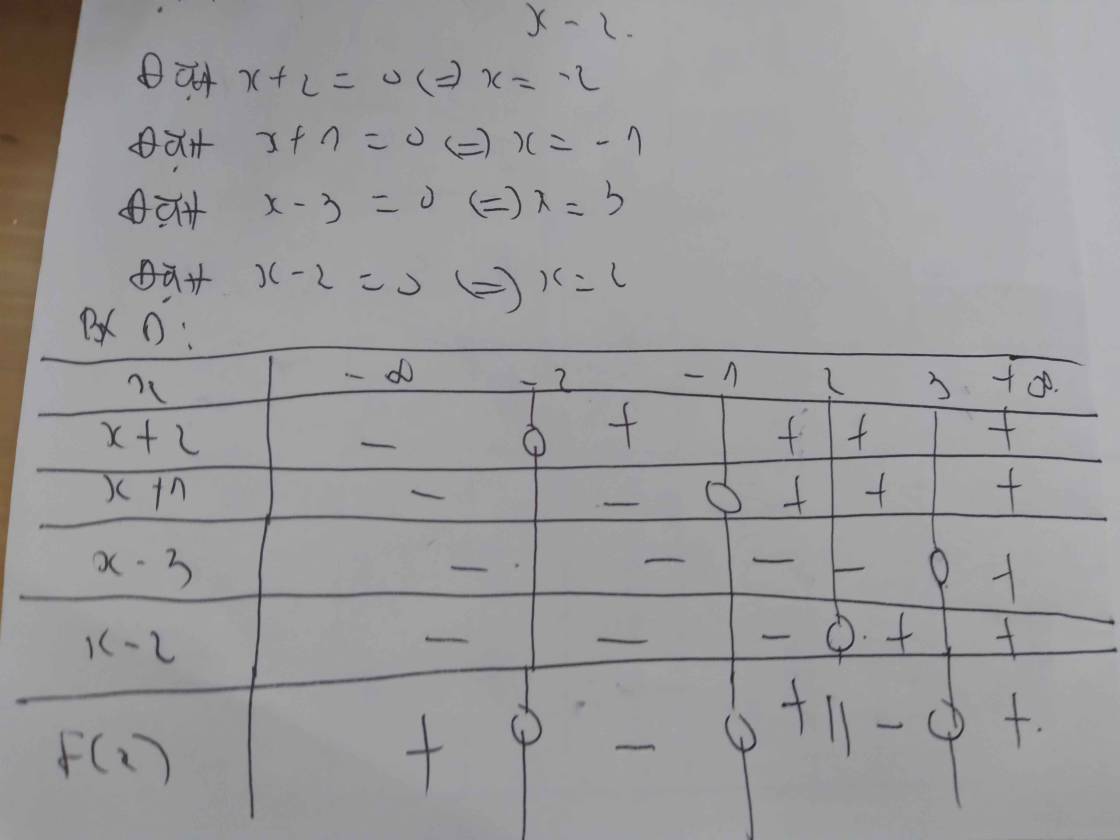

ĐKXĐ : \(\hept{\begin{cases}x\ne3\\x\ne-2\end{cases}}\)
<=> \(\frac{x^2-3x+5}{\left(x-3\right)\left(x+2\right)}-\frac{x+2}{\left(x-3\right)\left(x+2\right)}=0\)
<=> \(\frac{x^2-4x+3}{\left(x-3\right)\left(x+2\right)}=0\)
=> x2 - 4x + 3 = 0
Δ' = b'2 - ac = (-2)2 - 3 = 1
Δ' > 0, áp dụng công thức nghiệm thu được x1 = 3 (ktm) ; x2 = 1 (tm)
Vậy pt có nghiệm x = 1