giải dùm e mấy câu này với ạ
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


\(2^{10}:64\cdot16\)
\(=2^{10}:2^6\cdot2^4\)
\(=2^{10-6+4}\)
\(=2^8\)
\(2^{10}.64.16\\ =2^{10}.2^6.2^4\\ =2^{10+6+4}=2^{20}\)


1. have done
2. has written/ has not finished
3. left/ have never met
4. have you had...?
5. did you do/ did you play
6. bought/ has not worn
7. has taught/ graduated
8. Have you heard...?/ has been/ Have you read...?
9. got/ was/ went
10. earned/ has already spent

Bài 5:
a: 2x-(3-5x)=4(x+3)
=>2x-3+5x=4x+12
=>7x-3=4x+12
=>3x=15
=>x=5
b: =>5/3x-2/3+x=1+5/2-3/2x
=>25/6x=25/6
=>x=1
c: 3x-2=2x-3
=>3x-2x=-3+2
=>x=-1
d: =>2u+27=4u+27
=>u=0
e: =>5-x+6=12-8x
=>-x+11=12-8x
=>7x=1
=>x=1/7
f: =>-90+12x=-45+6x
=>12x-90=6x-45
=>6x-45=0
=>x=9/2

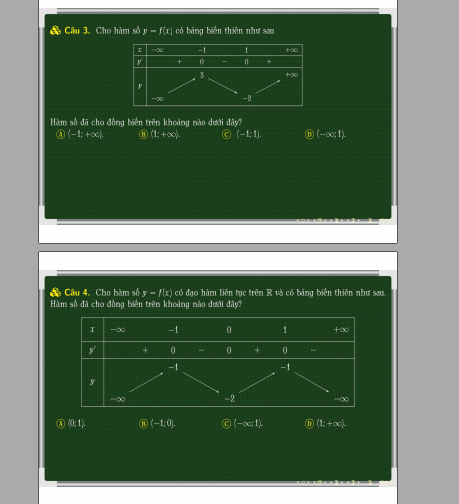
3.
Từ BBT ta thấy hàm đồng biến trên các khoảng \(\left(-\infty;-1\right)\) và \(\left(1;+\infty\right)\)
B đúng
4.
Từ BBT ta thấy hàm đồng biến trên các khoảng \(\left(-\infty;-1\right)\) và \(\left(0;1\right)\)
A đúng
1.
B sai (thiếu điều kiện \(f'\left(x\right)=0\) tại hữu hạn điểm)

11. \(I=\int\limits^2_1x\sqrt{x^2+1}dx\)
Đặt \(\sqrt{x^2+1}=t\Leftrightarrow x^2=t^2-1\Rightarrow xdx=tdt\) ; \(\left\{{}\begin{matrix}x=1\Rightarrow t=\sqrt{2}\\x=2\Rightarrow t=\sqrt{5}\end{matrix}\right.\)
\(I=\int\limits^{\sqrt{5}}_{\sqrt{2}}t.tdt=\int\limits^{\sqrt{5}}_{\sqrt{2}}t^2dt=\dfrac{1}{3}t^3|^{\sqrt{5}}_{\sqrt{2}}=\dfrac{1}{3}\left(5\sqrt{5}-2\sqrt{2}\right)\)
12. Đặt \(\sqrt[3]{8-4x}=t\Rightarrow x=\dfrac{8-t^3}{4}\Rightarrow dx=-\dfrac{3}{4}t^2dt\) ; \(\left\{{}\begin{matrix}x=0\Rightarrow t=2\\x=2\Rightarrow t=0\end{matrix}\right.\)
\(I=\int\limits^0_2t.\left(-\dfrac{3}{4}t^2dt\right)=\dfrac{3}{4}\int\limits^2_0t^3dt=\dfrac{3}{16}t^4|^2_0=3\)
13. Đặt \(\sqrt{3-2x}=t\Rightarrow x=\dfrac{3-t^2}{2}\Rightarrow dx=-tdt\) ; \(\left\{{}\begin{matrix}x=0\Rightarrow t=\sqrt{3}\\x=1\Rightarrow t=1\end{matrix}\right.\)
\(I=\int\limits^1_{\sqrt{3}}\dfrac{-tdt}{t}=\int\limits^{\sqrt{3}}_1dt=t|^{\sqrt{3}}_1=\sqrt{3}-1\)





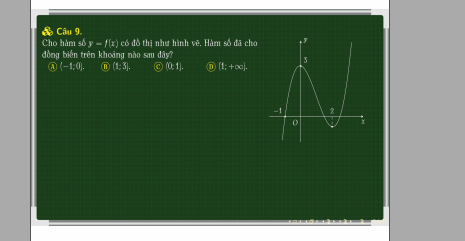







Câu 18: B
Câu 19: A
18B
19A
20D