Giải phương trình
\(x^4+2x^3+5x^2+4x+4=0\)
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


2x3 + 5x2 – 3x = 0
⇔ x(2x2 + 5x – 3) = 0
⇔ x.(2x2 + 6x – x – 3) = 0
⇔ x. [2x(x + 3) – (x + 3)] = 0
⇔ x.(2x – 1)(x + 3) = 0
⇔ x = 0 hoặc 2x – 1 = 0 hoặc x + 3 = 0
+ 2x – 1 = 0 ⇔ 2x = 1 ⇔ x = 1/2.
+ x + 3 = 0 ⇔ x = -3.
Vậy phương trình có tập nghiệm 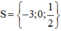


d: Ta có: \(\left(x+1\right)\left(x+2\right)\left(x+3\right)\left(x+4\right)=24\)
\(\Leftrightarrow\left(x^2+5x+4\right)\left(x^2+5x+6\right)-24=0\)
\(\Leftrightarrow x\left(x+5\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}x=0\\x=-5\end{matrix}\right.\)

\(5x^2+4x+2x^3+x^4-12=0\)
\(\Leftrightarrow x^4+2x^3+5x^2+4x-12=0\)
\(\Leftrightarrow x^4-x^3+3x^3-3x^2+8x^2-8x+12x-12=0\)
\(\Leftrightarrow x^3\left(x-1\right)+3x^2\left(x-1\right)+8x\left(x-1\right)+12\left(x-1\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x^3+3x^2+8x+12\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left[x^3+2x^2+x^2+2x+6x+12\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left[x^2\left(x+2\right)+x\left(x+2\right)+6\left(x+2\right)\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left(x^2+x+6\right)=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left[x^2+2\times\dfrac{1}{2}x+\left(\dfrac{1}{2}\right)^2-\left(\dfrac{1}{2}\right)^2+6\right]=0\)
\(\Leftrightarrow\left(x-1\right)\left(x+2\right)\left[\left(x+\dfrac{1}{2}\right)^2+\dfrac{23}{4}\right]\)
\(\Rightarrow\left[{}\begin{matrix}x-1=0\\x+2=0\\\left(x^2+\dfrac{1}{2}\right)^2+\dfrac{23}{4}=0\end{matrix}\right.\Rightarrow\left[{}\begin{matrix}x=1\\x=-2\end{matrix}\right.\)
Vì \(\left(x^2+\dfrac{1}{2}\right)^2\ge0\forall x\Rightarrow\left(x^2+\dfrac{1}{2}\right)^2+\dfrac{23}{4}\ge\dfrac{23}{4}\forall x\)
\(\Rightarrow\left(x^2+\dfrac{1}{2}\right)^2+\dfrac{23}{4}\) vô nghiệm
Vậy phương trình có tập nghiệm là\(S=\left\{1;-2\right\}\)

Chứng minh rằng phương trình sau có ít nhất hai nghiệm :
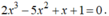
- Xét hàm số: f ( x ) = 2 x 3 - 5 x 2 + x + 1 là hàm đa thức.
⇒ Hàm số f liên tục trên R.
- Ta có:
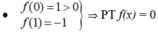 có ít nhất một nghiệm c1 ∈ (0;1).
có ít nhất một nghiệm c1 ∈ (0;1).
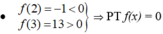 có ít nhất một nghiệm c2 ∈ (2;3).
có ít nhất một nghiệm c2 ∈ (2;3).
- Mà c ≠ c 2 nên PT f(x) = 0 có ít nhất 2 nghiệm.

a: P(x)=6x^3-4x^2+4x-2
Q(x)=-5x^3-10x^2+6x+11
M(x)=x^3-14x^2+10x+9
b: \(C\left(x\right)=7x^4-4x^3-6x+9+3x^4-7x^3-5x^2-9x+12\)
=10x^4-11x^3-5x^2-15x+21

\(a,x^2-6x+5=0\\ \Rightarrow\left(x^2-5x\right)-\left(x-5\right)=0\\ \Rightarrow x\left(x-5\right)-\left(x-5\right)=0\\ \Rightarrow\left(x-1\right)\left(x-5\right)=0\\ \Rightarrow\left[{}\begin{matrix}x=1\\x=5\end{matrix}\right.\)
\(b,2x^2+4x-8=0\\ \Rightarrow x^2+2x-4=0\\ \Rightarrow\left(x^2+2x+1\right)-5=0\\ \Rightarrow\left(x+1\right)^2-\sqrt{5^2}=0\\ \Rightarrow\left(x+1+\sqrt{5}\right)\left(x+1-\sqrt{5}\right)=0\\ \Rightarrow\left[{}\begin{matrix}x=-1-\sqrt{5}\\x=-1+\sqrt{5}\end{matrix}\right.\)
\(c,4y^2-4y+1=0\\ \Rightarrow\left(2y-1\right)^2=0\\ \Rightarrow2y-1=0\\ \Rightarrow y=\dfrac{1}{2}\)
\(d,5x^2-x+2=0\)
Ta có:\(\Delta=\left(-1\right)^2-4.5.2=1-40=-39\)
Vì \(\Delta< 0\Rightarrow\) pt vô nghiệm
<=>\(\left(x^2\right)^2+\left(x\right)^2+\left(2\right)^2+2x\cdot x^2+2.x.2+2.x^2\cdot2=0\)
<=> \(\left(x^2+x+2\right)^2=0\Leftrightarrow x^2+x+2=0\left(vn\right)\)
Vậy pt đã cho vn
bài này vô nghiệm bạn à