Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
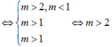
a, ĐK: \(x\le-1,x\ge3\)
\(pt\Leftrightarrow2\left(x^2-2x-3\right)+\sqrt{x^2-2x-3}-3=0\)
\(\Leftrightarrow\left(2\sqrt{x^2-2x-3}+3\right).\left(\sqrt{x^2-2x-3}-1\right)=0\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{x^2-2x-3}=-\dfrac{3}{2}\left(l\right)\\\sqrt{x^2-2x-3}=1\end{matrix}\right.\)
\(\Leftrightarrow x^2-2x-3=1\)
\(\Leftrightarrow x^2-2x-4=0\)
\(\Leftrightarrow x=1\pm\sqrt{5}\left(tm\right)\)
b, ĐK: \(-2\le x\le2\)
Đặt \(\sqrt{2+x}-2\sqrt{2-x}=t\Rightarrow t^2=10-3x-4\sqrt{4-x^2}\)
Khi đó phương trình tương đương:
\(3t-t^2=0\)
\(\Leftrightarrow\left[{}\begin{matrix}t=0\\t=3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}\sqrt{2+x}-2\sqrt{2-x}=0\\\sqrt{2+x}-2\sqrt{2-x}=3\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}2+x=8-4x\\2+x=17-4x+12\sqrt{2-x}\end{matrix}\right.\)
\(\Leftrightarrow\left[{}\begin{matrix}x=\dfrac{6}{5}\left(tm\right)\\5x-15=12\sqrt{2-x}\left(1\right)\end{matrix}\right.\)
Vì \(-2\le x\le2\Rightarrow5x-15< 0\Rightarrow\left(1\right)\) vô nghiệm
Vậy phương trình đã cho có nghiệm \(x=\dfrac{6}{5}\)
Bài 1:
c) ĐKXĐ: \(x\notin\left\{\dfrac{1}{4};-\dfrac{1}{4}\right\}\)
Ta có: \(\dfrac{3}{1-4x}=\dfrac{2}{4x+1}-\dfrac{8+6x}{16x^2-1}\)
\(\Leftrightarrow\dfrac{-3\left(4x+1\right)}{\left(4x-1\right)\left(4x+1\right)}=\dfrac{2\left(4x-1\right)}{\left(4x+1\right)\left(4x-1\right)}-\dfrac{6x+8}{\left(4x-1\right)\left(4x+1\right)}\)
Suy ra: \(-12x-3=8x-2-6x-8\)
\(\Leftrightarrow-12x-3-2x+10=0\)
\(\Leftrightarrow-14x+7=0\)
\(\Leftrightarrow-14x=-7\)
\(\Leftrightarrow x=\dfrac{1}{2}\)(nhận)
Vậy: \(S=\left\{\dfrac{1}{2}\right\}\)
\(\Delta'=\left(m-3\right)^2-\left(m^2+3\right)=-6m+6>0\Rightarrow m< 1\)
Theo hệ thức Viet: \(\left\{{}\begin{matrix}x_1+x_2=2\left(m-3\right)\\x_1x_2=m^2+3\end{matrix}\right.\)
\(x_1^2+x_2^2=86\)
\(\Leftrightarrow\left(x_1+x_2\right)^2-2x_1x_2=86\)
\(\Leftrightarrow4\left(m-3\right)^2-2\left(m^2+3\right)=86\)
\(\Leftrightarrow m^2-12m-28=0\Rightarrow\left[{}\begin{matrix}m=14\left(loại\right)\\m=-2\end{matrix}\right.\)
Ta có : \(\Delta=\left(2m+6\right)^2-4\left(m^2+3\right)=4m^2+24m+36-4m^2-12=24m+24\)
Để phương trình có 2 nghiệm phân biệt thì \(\Delta>0\)
\(24m+24>0\Leftrightarrow24m>-24\Leftrightarrow m>-1\)
Theo hệ thức Viet :\(\left\{{}\begin{matrix}x_1+x_2=-\dfrac{b}{a}=2m+6\\x_1x_2=\dfrac{c}{a}=m^2+3\end{matrix}\right.\)
mà \(\left(x_1+x_2\right)^2=\left(2m+6\right)^2\Leftrightarrow x_1^2+x_2^2=4m^2+24m+36-2x_1x_2\)
\(\Leftrightarrow x_1^2+x_2^2=4m^2+24m+36-2m^2-6=2m^2+24m+30\)
Lại có : \(x_1^2+x_2^2=86\)hay \(2m^2+24m+30=86\Leftrightarrow2\left(m^2+12m-28\right)=0\)
\(\Leftrightarrow2\left(m-2\right)\left(m+14\right)=0\Leftrightarrow\left[{}\begin{matrix}m=2\left(chon\right)\\m=-14\left(loại\right)\end{matrix}\right.\)
Câu a chắc lm đc chứ
b/ (x1 - 1)2 + (x2 - 1)2 = 4
=> x12 - 2x1 + 1 + x22 - 2x2 + 1 = 4
=> (x12 + x22) - 2(x1 + x2) - 2 = 0
=> (x1 + x2)2 - 2x1x2 - 2(x1 + x2) - 2 = 0
=> (2m - 2)2 - 2.3 - 2.(2m - 2) - 2 = 0
=> 4m2 - 8m + 4 - 6 - 4m + 4 - 2 = 0
=> 4m2 - 12m = 0
=> 4m(m - 3) = 0
=> 4m = 0 => m = 0
hoặc m - 3 = 0 => m = 3
Vậy m = 0 , m = 3
