biểu thức căn bặc hai của 2x+3 xác định khi nào
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.


a )
\(\sqrt{\frac{3}{4}-5x}\ge0\)
\(< =>\frac{3}{4}-5x\ge0\)
\(< =>-5x\ge-\frac{3}{4}\)
\(< =>\frac{-20x}{4}\ge-\frac{3}{4}\)
\(< =>-20x\ge-3\)
\(< =>x\ge\frac{3}{20}\)
\(\sqrt{\frac{-3}{1}-2x}\ge0\)
\(< =>-3-2x\ge0\)
\(< =>-2x\ge3\)
\(< =>x\ge-\frac{3}{2}\)

a: ĐKXĐ: x>=0; x<>1
b \(A=\left(\dfrac{2\sqrt{x}+x}{x\sqrt{x}-1}-\dfrac{1}{\sqrt{x}-1}\right):\dfrac{\sqrt{x}+2}{x+\sqrt{x}+1}\)
\(=\dfrac{x+2\sqrt{x}-x-\sqrt{x}-1}{x\sqrt{x}-1}\cdot\dfrac{x+\sqrt{x}+1}{\sqrt{x}+2}\)
\(=\dfrac{1}{\sqrt{x}+2}\)
c: Khi x=9-4 căn 5 thì \(A=\dfrac{1}{\sqrt{5}-2+2}=\dfrac{\sqrt{5}}{5}\)
d: căn x+2>=2
=>A<=1/2
Dấu = xảy ra khi x=0


Bài làm:
a) \(\sqrt{x^2-3x+2}=\sqrt{\left(x-1\right)\left(x-2\right)}\)
Ta xét 2 trường hợp sau:
Nếu: \(\hept{\begin{cases}x-1\ge0\\x-2\ge0\end{cases}\Rightarrow\hept{\begin{cases}x\ge1\\x\ge2\end{cases}\Rightarrow}}x\ge2\)
Nếu: \(\hept{\begin{cases}x-2\le0\\x-1\le0\end{cases}\Rightarrow}\hept{\begin{cases}x\le2\\x\le1\end{cases}\Rightarrow}x\le1\)
Vậy \(\orbr{\begin{cases}x\ge2\\x\le1\end{cases}}\)
b) \(\sqrt{2x^2+4x+5}=\sqrt{\left(x+2\right)^2+x^2+1}\)
Mà \(\left(x+2\right)^2+x^2+1>0\left(\forall x\right)\)
Vậy biểu thức xác đinh với mọi x
c) \(\sqrt{x^2+4x+5}=\sqrt{\left(x+2\right)^2+1}\)
Mà \(\left(x+2\right)^2+1>0\left(\forall x\right)\)
Vậy biểu thức xác định với mọi x
Học tốt!!!!
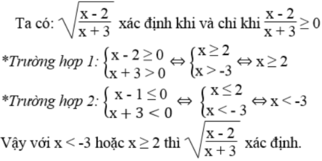
\(ĐK:2x+3\ge0\Leftrightarrow x\ge-\dfrac{3}{2}\)