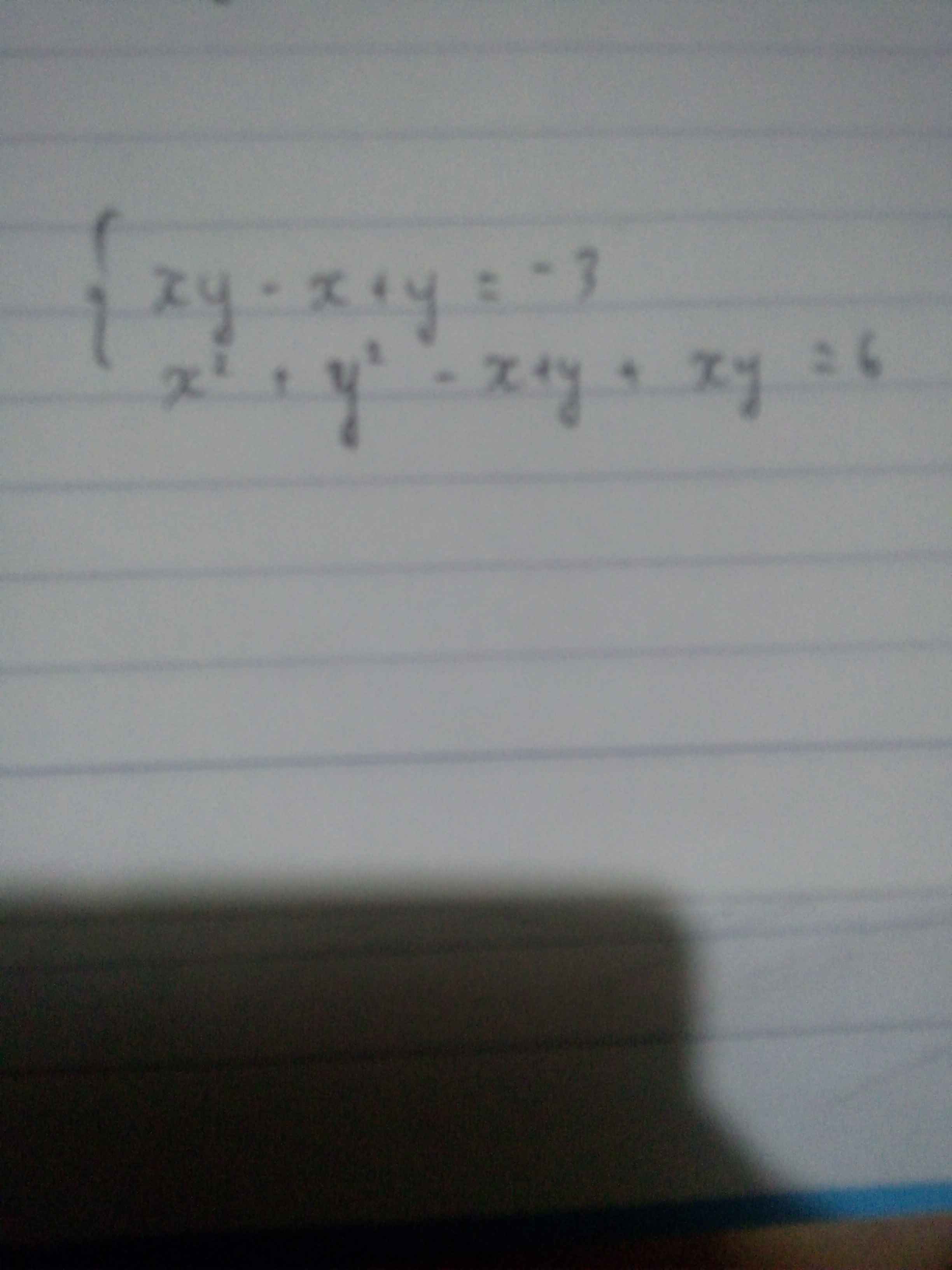\(x^2\)y-x+xy=6
K
Khách
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.
Những câu hỏi liên quan
NV
Nguyễn Việt Lâm
Giáo viên
21 tháng 9 2021
\(\Leftrightarrow\left\{{}\begin{matrix}3xy-3\left(x-y\right)=-9\\x^2+y^2+xy-\left(x-y\right)=6\end{matrix}\right.\)
Trừ vế cho vế:
\(x^2+y^2-2xy+2\left(x-y\right)=15\)
\(\Leftrightarrow\left(x-y\right)^2+2\left(x-y\right)-15=0\Rightarrow\left[{}\begin{matrix}x-y=3\\x-y=-5\end{matrix}\right.\)
\(\Rightarrow\left[{}\begin{matrix}y=x-3\\y=x+5\end{matrix}\right.\)
Thế vào pt đầu:
\(\Rightarrow\left[{}\begin{matrix}x\left(x-3\right)-x+x-3=-3\\x\left(x+5\right)-x+x+5=-3\end{matrix}\right.\)
\(\Leftrightarrow...\)
T
4
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
31 tháng 7 2023
a, x=1; y=2 => 12
x=2; y=1 => 21
b, x=1; y=5 => 15
x=5; y=1 => 51
GN
GV Nguyễn Trần Thành Đạt
Giáo viên
31 tháng 7 2023
c, x=1; y=6 => 16
x=6;y=1 => 61
x=2; y=3=> 23
x=3; y=2 => 32
d, x=1; y=8 => 18
x=2; y=4 => 24
x=4; y=2 => 42
x=8; y=1 => 81
22 tháng 7 2023
`a, (x-y)^2 = (x+y)^2 - 4xy = 12^2 - 35 . 4 = 144 - 140 = 4`.
`b, (x+y)^2 = (x-y)^2 + 4xy = 8^2 + 20.4 = 64 + 80 = 144`
`c, x^3 + y^3 = (x+y)^3 - 3xy(x+y) = 5^3 - 3 . 6 . 5 = 125 - 90 = 35`
`d, x^3 - y^3 = (x-y)^3 - 3xy(x-y) = 3^3 - 3 .40 . 3 = 27 - 360 = -333`.
CG
1
NM
Nguyễn Minh Quang
Giáo viên
12 tháng 8 2021
a. ta có : \(x^2+y^2=\left(x+y\right)^2-2xy=1^2-2\times\left(-6\right)=13\)
\(x^3+y^3=\left(x+y\right)^3-3xy\left(x+y\right)=1^3-3\times\left(-6\right)\times1=19\)
\(x^5+y^5=\left(x+y\right)\left[x^4-x^3y+x^2y^2-xy^3+y^4\right]\)
\(=\left(x+y\right)\left[\left(x^2+y^2\right)^2-x^2y^2-xy\left(x^2+y^2\right)\right]=1.\left(13^2-\left(-6\right)^2-\left(-6\right).13\right)=211\)
b.\(x^2+y^2=\left(x-y\right)^2+2xy=1+2\times6=13\)
\(x^3-y^3=\left(x-y\right)^3+3xy\left(x-y\right)=1^3+6.3.1=19\)
\(x^5-y^5=\left(x-y\right)\left[\left(x^4+x^3y+x^2y^2+xy^3+y^4\right)\right]\)
\(=\left(x-y\right)\left[\left(x^2+y^2\right)^2-x^2y^2+xy\left(x^2+y^2\right)\right]=1.\left(13^2-6^2+6.13\right)=211\)
24 tháng 11 2021
\(a,=\sqrt{x}\left(\sqrt{y}-\sqrt{x}\right)\\ b,=\left(\sqrt{x}-\sqrt{y}\right)^2\\ c,=\sqrt{xy}\left(\sqrt{x}-\sqrt{y}\right)\\ d,=\sqrt{x}\left(\sqrt{y}+2\right)-3\left(\sqrt{y}+2\right)\\ =\left(\sqrt{x}-3\right)\left(\sqrt{y}+2\right)\)
QD
1
12 tháng 6 2023
đkxđ: \(\left\{{}\begin{matrix}x\ne0\\y\ne0\end{matrix}\right.\)
pt đầu \(\Leftrightarrow x+\dfrac{2}{x}+y+\dfrac{1}{y}=6\) (3)
pt thứ 2 \(\Leftrightarrow x^2+\dfrac{4}{x^2}+y^2+\dfrac{1}{y^2}=14\) \(\Leftrightarrow\left(x^2+2.x.\dfrac{2}{x}+\dfrac{4}{x^2}\right)+\left(y^2+2y.\dfrac{1}{y}+\dfrac{1}{y^2}\right)=20\)
\(\Leftrightarrow\left(x+\dfrac{2}{x}\right)^2+\left(y+\dfrac{1}{y}\right)^2=20\) (4)
Đặt \(\left\{{}\begin{matrix}x+\dfrac{2}{x}=u\left(\left|u\right|\ge2\sqrt{2}\right)\\y+\dfrac{1}{y}=v\left(\left|v\right|\ge2\right)\end{matrix}\right.\) thì từ (3) và (4) suy ra \(\left\{{}\begin{matrix}u+v=6\\u^2+v^2=20\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}v=6-u\\u^2+\left(6-u\right)^2=20\end{matrix}\right.\)
\(u^2+\left(6-u\right)^2=20\) \(\Leftrightarrow u^2+36-12u+u^2=20\) \(\Leftrightarrow2u^2-12u+16=0\) \(\Leftrightarrow u^2-6u+8=0\) \(\Leftrightarrow\left(u-2\right)\left(u-4\right)=0\) \(\Leftrightarrow\left[{}\begin{matrix}u=2\left(loại\right)\\u=4\left(nhận\right)\end{matrix}\right.\).
\(\Rightarrow v=6-u=2\), suy ra \(\left\{{}\begin{matrix}x+\dfrac{2}{x}=4\\y+\dfrac{1}{y}=2\end{matrix}\right.\)\(\Leftrightarrow\left\{{}\begin{matrix}x=2\pm\sqrt{2}\\y=1\end{matrix}\right.\) (nhận).
Vậy hpt đã cho có các nghiệm \(\left(x;y\right)\in\left\{\left(2-\sqrt{2};1\right);\left(2+\sqrt{2};1\right)\right\}\)
24 tháng 9 2022
a: \(=\dfrac{3}{2}\sqrt{6}+\dfrac{2}{3}\sqrt{6}-2\sqrt{3}=\dfrac{13}{6}\sqrt{6}-2\sqrt{3}\)
b: \(VT=\dfrac{\sqrt{xy}\left(\sqrt{x}+\sqrt{y}\right)}{\sqrt{xy}}\cdot\left(\sqrt{x}+\sqrt{y}\right)=\left(\sqrt{x}+\sqrt{y}\right)^2\)
c: \(VT=\dfrac{\sqrt{y}}{\sqrt{x}\left(\sqrt{x}-\sqrt{y}\right)}+\dfrac{\sqrt{x}}{\sqrt{y}\left(\sqrt{y}-\sqrt{x}\right)}\)
\(=\dfrac{y-x}{\sqrt{xy}\left(\sqrt{x}-\sqrt{y}\right)}=\dfrac{-\left(\sqrt{x}+\sqrt{y}\right)}{\sqrt{xy}}\)