Với tan α = - 4 5 v ớ i 3 π 2 < α < 2 π . Giá trị của sinα và cosα là:
A. sin α = - 4 41 , cos α = - 5 41
B. sin α = 4 41 , cos α = 5 41
C. sin α = - 4 41 , cos α = 5 41
D. sin α = 4 41 , cos α = - 5 41
Hãy nhập câu hỏi của bạn vào đây, nếu là tài khoản VIP, bạn sẽ được ưu tiên trả lời.

a: pi/2<a<pi
=>sin a>0
\(sina=\sqrt{1-\left(-\dfrac{1}{\sqrt{3}}\right)^2}=\dfrac{\sqrt{2}}{\sqrt{3}}\)
\(sin\left(a+\dfrac{pi}{6}\right)=sina\cdot cos\left(\dfrac{pi}{6}\right)+sin\left(\dfrac{pi}{6}\right)\cdot cosa\)
\(=\dfrac{\sqrt{3}}{2}\cdot\dfrac{\sqrt{2}}{\sqrt{3}}+\dfrac{1}{2}\cdot-\dfrac{1}{\sqrt{3}}=\dfrac{\sqrt{6}-2}{2\sqrt{3}}\)
b: \(cos\left(a+\dfrac{pi}{6}\right)=cosa\cdot cos\left(\dfrac{pi}{6}\right)-sina\cdot sin\left(\dfrac{pi}{6}\right)\)
\(=\dfrac{-1}{\sqrt{3}}\cdot\dfrac{\sqrt{3}}{2}-\dfrac{\sqrt{2}}{\sqrt{3}}\cdot\dfrac{1}{2}=\dfrac{-\sqrt{3}-\sqrt{2}}{2\sqrt{3}}\)
c: \(sin\left(a-\dfrac{pi}{3}\right)\)
\(=sina\cdot cos\left(\dfrac{pi}{3}\right)-cosa\cdot sin\left(\dfrac{pi}{3}\right)\)
\(=\dfrac{\sqrt{2}}{\sqrt{3}}\cdot\dfrac{1}{2}+\dfrac{1}{\sqrt{3}}\cdot\dfrac{\sqrt{3}}{2}=\dfrac{\sqrt{2}+\sqrt{3}}{2\sqrt{3}}\)
d: \(cos\left(a-\dfrac{pi}{6}\right)\)
\(=cosa\cdot cos\left(\dfrac{pi}{6}\right)+sina\cdot sin\left(\dfrac{pi}{6}\right)\)
\(=\dfrac{-1}{\sqrt{3}}\cdot\dfrac{\sqrt{3}}{2}+\dfrac{\sqrt{2}}{\sqrt{3}}\cdot\dfrac{1}{2}=\dfrac{-\sqrt{3}+\sqrt{2}}{2\sqrt{3}}\)

Chọn D.
Vì 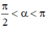 ⇒ sinα > 0, cosα < 0.
⇒ sinα > 0, cosα < 0.
Từ sinα + 2cosα = -1 ⇒ sinα = -1 - 2cosα.
Ta có:
(-1 - 2cosα ) 2 + cos 2 α = 1
⇔ 1 + 4cosα + 4 cos 2 α + cos 2 α = 1
⇔ 5cos2α + 4cosα = 0
⇔ cosα.(5cosα + 4) = 0
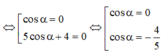
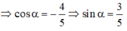
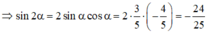

Chọn D.
Ta có:
sin 2 α + cos 2 α = 1
sinα + 2cosα = -1 ⇔ sinα = -1 - 2cosα
⇔ (-1 - 2cosα ) 2 + cos 2 α = 1
⇔ 1 + 4cosα + 4 cos 2 α + cos 2 α = 1
⇔ 5 cos 2 α + 4cosα = 0
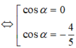
Vì π/2 < α < π ⇒ cosα < 0. Do đó, cos α = -4/5
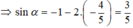
Ta lại có:
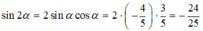

Vì \(\dfrac{\pi}{2}< \alpha< \pi\) \(\Rightarrow\) cos \(\alpha\) < 0
\(\Rightarrow\) cos \(\alpha\) = \(-\sqrt{1-sin^2\alpha}\) = \(-\dfrac{2\sqrt{2}}{3}\)
\(\Rightarrow\) tan \(\alpha\) = \(\dfrac{sin\alpha}{cos\alpha}=\dfrac{-\sqrt{2}}{4}\)
\(\Rightarrow\) cot \(\alpha\) = \(\dfrac{1}{tan\alpha}\) = \(-2\sqrt{2}\)
Chúc bn học tốt!

Chọn D.
Ta có: sin2α = a ⇒ 2sinα.cosα = a với 0 < α < 90o.
sin 2 α + cos 2 α = 1
⇔ sin 2 α + cos 2 α + 2sinαcosα - 2sinαcosα = 1
⇔ (sinα + cosα ) 2 - 2sinαcosα = 1
⇔ (sinα + cosα ) 2 = 1 + 2sinαcosα
⇔ (sinα + cosα ) 2 = 1 + a


Chọn C.
Áp dụng công thức: cos2α = 1 - 2 sin 2 α = 1 - 2.(0,6 ) 2 = 0,28
Chọn C.
yvghtoyhlu4lworiorioriorioritfzgh dfkj gbvkjfdsj
vnfsmvgbjj cdtndgfbjfdhj gfhb gfkj
dgfhjsgs j fjb rtsfb hn
1732
4983268893574945866346785785784685467847858678675